Preorder

This article is about binary relations. For the graph vertex ordering, seedepth-first search.For purchase orders for unreleased products, seepre-order.For other uses, seePreorder (disambiguation).

"Quasiorder" redirects here. For irreflexive transitive relations, seestrict order.

Transitivebinary relations
Symmetric Antisymmetric Connected Well-founded Has joins Has meets Reflexive Irreflexive Asymmetric Total, Semiconnex Anti- reflexive Equivalence relation Y ✗ ✗ ✗ ✗ ✗ Y ✗ ✗ Preorder(Quasiorder) ✗ ✗ ✗ ✗ ✗ ✗ Y ✗ ✗ Partial order ✗ Y ✗ ✗ ✗ ✗ Y ✗ ✗ Total preorder ✗ ✗ Y ✗ ✗ ✗ Y ✗ ✗ Total order ✗ Y Y ✗ ✗ ✗ Y ✗ ✗ Prewellordering ✗ ✗ Y Y ✗ ✗ Y ✗ ✗ Well-quasi-ordering ✗ ✗ ✗ Y ✗ ✗ Y ✗ ✗ Well-ordering ✗ Y Y Y ✗ ✗ Y ✗ ✗ Lattice ✗ Y ✗ ✗ Y Y Y ✗ ✗ Join-semilattice ✗ Y ✗ ✗ Y ✗ Y ✗ ✗ Meet-semilattice ✗ Y ✗ ✗ ✗ Y Y ✗ ✗ Strict partial order ✗ Y ✗ ✗ ✗ ✗ ✗ Y Y Strict weak order ✗ Y ✗ ✗ ✗ ✗ ✗ Y Y Strict total order ✗ Y Y ✗ ✗ ✗ ✗ Y Y Symmetric Antisymmetric Connected Well-founded Has joins Has meets Reflexive Irreflexive Asymmetric Definitions, for all$a,b$and$S\neq \varnothing:$ ${\begin{aligned}&aRb\\\Rightarrow {}&bRa\end{aligned}}$ ${\begin{aligned}aRb{\text{ and }}&bRa\\\Rightarrow a={}&b\end{aligned}}$ ${\begin{aligned}a\neq {}&b\Rightarrow \\aRb{\text{ or }}&bRa\end{aligned}}$ ${\begin{aligned}\min S\\{\text{exists}}\end{aligned}}$ ${\begin{aligned}a\vee b\\{\text{exists}}\end{aligned}}$ ${\begin{aligned}a\wedge b\\{\text{exists}}\end{aligned}}$ $aRa$ ${\text{not }}aRa$ ${\begin{aligned}aRb\Rightarrow \\{\text{not }}bRa\end{aligned}}$
Yindicates that the column's property is always true for the row's term (at the very left), while✗indicates that the property is not guaranteed in general (it might, or might not, hold). For example, that every equivalence relation is symmetric, but not necessarily antisymmetric, is indicated byYin the "Symmetric" column and✗in the "Antisymmetric" column, respectively. All definitions tacitly require thehomogeneous relation$R$betransitive:for all$a,b,c,$if$aRb$and$bRc$then$aRc.$ A term's definition may require additional properties that are not listed in this table.

Inmathematics,especially inorder theory,apreorderorquasiorderis abinary relationthat isreflexiveandtransitive.The namepreorderis meant to suggest that preorders arealmostpartial orders,but not quite, as they are not necessarilyantisymmetric.

Hasse diagramof the preorderx R ydefined byx//4≤y//4 on thenatural numbers.Equivalence classes (sets of elements such thatx R yandy R x) are shown together as a single node. The relation on equivalence classes is apartial order.

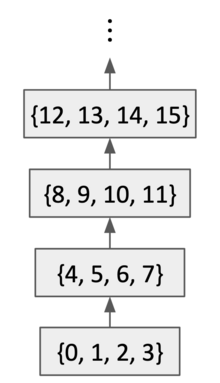
A natural example of a preorder is thedivides relation"x divides y" between integers,polynomials,or elements of acommutative ring.For example, the divides relation is reflexive as every integer divides itself. But the divides relation is not antisymmetric, because$1$divides$-1$and$-1$divides$1$.It is to this preorder that "greatest" and "lowest" refer in the phrases "greatest common divisor"and"lowest common multiple"(except that, for integers, the greatest common divisor is also the greatest for the natural order of the integers).

Preorders are closely related toequivalence relationsand (non-strict) partial orders. Both of these are special cases of a preorder: an antisymmetric preorder is a partial order, and asymmetricpreorder is an equivalence relation. Moreover, a preorder on a set$X$can equivalently be defined as an equivalence relation on$X$,together with a partial order on the set of equivalence class. Like partial orders and equivalence relations, preorders (on a nonempty set) are neverasymmetric.

A preorder can be visualized as adirected graph,with elements of the set corresponding to vertices, and the order relation between pairs of elements corresponding to the directed edges between vertices. The converse is not true: most directed graphs are neither reflexive nor transitive. A preorder that is antisymmetric no longer has cycles; it is a partial order, and corresponds to adirected acyclic graph.A preorder that is symmetric is an equivalence relation; it can be thought of as having lost the direction markers on the edges of the graph. In general, a preorder's corresponding directed graph may have many disconnected components.

As a binary relation, a preorder may be denoted$\,\lesssim \,$or$\,\leq \,$.In words, when$a\lesssim b,$one may say thatbcoversaor thataprecedesb,or thatbreducestoa.Occasionally, the notation ← or → is also used.

Definition

Let$\,\lesssim \,$be a binary relation on aset$P,$so that by definition,$\,\lesssim \,$is some subset of$P\times P$and the notation$a\lesssim b$is used in place of$(a,b)\in \,\lesssim.$Then$\,\lesssim \,$is called apreorderorquasiorderif it isreflexiveandtransitive;that is, if it satisfies:

1. Reflexivity:$a\lesssim a$for all$a\in P,$and
2. Transitivity:if$a\lesssim b{\text{ and }}b\lesssim c{\text{ then }}a\lesssim c$for all$a,b,c\in P.$

A set that is equipped with a preorder is called apreordered set(orproset).

Preorders as partial orders on partitions

Given a preorder$\,\lesssim \,$on$S$one may define anequivalence relation$\,\sim \,$on$S$such that $$a\sim b\quad {\text{ if and only if }}\quad a\lesssim b\;{\text{ and }}\;b\lesssim a.$$ The resulting relation$\,\sim \,$is reflexive since the preorder$\,\lesssim \,$is reflexive; transitive by applying the transitivity of$\,\lesssim \,$twice; and symmetric by definition.

Using this relation, it is possible to construct a partial order on the quotient set of the equivalence,$S/\sim,$which is the set of allequivalence classesof$\,\sim.$If the preorder is denoted by$R^{+=},$then$S/\sim$is the set of$R$-cycleequivalence classes: $x\in [y]$if and only if$x=y$or$x$is in an$R$-cycle with$y$. In any case, on$S/\sim$it is possible to define$[x]\leq [y]$if and only if$x\lesssim y.$ That this is well-defined, meaning that its defining condition does not depend on which representatives of$[x]$and$[y]$are chosen, follows from the definition of$\,\sim.\,$It is readily verified that this yields a partially ordered set.

Conversely, from any partial order on a partition of a set$S,$it is possible to construct a preorder on$S$itself. There is aone-to-one correspondencebetween preorders and pairs (partition, partial order).

Example:Let$S$be aformal theory,which is a set ofsentenceswith certain properties (details of which can be found inthe article on the subject). For instance,$S$could be afirst-order theory(likeZermelo–Fraenkel set theory) or a simplerzeroth-order theory.One of the many properties of$S$is that it is closed under logical consequences so that, for instance, if a sentence$A\in S$logically implies some sentence$B,$which will be written as$A\Rightarrow B$and also as$B\Leftarrow A,$then necessarily$B\in S$(bymodus ponens). The relation$\,\Leftarrow \,$is a preorder on$S$because$A\Leftarrow A$always holds and whenever$A\Leftarrow B$and$B\Leftarrow C$both hold then so does$A\Leftarrow C.$ Furthermore, for any$A,B\in S,$$A\sim B$if and only if$A\Leftarrow B{\text{ and }}B\Leftarrow A$;that is, two sentences are equivalent with respect to$\,\Leftarrow \,$if and only if they arelogically equivalent.This particular equivalence relation$A\sim B$is commonly denoted with its own special symbol$A\iff B,$and so this symbol$\,\iff \,$may be used instead of$\,\sim.$The equivalence class of a sentence$A,$denoted by$[A],$consists of all sentences$B\in S$that are logically equivalent to$A$(that is, all$B\in S$such that$A\iff B$). The partial order on$S/\sim$induced by$\,\Leftarrow,\,$which will also be denoted by the same symbol$\,\Leftarrow,\,$is characterized by$[A]\Leftarrow [B]$if and only if$A\Leftarrow B,$where the right hand side condition is independent of the choice of representatives$A\in [A]$and$B\in [B]$of the equivalence classes. All that has been said of$\,\Leftarrow \,$so far can also be said of itsconverse relation$\,\Rightarrow.\,$ The preordered set$(S,\Leftarrow )$is adirected setbecause if$A,B\in S$and if$C:=A\wedge B$denotes the sentence formed bylogical conjunction$\,\wedge,\,$then$A\Leftarrow C$and$B\Leftarrow C$where$C\in S.$The partially ordered set$\left(S/\sim,\Leftarrow \right)$is consequently also a directed set. SeeLindenbaum–Tarski algebrafor a related example.

Relationship to strict partial orders

If reflexivity is replaced withirreflexivity(while keeping transitivity) then we get the definition of astrict partial orderon$P$.For this reason, the termstrict preorderis sometimes used for a strict partial order. That is, this is a binary relation$\,<\,$on$P$that satisfies:

1. Irreflexivityor anti-reflexivity:not$a<a$for all$a\in P;$that is,$\,a<a$isfalsefor all$a\in P,$and
2. Transitivity:if$a<b{\text{ and }}b<c{\text{ then }}a<c$for all$a,b,c\in P.$

Strict partial order induced by a preorder

Any preorder$\,\lesssim \,$gives rise to a strict partial order defined by$a<b$if and only if$a\lesssim b$and not$b\lesssim a$. Using the equivalence relation$\,\sim \,$introduced above,$a<b$if and only if$a\lesssim b{\text{ and not }}a\sim b;$ and so the following holds $$a\lesssim b\quad {\text{ if and only if }}\quad a<b\;{\text{ or }}\;a\sim b.$$ The relation$\,<\,$is astrict partial orderandeverystrict partial order can be constructed this way. Ifthe preorder$\,\lesssim \,$isantisymmetric(and thus a partial order) then the equivalence$\,\sim \,$is equality (that is,$a\sim b$if and only if$a=b$) and so in this case, the definition of$\,<\,$can be restated as: $$a<b\quad {\text{ if and only if }}\quad a\lesssim b\;{\text{ and }}\;a\neq b\quad \quad ({\text{assuming }}\lesssim {\text{ is antisymmetric}}).$$ But importantly, this new condition isnotused as (nor is it equivalent to) the general definition of the relation$\,<\,$(that is,$\,<\,$isnotdefined as:$a<b$if and only if$a\lesssim b{\text{ and }}a\neq b$) because if the preorder$\,\lesssim \,$is not antisymmetric then the resulting relation$\,<\,$would not be transitive (consider how equivalent non-equal elements relate). This is the reason for using the symbol "$\lesssim$"instead of the" less than or equal to "symbol"$\leq$",which might cause confusion for a preorder that is not antisymmetric since it might misleadingly suggest that$a\leq b$implies$a<b{\text{ or }}a=b.$

Preorders induced by a strict partial order

Using the construction above, multiple non-strict preorders can produce the same strict preorder$\,<,\,$so without more information about how$\,<\,$was constructed (such knowledge of the equivalence relation$\,\sim \,$for instance), it might not be possible to reconstruct the original non-strict preorder from$\,<.\,$Possible (non-strict) preorders that induce the given strict preorder$\,<\,$include the following:

• Define$a\leq b$as$a<b{\text{ or }}a=b$(that is, take the reflexive closure of the relation). This gives the partial order associated with the strict partial order "$<$"through reflexive closure; in this case the equivalence is equality$\,=,$so the symbols$\,\lesssim \,$and$\,\sim \,$are not needed.
• Define$a\lesssim b$as "${\text{ not }}b<a$"(that is, take the inverse complement of the relation), which corresponds to defining$a\sim b$as "neither$a<b{\text{ nor }}b<a$";these relations$\,\lesssim \,$and$\,\sim \,$are in general not transitive; however, if they are then$\,\sim \,$is an equivalence; in that case "$<$"is astrict weak order.The resulting preorder isconnected(formerly called total); that is, atotal preorder.

If$a\leq b$then$a\lesssim b.$ The converse holds (that is,$\,\lesssim \;\;=\;\;\leq \,$) if and only if whenever$a\neq b$then$a<b$or$b<a.$

Examples

Graph theory

• Thereachabilityrelationship in anydirected graph(possibly containing cycles) gives rise to a preorder, where$x\lesssim y$in the preorder if and only if there is a path fromxtoyin the directed graph. Conversely, every preorder is the reachability relationship of a directed graph (for instance, the graph that has an edge fromxtoyfor every pair(x,y)with$x\lesssim y$). However, many different graphs may have the same reachability preorder as each other. In the same way, reachability ofdirected acyclic graphs,directed graphs with no cycles, gives rise topartially ordered sets(preorders satisfying an additional antisymmetry property).
• Thegraph-minorrelation is also a preorder.

Computer science

In computer science, one can find examples of the following preorders.

• Asymptotic ordercauses a preorder over functions$f:\mathbb {N} \to \mathbb {N}$.The corresponding equivalence relation is calledasymptotic equivalence.
• Polynomial-time,many-one (mapping)andTuring reductionsare preorders on complexity classes.
• Subtypingrelations are usually preorders.
• Simulation preordersare preorders (hence the name).
• Reduction relationsinabstract rewriting systems.
• Theencompassment preorderon the set ofterms,defined by$s\lesssim t$if asubtermoftis asubstitution instanceofs.
• Theta-subsumption,which is when the literals in a disjunctive first-order formula are contained by another, after applying asubstitutionto the former.

Category theory

• Acategorywith at most onemorphismfrom any objectxto any other objectyis a preorder. Such categories are calledthin.Here theobjectscorrespond to the elements of$P,$and there is one morphism for objects which are related, zero otherwise. In this sense, categories "generalize" preorders by allowing more than one relation between objects: each morphism is a distinct (named) preorder relation.
• Alternately, a preordered set can be understood as anenriched category,enriched over the category$2=(0\to 1).$

Other

Further examples:

• Everyfinite topological spacegives rise to a preorder on its points by defining$x\lesssim y$if and only ifxbelongs to everyneighborhoodofy.Every finite preorder can be formed as thespecialization preorderof a topological space in this way. That is, there is aone-to-one correspondencebetween finite topologies and finite preorders. However, the relation between infinite topological spaces and their specialization preorders is not one-to-one.

• Anetis adirectedpreorder, that is, each pair of elements has anupper bound.The definition of convergence via nets is important intopology,where preorders cannot be replaced bypartially ordered setswithout losing important features.

• The relation defined by$x\lesssim y$if$f(x)\lesssim f(y),$wherefis a function into some preorder.
• The relation defined by$x\lesssim y$if there exists someinjectionfromxtoy.Injection may be replaced bysurjection,or any type of structure-preserving function, such asring homomorphism,orpermutation.
• Theembeddingrelation for countabletotal orderings.

Example of atotal preorder:

• Preference,according to common models.

Constructions

Every binary relation$R$on a set$S$can be extended to a preorder on$S$by taking thetransitive closureandreflexive closure,$R^{+=}.$The transitive closure indicates path connection in$R:xR^{+}y$if and only if there is an$R$-pathfrom$x$to$y.$

Left residual preorder induced by a binary relation

Given a binary relation$R,$the complemented composition$R\backslash R={\overline {R^{\textsf {T}}\circ {\overline {R}}}}$forms a preorder called theleft residual,where$R^{\textsf {T}}$denotes theconverse relationof$R,$and${\overline {R}}$denotes thecomplementrelation of$R,$while$\circ$denotesrelation composition.

Related definitions

If a preorder is alsoantisymmetric,that is,$a\lesssim b$and$b\lesssim a$implies$a=b,$then it is apartial order.

On the other hand, if it issymmetric,that is, if$a\lesssim b$implies$b\lesssim a,$then it is anequivalence relation.

A preorder istotalif$a\lesssim b$or$b\lesssim a$for all$a,b\in P.$

Apreordered classis aclassequipped with a preorder. Every set is a class and so every preordered set is a preordered class.

Uses

Preorders play a pivotal role in several situations:

• Every preorder can be given a topology, theAlexandrov topology;and indeed, every preorder on a set is in one-to-one correspondence with an Alexandrov topology on that set.
• Preorders may be used to defineinterior algebras.
• Preorders provide theKripke semanticsfor certain types ofmodal logic.
• Preorders are used inforcinginset theoryto proveconsistencyandindependenceresults.

Number of preorders

Number ofn-element binary relations of different types

Elem­ents	Any	Transitive	Reflexive	Symmetric	Preorder	Partial order	Total preorder	Total order	Equivalence relation
0	1	1	1	1	1	1	1	1	1
1	2	2	1	2	1	1	1	1	1
2	16	13	4	8	4	3	3	2	2
3	512	171	64	64	29	19	13	6	5
4	65,536	3,994	4,096	1,024	355	219	75	24	15
n	2n2		2n(n−1)	2n(n+1)/2			∑n k=0k!S(n,k)	n!	∑n k=0S(n,k)
OEIS	A002416	A006905	A053763	A006125	A000798	A001035	A000670	A000142	A000110

Note thatS(n,k)refers toStirling numbers of the second kind.

As explained above, there is a 1-to-1 correspondence between preorders and pairs (partition, partial order). Thus the number of preorders is the sum of the number of partial orders on every partition. For example:

• for$n=3:$
  • 1 partition of 3, giving 1 preorder
  • 3 partitions of2 + 1,giving$3\times 3=9$preorders
  • 1 partition of1 + 1 + 1,giving 19 preorders
  I.e., together, 29 preorders.
• for$n=4:$
  • 1 partition of 4, giving 1 preorder
  • 7 partitions with two classes (4 of3 + 1and 3 of2 + 2), giving$7\times 3=21$preorders
  • 6 partitions of2 + 1 + 1,giving$6\times 19=114$preorders
  • 1 partition of1 + 1 + 1 + 1,giving 219 preorders
  I.e., together, 355 preorders.

Interval

For$a\lesssim b,$theinterval$[a,b]$is the set of pointsxsatisfying$a\lesssim x$and$x\lesssim b,$also written$a\lesssim x\lesssim b.$It contains at least the pointsaandb.One may choose to extend the definition to all pairs$(a,b)$The extra intervals are all empty.

Using the corresponding strict relation "$<$",one can also define the interval$(a,b)$as the set of pointsxsatisfying$a<x$and$x<b,$also written$a<x<b.$An open interval may be empty even if$a<b.$

Also$[a,b)$and$(a,b]$can be defined similarly.

See also

• Partial order– preorder that isantisymmetric
• Equivalence relation– preorder that issymmetric
• Total preorder– preorder that istotal
• Total order– preorder that is antisymmetric and total
• Directed set
• Category of preordered sets
• Prewellordering
• Well-quasi-ordering

References

• Schmidt, Gunther, "Relational Mathematics", Encyclopedia of Mathematics and its Applications, vol. 132, Cambridge University Press, 2011,ISBN978-0-521-76268-7
• Schröder, Bernd S. W. (2002),Ordered Sets: An Introduction,Boston: Birkhäuser,ISBN0-8176-4128-9