Contraposition

Inlogicandmathematics,contraposition,ortransposition,refers to theinferenceof going from aconditional statementinto itslogically equivalentcontrapositive,and an associated proof method known as§ Proof by contrapositive.The contrapositive of a statement has itsantecedentandconsequentinvertedandflipped.

Conditional statement$P\rightarrow Q$.Informulas:the contrapositive of$P\rightarrow Q$is$\neg Q\rightarrow \neg P$.

IfP,ThenQ.— If notQ,Then notP."Ifit is raining,thenI wear my coat "—"IfI don't wear my coat,thenit isn't raining. "

The law of contraposition says that a conditional statement is true if, and only if, its contrapositive is true.

The contrapositive ($\neg Q\rightarrow \neg P$) can be compared with three other statements:

Inversion(theinverse),$\neg P\rightarrow \neg Q$

"Ifit is not raining,thenI don't wear my coat."Unlike the contrapositive, the inverse'struth valueis not at all dependent on whether or not the original proposition was true, as evidenced here.

Conversion(theconverse),$Q\rightarrow P$

"IfI wear my coat,thenit is raining."The converse is actually the contrapositive of the inverse, and so always has the same truth value as the inverse (which as stated earlier does not always share the same truth value as that of the original proposition).

Negation(thelogical complement),$\neg (P\rightarrow Q)$

"It is not the case thatifit is rainingthenI wear my coat.",or equivalently,"Sometimes, when it is raining, I don't wear my coat."If the negation is true, then the original proposition (and by extension the contrapositive) is false.

Note that if$P\rightarrow Q$is true and one is given that$Q$is false (i.e.,$\neg Q$), then it can logically be concluded that$P$must be also false (i.e.,$\neg P$). This is often called thelaw of contrapositive,or themodus tollensrule of inference.

Intuitive explanation

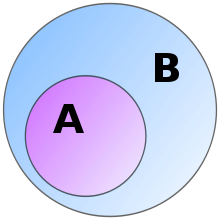
In theEuler diagramshown, if something is in A, it must be in B as well. So we can interpret "all of A is in B" as:

$A\to B$

It is also clear that anything that isnotwithin B (the blue region)cannotbe within A, either. This statement, which can be expressed as:

$\neg B\to \neg A$

is the contrapositive of the above statement. Therefore, one can say that

$(A\to B)\leftrightarrow (\neg B\to \neg A).$

In practice, this equivalence can be used to make proving a statement easier. For example, if one wishes to prove that every girl in the United States (A) has brown hair (B), one can either try to directly prove$A\to B$by checking that all girls in the United States do indeed have brown hair, or try to prove$\neg B\to \neg A$by checking that all girls without brown hair are indeed all outside the US. In particular, if one were to find at least one girl without brown hair within the US, then one would have disproved$\neg B\to \neg A$,and equivalently$A\to B$.

In general, for any statement whereAimpliesB,not Balways impliesnot A.As a result, proving or disproving either one of these statements automatically proves or disproves the other, as they are logically equivalent to each other.

Formal definition

A propositionQis implicated by a propositionPwhen the following relationship holds:

$(P\to Q)$

This states that, "if$P$,then$Q$",or," ifSocrates is a man,thenSocrates is human."In a conditional such as this,$P$is theantecedent,and$Q$is theconsequent.One statement is thecontrapositiveof the other only when its antecedent is thenegatedconsequent of the other, and vice versa. Thus a contrapositive generally takes the form of:

$(\neg Q\to \neg P).$

That is, "If not-$Q$,then not-$P$",or, more clearly," If$Q$is not the case, thenPis not the case. "Using our example, this is rendered as" IfSocrates is not human,thenSocrates is not a man."This statement is said to becontraposedto the original and is logically equivalent to it. Due to theirlogical equivalence,stating one effectively states the other; when one istrue,the other is also true, and when one is false, the other is also false.

Strictly speaking, a contraposition can only exist in two simple conditionals. However, a contraposition may also exist in two complex, universal conditionals, if they are similar. Thus,$\forall {x}(P{x}\to Q{x})$,or "All$P$s are$Q$s, "is contraposed to$\forall {x}(\neg Q{x}\to \neg P{x})$,or "All non-$Q$s are non-$P$s. "

Sequent notation

Thetranspositionrule may be expressed as asequent:

$(P\to Q)\vdash (\neg Q\to \neg P),$

where$\vdash$is a metalogical symbol meaning that$(\neg Q\to \neg P)$is asyntactic consequenceof$(P\to Q)$in some logical system; or as a rule of inference:

${\frac {P\to Q}{\therefore \neg Q\to \neg P}},$

where the rule is that wherever an instance of "$P\to Q$"appears on a line of a proof, it can be replaced with"$\neg Q\to \neg P$";or as the statement of a truth-functionaltautologyortheoremof propositional logic. The principle was stated as a theorem of propositional logic byRussellandWhiteheadinPrincipia Mathematicaas

$(P\to Q)\to (\neg Q\to \neg P),$

where$P$and$Q$are propositions expressed in someformal system.

Proofs

Simple proof by definition of a conditional

Infirst-order logic,the conditional is defined as:

$A\to B\,\leftrightarrow \,\neg A\lor B$

which can be made equivalent to its contrapositive, as follows:

${\begin{aligned}\neg A\lor B\,&\,\leftrightarrow B\lor \neg A\\\,&\,\leftrightarrow \neg B\to \neg A\end{aligned}}$

Simple proof by contradiction

Let:

$(A\to B)\land \neg B$

It is given that, if A is true, then B is true, and it is also given that B is not true. We can then show that A must not be true by contradiction. For if A were true, then B would have to also be true (byModus Ponens). However, it is given that B is not true, so we have a contradiction. Therefore, A is not true (assuming that we are dealing withbivalent statementsthat are either true or false):

$(A\to B)\to (\neg B\to \neg A)$

We can apply the same process the other way round, starting with the assumptions that:

$(\neg B\to \neg A)\land A$

Here, we also know that B is either true or not true. If B is not true, then A is also not true. However, it is given that A is true, so the assumption that B is not true leads to a contradiction, which means that it is not the case that B is not true. Therefore, B must be true:

$(\neg B\to \neg A)\to (A\to B)$

Combining the two proved statements together, we obtain the sought-after logical equivalence between a conditional and its contrapositive:

$(A\to B)\equiv (\neg B\to \neg A)$

More rigorous proof of the equivalence of contrapositives

Logical equivalence between two propositions means that they are true together or false together. To prove that contrapositives arelogically equivalent,we need to understand when material implication is true or false.

$P\to Q$

This is only false when$P$is true and$Q$is false. Therefore, we can reduce this proposition to the statement "False when$P$and not-$Q$"(i.e." True when it is not the case that$P$and not-$Q$"):

$\neg (P\land \neg Q)$

The elements of aconjunctioncan be reversed with no effect (bycommutativity):

$\neg (\neg Q\land P)$

We define$R$as equal to "$\neg Q$",and$S$as equal to$\neg P$(from this,$\neg S$is equal to$\neg \neg P$,which is equal to just$P$):

$\neg (R\land \neg S)$

This reads "It is not the case that (Ris true andSis false) ", which is the definition of a material conditional. We can then make this substitution:

$R\to S$

By revertingRandSback into$P$and$Q$,we then obtain the desired contrapositive:

$\neg Q\to \neg P$

In classical propositional calculus system

InHilbert-style deductive systemsfor propositional logic, only one side of the transposition is taken as an axiom, and the other is a theorem. We describe a proof of this theorem in the system of three axioms proposed byJan Łukasiewicz:

A1.$\phi \to \left(\psi \to \phi \right)$

A2.$\left(\phi \to \left(\psi \rightarrow \xi \right)\right)\to \left(\left(\phi \to \psi \right)\to \left(\phi \to \xi \right)\right)$

A3.$\left(\lnot \phi \to \lnot \psi \right)\to \left(\psi \to \phi \right)$

(A3) already gives one of the directions of the transposition. The other side,$(\psi \to \phi )\to (\neg \phi \to \neg \psi )$,is proven below, using the following lemmas provenhere:

(DN1)$\neg \neg p\to p$-Double negation(one direction)

(DN2)$p\to \neg \neg p$- Double negation (another direction)

(HS1)$(q\to r)\to ((p\to q)\to (p\to r))$- one form ofHypothetical syllogism

(HS2)$(p\to q)\to ((q\to r)\to (p\to r))$- another form of Hypothetical syllogism.

We also use the method of thehypothetical syllogism metatheoremas a shorthand for several proof steps.

The proof is as follows:

1. $q\to \neg \neg q$(instance of the (DN2))
2. $(q\to \neg \neg q)\to ((p\to q)\to (p\to \neg \neg q))$(instance of the (HS1)
3. $(p\to q)\to (p\to \neg \neg q)$(from (1) and (2) by modus ponens)
4. $\neg \neg p\to p$(instance of the (DN1))
5. $(\neg \neg p\to p)\to ((p\to \neg \neg q)\to (\neg \neg p\to \neg \neg q))$(instance of the (HS2))
6. $(p\to \neg \neg q)\to (\neg \neg p\to \neg \neg q)$(from (4) and (5) by modus ponens)
7. $(p\to q)\to (\neg \neg p\to \neg \neg q)$(from (3) and (6) using the hypothetical syllogism metatheorem)
8. $(\neg \neg p\to \neg \neg q)\to (\neg q\to \neg p)$(instance of (A3))
9. $(p\to q)\to (\neg q\to \neg p)$(from (7) and (8) using the hypothetical syllogism metatheorem)

Comparisons

name	form	description
implication	ifPthenQ	first statement implies truth of second
inverse	if notPthen notQ	negation of both statements
converse	ifQthenP	reversal of both statements
contrapositive	if notQthen notP	reversal and negation of both statements
negation	Pand notQ	contradicts the implication

Examples

Take the statement "All red objects have color."This can be equivalently expressed as"If an object is red, then it has color."

• Thecontrapositiveis "If an object does not have color, then it is not red."This follows logically from our initial statement and, like it, it is evidently true.
• Theinverseis "If an object is not red, then it does not have color."An object which is blue is not red, and still has color. Therefore, in this case the inverse is false.
• Theconverseis "If an object has color, then it is red."Objects can have other colors, so the converse of our statement is false.
• Thenegationis "There exists a red object that does not have color."This statement is false because the initial statement which it negates is true.

In other words, the contrapositive is logically equivalent to a givenconditionalstatement, though not sufficient for abiconditional.

Similarly, take the statement "Allquadrilateralshave four sides,"or equivalently expressed"If a polygon is a quadrilateral, then it has four sides."

• Thecontrapositiveis "If a polygon does not have four sides, then it is not a quadrilateral."This follows logically, and as a rule, contrapositives share thetruth valueof their conditional.
• Theinverseis "If a polygon is not a quadrilateral, then it does not have four sides."In this case, unlike the last example, the inverse of the statement is true.
• Theconverseis "If a polygon has four sides, then it is a quadrilateral."Again, in this case, unlike the last example, the converse of the statement is true.
• Thenegationis "There is at least one quadrilateral that does not have four sides."This statement is clearly false.

Since the statement and the converse are both true, it is called abiconditional,and can be expressed as "A polygon is a quadrilateralif, and only if,it has four sides."(The phraseif and only ifis sometimes abbreviated asiff.) That is, having four sides is both necessary to be a quadrilateral, and alone sufficient to deem it a quadrilateral.

Truth

• If a statement is true, then its contrapositive is true (and vice versa).
• If a statement is false, then its contrapositive is false (and vice versa).
• If a statement's inverse is true, then its converse is true (and vice versa).
• If a statement's inverse is false, then its converse is false (and vice versa).
• If a statement's negation is false, then the statement is true (and vice versa).
• If a statement (or its contrapositive) and the inverse (or the converse) are both true or both false, then it is known as alogical biconditional.

Traditional logic

See also:Categorical proposition § Contraposition

Intraditional logic,contrapositionis a form ofimmediate inferencein which apropositionis inferred from another and where the former has for itssubjectthecontradictoryof the original logical proposition'spredicate.In some cases, contraposition involves a change of the former's quality (i.e. affirmation or negation).For its symbolic expression in modern logic, see therule of transposition.Contraposition also has philosophical application distinct from the other traditionalinferenceprocesses ofconversionandobversionwhere equivocation varies with different proposition types.

Intraditional logic,the process of contraposition is a schema composed of several steps of inference involvingcategorical propositionsandclasses.A categorical proposition contains asubjectandpredicatewhere the existential impact of thecopulaimplies the proposition as referring to a classwith at least one member,in contrast to the conditional form ofhypotheticalormaterially implicativepropositions, which are compounds of other propositions, e.g. "If P, then Q" (P and Q are both propositions), and their existential impact is dependent upon further propositions where quantification existence is instantiated (existential instantiation), not on the hypothetical or materially implicative propositions themselves.

Full contrapositionis the simultaneous interchange andnegationof the subject and predicate, and is valid only for the type "A" and type "O" propositions ofAristotelian logic,while it is conditionally valid for "E" type propositions if a change in quantity fromuniversaltoparticularis made (partial contraposition). Since the validobverseis obtained for all the four types (A, E, I, and O types) of traditional propositions, yielding propositions with the contradictory of the original predicate, (full) contraposition is obtained by converting the obvert of the original proposition. For "E" statements, partial contraposition can be obtained by additionally making a change in quantity. Becausenothing is said in the definition of contraposition with regard to the predicate of the inferred proposition,it can be either the original subject, or its contradictory, resulting in two contrapositives which are the obverts of one another in the "A", "O", and "E" type propositions.

By example: from an original, 'A' type categorical proposition,

All residents are voters,

which presupposes that all classes have members and the existential import presumed in the form of categorical propositions, one can derive first byobversionthe 'E' type proposition,

No residents are non-voters.

The contrapositive of the original proposition is then derived byconversionto another 'E' type proposition,

No non-voters are residents.

The process is completed by further obversion resulting in the 'A' type proposition that is the obverted contrapositive of the original proposition,

All non-voters are non-residents.

The schema of contraposition:

Original proposition	Obversion		(Full) Contraposition	Obverted (full) contraposition
(A) All S is P	(E) No S is non-P	↔	(E) No non-P is S	(A) All non-P is non-S
(E) No S is P	(A) All S is non-P		None	None
(I) Some S is P	(O) Some S is not non-P		None	None
(O) Some S is not P	(I) Some S is non-P	↔	(I) Some non-P is S	(O) Some non-P is not non-S

Notice that contraposition is a valid form of immediate inference only when applied to "A" and "O" propositions. It is not valid for "I" propositions, where the obverse is an "O" proposition which has no validconverse.The contraposition of the "E" proposition is valid only with limitations (per accidens). This is because the obverse of the "E" proposition is an "A" proposition which cannot be validly converted except by limitation, that is, contraposition plus a change in the quantity of the proposition fromuniversaltoparticular.

Also, notice that contraposition is a method of inference which may require the use of other rules of inference. The contrapositive is the product of the method of contraposition, with different outcomes depending upon whether the contraposition is full, or partial. The successive applications of conversion and obversion within the process of contraposition may be given by a variety of names.

The process of thelogical equivalenceof a statement and its contrapositive as defined in traditional class logic isnotone of the axioms ofpropositional logic.In traditional logic there is more than one contrapositive inferred from each original statement. In regard to the "A" proposition this is circumvented in the symbolism of modern logic by the rule oftransposition,or the law of contraposition. In its technical usage within the field of philosophic logic, the term "contraposition" may be limited by logicians (e.g.Irving Copi,Susan Stebbing) to traditional logic and categorical propositions. In this sense the use of the term "contraposition" is usually referred to by "transposition" when applied to hypothetical propositions or material implications.

Form of transposition

In the inferred proposition, the consequent is the contradictory of the antecedent in the original proposition, and the antecedent of the inferred proposition is the contradictory of the consequent of the original proposition. The symbol for material implication signifies the proposition as a hypothetical, or the "if–then" form, e.g. "ifP,thenQ".

The biconditional statement of the rule of transposition (↔) refers to the relation between hypothetical (→)propositions,with each proposition including an antecedent and consequential term. As a matter of logical inference, to transpose or convert the terms of one proposition requires the conversion of the terms of the propositions on both sides of the biconditional relationship, meaning that transposing or converting(P→Q)to(Q→P)requires that the other proposition,(¬Q→ ¬P),to be transposed or converted to(¬P→ ¬Q).Otherwise, converting the terms of one proposition and not the other renders the rule invalid, violating thesufficient conditionandnecessary conditionof the terms of the propositions, where the violation is that the changed proposition commits the fallacy ofdenying the antecedentoraffirming the consequentby means of illicitconversion.

The truth of the rule of transposition is dependent upon the relations of sufficient condition and necessary condition in logic.

Sufficient condition

In the proposition "IfP,thenQ",the occurrence ofPis sufficient reason for the occurrence ofQ.P,as an individual or a class, materially implicatesQ,but the relation ofQtoPis such that the converse proposition "IfQ,thenP"does not necessarily have sufficient condition. The rule of inference for sufficient condition ismodus ponens,which is an argument for conditional implication:

1. Premise (1): IfP,thenQ
2. Premise (2):P
3. Conclusion: Therefore,Q

Necessary condition

Since the converse of premise (1) is not valid, all that can be stated of the relationship ofPandQis that in the absence ofQ,Pdoes not occur, meaning thatQis the necessary condition forP.The rule of inference for necessary condition ismodus tollens:

1. Premise (1): IfP,thenQ
2. Premise (2): notQ
3. Conclusion: Therefore, notP

Necessity and sufficiency example

An example traditionally used by logicians contrasting sufficient and necessary conditions is the statement "If there is fire, then oxygen is present". An oxygenated environment is necessary for fire or combustion, but simply because there is an oxygenated environment does not necessarily mean that fire or combustion is occurring. While one can infer that fire stipulates the presence of oxygen, from the presence of oxygen the converse "If there is oxygen present, then fire is present" cannot be inferred. All that can be inferred from the original proposition is that "If oxygen is not present, then there cannot be fire".

Relationship of propositions

The symbol for the biconditional ( "↔" ) signifies the relationship between the propositions is both necessary and sufficient, and is verbalized as "if and only if",or, according to the example" IfP,thenQ'if and only if' if notQ,then notP".

Necessary and sufficient conditions can be explained by analogy in terms of the concepts and the rules of immediate inference of traditional logic. In the categorical proposition "AllSisP",the subject termSis said to be distributed, that is, all members of its class are exhausted in its expression. Conversely, the predicate termPcannot be said to be distributed, or exhausted in its expression because it is indeterminate whether every instance of a member ofPas a class is also a member ofSas a class. All that can be validly inferred is that "SomePareS".Thus, thetype "A" proposition"AllPisS"cannot be inferred by conversion from the original type" A "proposition" AllSisP".All that can be inferred is the type" A "proposition" All non-Pis non-S"(note that (P→Q) and (¬Q→ ¬P) are both type "A" propositions). Grammatically, one cannot infer "all mortals are men" from "All men are mortal". An type "A" proposition can only be immediately inferred by conversion when both the subject and predicate are distributed, as in the inference "All bachelors are unmarried men" from "All unmarried men are bachelors".

Distinguished from transposition

See also:Transposition (mathematics)andSet theory

While most authors use the terms for the same thing, some authors distinguish transposition from contraposition. Intraditional logicthe reasoning process of transposition as a rule of inference is applied tocategorical propositionsthroughcontrapositionandobversion,a series of immediate inferences where the rule of obversion is first applied to the original categorical proposition "AllSisP";yielding the obverse" NoSis non-P".In the obversion of the original proposition to a type" E "proposition, both terms become distributed. The obverse is then converted, resulting in" No non-PisS",maintaining distribution of both terms. The" No non-PisS"is again obverted, resulting in the [contrapositive]" All non-Pis non-S".Since nothing is said in the definition of contraposition with regard to the predicate of the inferred proposition, it is permissible that it could be the original subject or its contradictory, and the predicate term of the resulting type" A "proposition is again undistributed. This results in two contrapositives, one where the predicate term is distributed, and another where the predicate term is undistributed.

Contraposition is a type ofimmediate inferencein which from a given categorical proposition another categorical proposition is inferred which has as its subject the contradictory of the original predicate. Since nothing is said in the definition of contraposition with regard to the predicate of the inferred proposition, it is permissible that it could be the original subject or its contradictory. This is in contradistinction to the form of the propositions of transposition, which may be material implication, or a hypothetical statement. The difference is that in its application to categorical propositions the result of contraposition is two contrapositives, each being the obvert of the other,i.e. "No non-PisS"and" All non-Pis non-S".The distinction between the two contrapositives is absorbed and eliminated in the principle of transposition, which presupposes the" mediate inferences "of contraposition and is also referred to as the "law of contraposition".

Proof by contrapositive

Because thecontrapositiveof a statement always has the same truth value (truth or falsity) as the statement itself, it can be a powerful tool for proving mathematicaltheorems(especially if the truth of the contrapositive is easier to establish than the truth of the statement itself). Aproof by contrapositiveis adirect proofof the contrapositive of a statement.However, indirect methods such asproof by contradictioncan also be used with contraposition, as, for example, in the proof of the irrationality of thesquare root of 2.By the definition of arational number,the statement can be made that "If${\sqrt {2}}$is rational, then it can be expressed as anirreducible fraction".This statement istruebecause it is a restatement of a definition. The contrapositive of this statement is "If${\sqrt {2}}$cannot be expressed as an irreducible fraction, then it is not rational".This contrapositive, like the original statement, is also true. Therefore, if it can be proven that${\sqrt {2}}$cannot be expressed as an irreducible fraction, then it must be the case that${\sqrt {2}}$is not a rational number. The latter can be proved by contradiction.

The previous example employed the contrapositive of a definition to prove a theorem. One can also prove a theorem by proving the contrapositive of the theorem's statement. To prove thatif a positive integerNis anon-square number,its square root is irrational,we can equivalently prove its contrapositive, thatif a positive integerNhas a square root that is rational, thenNis a square number.This can be shown by setting√Nequal to the rational expressiona/bwithaandbbeing positive integers with no common prime factor, and squaring to obtainN=a²/b²and noting that sinceNis a positive integerb=1 so thatN=a²,a square number.

Inmathematics,proof by contrapositive,or proof by contraposition, is arule of inferenceused inproofs,where one infers a conditional statement from its contrapositive.In other words, the conclusion "ifA,thenB"is inferred by constructing a proof of the claim" if notB,then notA"instead. More often than not, this approach is preferred if the contrapositive is easier to prove than the original conditional statement itself.

Logically, the validity of proof by contrapositive can be demonstrated by the use of the followingtruth table,where it is shown thatp→qand$\lnot$q→$\lnot$pshare the same truth values in all scenarios:

p	q	$\lnot$p	$\lnot$q	p→q	$\lnot$q→$\lnot$p
T	T	F	F	T	T
T	F	F	T	F	F
F	T	T	F	T	T
F	F	T	T	T	T

Difference with proof by contradiction

Proof by contradiction:Assume (for contradiction) that$\neg A$is true. Use this assumption to prove acontradiction.It follows that$\neg A$is false, so$A$is true.

Proof by contrapositive:To prove$A\to B$,prove its contrapositive statement, which is$\neg B\to \neg A$.

Example

Let$x$be an integer.

To prove:If$x^{2}$is even, then$x$is even.

Although adirect proofcan be given, we choose to prove this statement by contraposition. The contrapositive of the above statement is:

If$x$is not even, then$x^{2}$is not even.

This latter statement can be proven as follows: suppose thatxis not even, thenxis odd. The product of two odd numbers is odd, hence$x^{2}=x\cdot x$is odd. Thus$x^{2}$is not even.

Having proved the contrapositive, we can then infer that the original statement is true.

In nonclassical logics

Intuitionistic logic

Inintuitionistic logic,the statement$P\to Q$cannot be proven to be equivalent to$\lnot Q\to \lnot P$.We can prove that$P\to Q$implies$\lnot Q\to \lnot P$(see below), but the reverse implication, from$\lnot Q\to \lnot P$to$P\to Q$,requires thelaw of the excluded middleor an equivalent axiom.

Assume$P\to Q$(initial assumption)

Assume$Q\to \bot$

From$P\to Q$and$Q\to \bot$,conclude$P\to \bot$

Discharge assumption; conclude$(Q\to \bot )\to (P\to \bot )$

Turning$(A\to \bot )$into$\lnot A$,conclude$\lnot Q\to \lnot P$

Discharge assumption; conclude$(P\to Q)\to (\lnot Q\to \lnot P)$.

Subjective logic

Contrapositionrepresents an instance of the subjective Bayes' theorem insubjective logicexpressed as:

$(\omega _{P{\tilde {|}}Q}^{A},\omega _{P{\tilde {|}}\lnot Q}^{A})=(\omega _{Q|P}^{A},\omega _{Q|\lnot P}^{A})\,{\widetilde {\phi \,}}\,a_{P}\,,$

where$(\omega _{Q|P}^{A},\omega _{Q|\lnot P}^{A})$denotes a pair of binomial conditional opinions given by source$A$.The parameter$a_{P}$denotes thebase rate(aka. theprior probability) of$P$.The pair of derivative inverted conditional opinions is denoted$(\omega _{P{\tilde {|}}Q}^{A},\omega _{P{\tilde {|}}\lnot Q}^{A})$.The conditional opinion$\omega _{Q|P}^{A}$generalizes the logical statement$P\to Q$,i.e. in addition to assigning TRUE or FALSE the source$A$can assign any subjective opinion to the statement. The case where$\omega _{Q\mid P}^{A}$is an absolute TRUE opinion is equivalent to source$A$saying that$P\to Q$is TRUE, and the case where$\omega _{Q\mid P}^{A}$is an absolute FALSE opinion is equivalent to source$A$saying that$P\to Q$is FALSE. In the case when the conditional opinion$\omega _{Q|P}^{A}$is absolute TRUE the subjective Bayes' theorem operator${\widetilde {\phi \,}}$ofsubjective logicproduces an absolute FALSE derivative conditional opinion$\omega _{P{\widetilde {|}}\lnot Q}^{A}$and thereby an absolute TRUE derivative conditional opinion$\omega _{\lnot P{\widetilde {|}}\lnot Q}^{A}$which is equivalent to$\lnot Q\to \lnot P$being TRUE. Hence, the subjective Bayes' theorem represents a generalization of bothcontrapositionandBayes' theorem.

In probability theory

Contrapositionrepresents an instance ofBayes' theoremwhich in a specific form can be expressed as:

$\Pr(\lnot P\mid \lnot Q)={\frac {\Pr(\lnot Q\mid \lnot P)\,a(\lnot P)}{\Pr(\lnot Q\mid \lnot P)\,a(\lnot P)+\Pr(\lnot Q\mid P)\,a(P)}}.$

In the equation above theconditional probability$\Pr(\lnot Q\mid P)$generalizes the logical statement$P\to \lnot Q$,i.e. in addition to assigning TRUE or FALSE we can also assign any probability to the statement. The term$a(P)$denotes thebase rate(aka. theprior probability) of$P$.Assume that$\Pr(\lnot Q\mid P)=1$is equivalent to$P\to \lnot Q$being TRUE, and that$\Pr(\lnot Q\mid P)=0$is equivalent to$P\to \lnot Q$being FALSE. It is then easy to see that$\Pr(\lnot P\mid \lnot Q)=1$when$\Pr(Q\mid P)=1$i.e. when$P\to Q$is TRUE. This is because$\Pr(\lnot Q\mid P)=1-\Pr(Q\mid P)=0$so that the fraction on the right-hand side of the equation above is equal to 1, and hence$\Pr(\lnot P\mid \lnot Q)=1$which is equivalent to$\lnot Q\to \lnot P$being TRUE. Hence,Bayes' theoremrepresents a generalization ofcontraposition.

See also

• Reductio ad absurdum
• Logical equivalence

References

• Brody, Bobuch A. (1973). "Glossary of Logical Terms".Encyclopedia of Philosophy.Vol. 5–6. Macmillan. p. 61ff.
• Copi, Irving M. (1953).Introduction to Logic.Macmillan.
• Copi, Irving M.; Cohen, Carl (2005).Introduction to Logic.Prentice Hall.
• Copi, Irving M.; Cohen, Carl; Rodych, Victor (2016).Introduction to Logic.Taylor & Francis.ISBN978-1-315-51087-3.
• Copi, Irving M. (1979).Symbolic Logic(5th ed.). MacMillan.
• Hurley, Patrick J. (2011).A Concise Introduction to Logic(11th ed.). Cengage Learning.ISBN9780840034175.
• Moore, Brooke Noel; Parker, Richard Burl (2020) [1986].Critical thinking(13th ed.). New York City: McGraw-Hill Education.ISBN978-1-260-80787-5.OCLC1122695276.
• Prior, Arthur Norman (1973). "Logic, Traditional".Encyclopedia of Philosophy.Vol. 5. Macmillan.
• Stebbing, L. Susan (1961).A Modern Introduction to Logic(7th ed.). Harper.

Sources

• Audun Jøsang, 2016,Subjective Logic; A formalism for Reasoning Under UncertaintySpringer, Cham,ISBN978-3-319-42337-1
• Blumberg, Albert E. "Logic, Modern".Encyclopedia of Philosophy,Vol.5, Macmillan, 1973.
• Brody, Bobuch A. "Glossary of Logical Terms". Encyclopedia of Philosophy. Vol. 5-6, p. 61. Macmillan, 1973.
• Copi, Irving.Introduction to Logic.MacMillan, 1953.
• Copi, Irving.Symbolic Logic.MacMillan, 1979, fifth edition.
• Prior, A.N. "Logic, Traditional".Encyclopedia of Philosophy,Vol.5, Macmillan, 1973.
• Stebbing, Susan.A Modern Introduction to Logic.Cromwell Company, 1931.

External links

• Media related toContrapositionat Wikimedia Commons
• Improper Transposition(Fallacy Files)