Lorentz transformation

Inphysics,theLorentz transformationsare a six-parameter family oflineartransformationsfrom acoordinate frameinspacetimeto another frame that moves at a constantvelocityrelative to the former. The respective inverse transformation is then parameterized by the negative of this velocity. The transformations are named after the DutchphysicistHendrik Lorentz.

The most common form of the transformation, parametrized by the real constant$v,$representing a velocity confined to thex-direction, is expressed as $${\begin{aligned}t'&=\gamma \left(t-{\frac {vx}{c^{2}}}\right)\\x'&=\gamma \left(x-vt\right)\\y'&=y\\z'&=z\end{aligned}}$$ where(t,x,y,z)and(t′,x′,y′,z′)are the coordinates of an event in two frames with the origins coinciding att=t′=0, where the primed frame is seen from the unprimed frame as moving with speedvalong thex-axis, wherecis thespeed of light,and${\textstyle \gamma =\left({\sqrt {1-{\frac {v^{2}}{c^{2}}}}}\right)^{-1}}$is theLorentz factor.When speedvis much smaller thanc,the Lorentz factor is negligibly different from 1, but asvapproachesc,$\gamma$grows without bound. The value ofvmust be smaller thancfor the transformation to make sense.

Expressing the speed as${\textstyle \beta ={\frac {v}{c}},}$an equivalent form of the transformation is $${\begin{aligned}ct'&=\gamma \left(ct-\beta x\right)\\x'&=\gamma \left(x-\beta ct\right)\\y'&=y\\z'&=z.\end{aligned}}$$

Frames of reference can be divided into two groups:inertial(relative motion with constant velocity) andnon-inertial(accelerating, moving in curved paths, rotational motion with constantangular velocity,etc.). The term "Lorentz transformations" only refers to transformations betweeninertialframes, usually in the context of special relativity.

In eachreference frame,an observer can use a local coordinate system (usuallyCartesian coordinatesin this context) to measure lengths, and a clock to measure time intervals. Aneventis something that happens at a point in space at an instant of time, or more formally a point inspacetime.The transformations connect the space and time coordinates of aneventas measured by an observer in each frame.

They supersede theGalilean transformationofNewtonian physics,which assumes anabsolute space and time(seeGalilean relativity). The Galilean transformation is a good approximation only at relative speeds much less than the speed of light. Lorentz transformations have a number of unintuitive features that do not appear in Galilean transformations. For example, they reflect the fact that observers moving at differentvelocitiesmay measure differentdistances,elapsed times,and even differentorderings of events,but always such that thespeed of lightis the same in all inertial reference frames. The invariance of light speed is one of thepostulates of special relativity.

Historically, the transformations were the result of attempts by Lorentz and others to explain how the speed oflightwas observed to be independent of thereference frame,and to understand the symmetries of the laws ofelectromagnetism.The transformations later became a cornerstone forspecial relativity.

The Lorentz transformation is alinear transformation.It may include a rotation of space; a rotation-free Lorentz transformation is called aLorentz boost.InMinkowski space—the mathematical model of spacetime in special relativity—the Lorentz transformations preserve thespacetime intervalbetween any two events. This property is the defining property of a Lorentz transformation. They describe only the transformations in which the spacetime event at the origin is left fixed. They can be considered as ahyperbolic rotationof Minkowski space. The more general set of transformations that also includes translations is known as thePoincaré group.

History

Main article:History of Lorentz transformations

Many physicists—includingWoldemar Voigt,George FitzGerald,Joseph Larmor,andHendrik Lorentzhimself—had been discussing the physics implied by these equations since 1887.Early in 1889,Oliver Heavisidehad shown fromMaxwell's equationsthat theelectric fieldsurrounding a spherical distribution of charge should cease to havespherical symmetryonce the charge is in motion relative to theluminiferous aether.FitzGerald then conjectured that Heaviside's distortion result might be applied to a theory of intermolecular forces. Some months later, FitzGerald published the conjecture that bodies in motion are being contracted, in order to explain the baffling outcome of the1887 aether-wind experiment of Michelson and Morley.In 1892, Lorentz independently presented the same idea in a more detailed manner, which was subsequently calledFitzGerald–Lorentz contraction hypothesis.Their explanation was widely known before 1905.

Lorentz (1892–1904) and Larmor (1897–1900), who believed the luminiferous aether hypothesis, also looked for the transformation under whichMaxwell's equationsare invariant when transformed from the aether to a moving frame. They extended theFitzGerald–Lorentz contractionhypothesis and found out that the time coordinate has to be modified as well ( "local time").Henri Poincarégave a physical interpretation to local time (to first order inv/c,the relative velocity of the two reference frames normalized to the speed of light) as the consequence of clock synchronization, under the assumption that the speed of light is constant in moving frames.Larmor is credited to have been the first to understand the crucialtime dilationproperty inherent in his equations.

In 1905, Poincaré was the first to recognize that the transformation has the properties of amathematical group, and he named it after Lorentz. Later in the same yearAlbert Einsteinpublished what is now calledspecial relativity,by deriving the Lorentz transformation under the assumptions of theprinciple of relativityand the constancy of the speed of light in anyinertial reference frame,and by abandoning the mechanistic aether as unnecessary.

Derivation of the group of Lorentz transformations

Main articles:Derivations of the Lorentz transformationsandLorentz group

Aneventis something that happens at a certain point in spacetime, or more generally, the point in spacetime itself. In any inertial frame an event is specified by a time coordinatectand a set ofCartesian coordinatesx,y,zto specify position in space in that frame. Subscripts label individual events.

From Einstein'ssecond postulate of relativity(invariance ofc) it follows that:

$$c^{2}(t_{2}-t_{1})^{2}-(x_{2}-x_{1})^{2}-(y_{2}-y_{1})^{2}-(z_{2}-z_{1})^{2}=0\quad {\text{(lightlike separated events 1, 2)}}$$(D1)

in all inertial frames for events connected bylight signals.The quantity on the left is called thespacetime intervalbetween eventsa₁= (t₁,x₁,y₁,z₁)anda₂= (t₂,x₂,y₂,z₂).The interval betweenany twoevents, not necessarily separated by light signals, is in fact invariant, i.e., independent of the state of relative motion of observers in different inertial frames, as isshown using homogeneity and isotropy of space.The transformation sought after thus must possess the property that:

$${\begin{aligned}&c^{2}(t_{2}-t_{1})^{2}-(x_{2}-x_{1})^{2}-(y_{2}-y_{1})^{2}-(z_{2}-z_{1})^{2}\\[6pt]={}&c^{2}(t_{2}'-t_{1}')^{2}-(x_{2}'-x_{1}')^{2}-(y_{2}'-y_{1}')^{2}-(z_{2}'-z_{1}')^{2}\quad {\text{(all events 1, 2)}}.\end{aligned}}$$(D2)

where(t,x,y,z)are the spacetime coordinates used to define events in one frame, and(t′,x′,y′,z′)are the coordinates in another frame. First one observes that (D2) is satisfied if an arbitrary4-tuplebof numbers are added to eventsa₁anda₂.Such transformations are calledspacetime translationsand are not dealt with further here. Then one observes that alinearsolution preserving the origin of the simpler problem solves the general problem too:

$${\begin{aligned}&c^{2}t^{2}-x^{2}-y^{2}-z^{2}=c^{2}t'^{2}-x'^{2}-y'^{2}-z'^{2}\\[6pt]{\text{or}}\quad &c^{2}t_{1}t_{2}-x_{1}x_{2}-y_{1}y_{2}-z_{1}z_{2}=c^{2}t'_{1}t'_{2}-x'_{1}x'_{2}-y'_{1}y'_{2}-z'_{1}z'_{2}\end{aligned}}$$(D3)

(a solution satisfying the first formula automatically satisfies the second one as well; seepolarization identity). Finding the solution to the simpler problem is just a matter of look-up in the theory ofclassical groupsthat preservebilinear formsof various signature.First equation in (D3) can be written more compactly as:

$$(a,a)=(a',a')\quad {\text{or}}\quad a\cdot a=a'\cdot a',$$(D4)

where(·, ·)refers to the bilinear form ofsignature(1, 3)onR⁴exposed by the right hand side formula in (D3). The alternative notation defined on the right is referred to as therelativistic dot product.Spacetime mathematically viewed asR⁴endowed with this bilinear form is known asMinkowski spaceM.The Lorentz transformation is thus an element of the groupO(1, 3),theLorentz groupor, for those that prefer the othermetric signature,O(3, 1)(also called the Lorentz group).One has:

$$(a,a)=(\Lambda a,\Lambda a)=(a',a'),\quad \Lambda \in \mathrm {O} (1,3),\quad a,a'\in M,$$(D5)

which is precisely preservation of the bilinear form (D3) which implies (by linearity ofΛand bilinearity of the form) that (D2) is satisfied. The elements of the Lorentz group arerotationsandboostsand mixes thereof. If the spacetime translations are included, then one obtains theinhomogeneous Lorentz groupor thePoincaré group.

Generalities

The relations between the primed and unprimed spacetime coordinates are theLorentz transformations,each coordinate in one frame is alinear functionof all the coordinates in the other frame, and theinverse functionsare the inverse transformation. Depending on how the frames move relative to each other, and how they are oriented in space relative to each other, other parameters that describe direction, speed, and orientation enter the transformation equations.

Transformations describing relative motion with constant (uniform) velocity and without rotation of the space coordinate axes are calledLorentz boostsor simplyboosts,and the relative velocity between the frames is the parameter of the transformation. The other basic type of Lorentz transformation is rotation in the spatial coordinates only, these like boosts are inertial transformations since there is no relative motion, the frames are simply tilted (and not continuously rotating), and in this case quantities defining the rotation are the parameters of the transformation (e.g.,axis–angle representation,orEuler angles,etc.). A combination of a rotation and boost is ahomogeneous transformation,which transforms the origin back to the origin.

The full Lorentz groupO(3, 1)also contains special transformations that are neither rotations nor boosts, but ratherreflectionsin a plane through the origin. Two of these can be singled out;spatial inversionin which the spatial coordinates of all events are reversed in sign andtemporal inversionin which the time coordinate for each event gets its sign reversed.

Boosts should not be conflated with mere displacements in spacetime; in this case, the coordinate systems are simply shifted and there is no relative motion. However, these also count as symmetries forced by special relativity since they leave the spacetime interval invariant. A combination of a rotation with a boost, followed by a shift in spacetime, is aninhomogeneous Lorentz transformation,an element of the Poincaré group, which is also called the inhomogeneous Lorentz group.

Physical formulation of Lorentz boosts

Further information:Derivations of the Lorentz transformations

Coordinate transformation

The spacetime coordinates of an event, as measured by each observer in their inertial reference frame (in standard configuration) are shown in the speech bubbles.
Top:frameF′moves at velocityvalong thex-axis of frameF.
Bottom:frameFmoves at velocity −valong thex′-axis of frameF′.

A "stationary" observer in frameFdefines events with coordinatest,x,y,z.Another frameF′moves with velocityvrelative toF,and an observer in this "moving" frameF′defines events using the coordinatest′,x′,y′,z′.

The coordinate axes in each frame are parallel (thexandx′axes are parallel, theyandy′axes are parallel, and thezandz′axes are parallel), remain mutually perpendicular, and relative motion is along the coincidentxx′axes. Att=t′ = 0,the origins of both coordinate systems are the same,(x, y, z) = (x′,y′,z′) = (0, 0, 0).In other words, the times and positions are coincident at this event. If all these hold, then the coordinate systems are said to be instandard configuration,orsynchronized.

If an observer inFrecords an eventt,x,y,z,then an observer inF′records thesameevent with coordinates

Lorentz boost(xdirection)

${\begin{aligned}t'&=\gamma \left(t-{\frac {vx}{c^{2}}}\right)\\x'&=\gamma \left(x-vt\right)\\y'&=y\\z'&=z\end{aligned}}$

wherevis the relative velocity between frames in thex-direction,cis thespeed of light,and $$\gamma ={\frac {1}{\sqrt {1-{\frac {v^{2}}{c^{2}}}}}}$$ (lowercasegamma) is theLorentz factor.

Here,vis theparameterof the transformation, for a given boost it is a constant number, but can take a continuous range of values. In the setup used here, positive relative velocityv> 0is motion along the positive directions of thexx′axes, zero relative velocityv= 0is no relative motion, while negative relative velocityv< 0is relative motion along the negative directions of thexx′axes. The magnitude of relative velocityvcannot equal or exceedc,so only subluminal speeds−c<v<care allowed. The corresponding range ofγis1 ≤γ< ∞.

The transformations are not defined ifvis outside these limits. At the speed of light (v=c)γis infinite, andfaster than light(v>c)γis acomplex number,each of which make the transformations unphysical. The space and time coordinates are measurable quantities and numerically must be real numbers.

As anactive transformation,an observer in F′ notices the coordinates of the event to be "boosted" in the negative directions of thexx′axes, because of the−vin the transformations. This has the equivalent effect of thecoordinate systemF′ boosted in the positive directions of thexx′axes, while the event does not change and is simply represented in another coordinate system, apassive transformation.

The inverse relations (t,x,y,zin terms oft′,x′,y′,z′) can be found by algebraically solving the original set of equations. A more efficient way is to use physical principles. HereF′is the "stationary" frame whileFis the "moving" frame. According to the principle of relativity, there is no privileged frame of reference, so the transformations fromF′toFmust take exactly the same form as the transformations fromFtoF′.The only difference isFmoves with velocity−vrelative toF′(i.e., the relative velocity has the same magnitude but is oppositely directed). Thus if an observer inF′notes an eventt′,x′,y′,z′,then an observer inFnotes thesameevent with coordinates

Inverse Lorentz boost(xdirection)

${\begin{aligned}t&=\gamma \left(t'+{\frac {vx'}{c^{2}}}\right)\\x&=\gamma \left(x'+vt'\right)\\y&=y'\\z&=z',\end{aligned}}$

and the value ofγremains unchanged. This "trick" of simply reversing the direction of relative velocity while preserving its magnitude, and exchanging primed and unprimed variables, always applies to finding the inverse transformation of every boost in any direction.

Sometimes it is more convenient to useβ=v/c(lowercasebeta) instead ofv,so that $${\begin{aligned}ct'&=\gamma \left(ct-\beta x\right)\,,\\x'&=\gamma \left(x-\beta ct\right)\,,\\\end{aligned}}$$ which shows much more clearly the symmetry in the transformation. From the allowed ranges ofvand the definition ofβ,it follows−1 <β< 1.The use ofβandγis standard throughout the literature.

The Lorentz transformations can also be derived in a way that resembles circular rotations in 3d space using thehyperbolic functions.For the boost in thexdirection, the results are

Lorentz boost(xdirection with rapidityζ)

${\begin{aligned}ct'&=ct\cosh \zeta -x\sinh \zeta \\x'&=x\cosh \zeta -ct\sinh \zeta \\y'&=y\\z'&=z\end{aligned}}$

whereζ(lowercasezeta) is a parameter calledrapidity(many other symbols are used, includingθ, ϕ, φ, η, ψ, ξ). Given the strong resemblance to rotations of spatial coordinates in 3d space in the Cartesian xy, yz, and zx planes, a Lorentz boost can be thought of as ahyperbolic rotationof spacetime coordinates in the xt, yt, and zt Cartesian-time planes of 4dMinkowski space.The parameterζis thehyperbolic angleof rotation, analogous to the ordinary angle for circular rotations. This transformation can be illustrated with aMinkowski diagram.

The hyperbolic functions arise from thedifferencebetween the squares of the time and spatial coordinates in the spacetime interval, rather than a sum. The geometric significance of the hyperbolic functions can be visualized by takingx= 0orct= 0in the transformations. Squaring and subtracting the results, one can derive hyperbolic curves of constant coordinate values but varyingζ,which parametrizes the curves according to the identity $$\cosh ^{2}\zeta -\sinh ^{2}\zeta =1\,.$$

Conversely thectandxaxes can be constructed for varying coordinates but constantζ.The definition $$\tanh \zeta ={\frac {\sinh \zeta }{\cosh \zeta }}\,,$$ provides the link between a constant value of rapidity, and theslopeof thectaxis in spacetime. A consequence these two hyperbolic formulae is an identity that matches the Lorentz factor $$\cosh \zeta ={\frac {1}{\sqrt {1-\tanh ^{2}\zeta }}}\,.$$

Comparing the Lorentz transformations in terms of the relative velocity and rapidity, or using the above formulae, the connections betweenβ,γ,andζare $${\begin{aligned}\beta &=\tanh \zeta \,,\\\gamma &=\cosh \zeta \,,\\\beta \gamma &=\sinh \zeta \,.\end{aligned}}$$

Taking the inverse hyperbolic tangent gives the rapidity$$\zeta =\tanh ^{-1}\beta \,.$$

Since−1 <β< 1,it follows−∞ <ζ< ∞.From the relation betweenζandβ,positive rapidityζ> 0is motion along the positive directions of thexx′axes, zero rapidityζ= 0is no relative motion, while negative rapidityζ< 0is relative motion along the negative directions of thexx′axes.

The inverse transformations are obtained by exchanging primed and unprimed quantities to switch the coordinate frames, and negating rapidityζ→ −ζsince this is equivalent to negating the relative velocity. Therefore,

Inverse Lorentz boost(xdirection with rapidityζ)

${\begin{aligned}ct&=ct'\cosh \zeta +x'\sinh \zeta \\x&=x'\cosh \zeta +ct'\sinh \zeta \\y&=y'\\z&=z'\end{aligned}}$

The inverse transformations can be similarly visualized by considering the cases whenx′ = 0andct′ = 0.

So far the Lorentz transformations have been applied toone event.If there are two events, there is a spatial separation and time interval between them. It follows from thelinearityof the Lorentz transformations that two values of space and time coordinates can be chosen, the Lorentz transformations can be applied to each, then subtracted to get the Lorentz transformations of the differences;

$${\begin{aligned}\Delta t'&=\gamma \left(\Delta t-{\frac {v\,\Delta x}{c^{2}}}\right)\,,\\\Delta x'&=\gamma \left(\Delta x-v\,\Delta t\right)\,,\end{aligned}}$$ with inverse relations $${\begin{aligned}\Delta t&=\gamma \left(\Delta t'+{\frac {v\,\Delta x'}{c^{2}}}\right)\,,\\\Delta x&=\gamma \left(\Delta x'+v\,\Delta t'\right)\,.\end{aligned}}$$

whereΔ(uppercasedelta) indicates a difference of quantities; e.g.,Δx=x₂−x₁for two values ofxcoordinates, and so on.

These transformations ondifferencesrather than spatial points or instants of time are useful for a number of reasons:

• in calculations and experiments, it is lengths between two points or time intervals that are measured or of interest (e.g., the length of a moving vehicle, or time duration it takes to travel from one place to another),
• the transformations of velocity can be readily derived by making the difference infinitesimally small and dividing the equations, and the process repeated for the transformation of acceleration,
• if the coordinate systems are never coincident (i.e., not in standard configuration), and if both observers can agree on an eventt₀,x₀,y₀,z₀inFandt₀′,x₀′,y₀′,z₀′inF′,then they can use that event as the origin, and the spacetime coordinate differences are the differences between their coordinates and this origin, e.g.,Δx=x−x₀,Δx′ =x′ −x₀′,etc.

Physical implications

A critical requirement of the Lorentz transformations is the invariance of the speed of light, a fact used in their derivation, and contained in the transformations themselves. If inFthe equation for a pulse of light along thexdirection isx=ct,then inF′the Lorentz transformations givex′ =ct′,and vice versa, for any−c<v<c.

For relative speeds much less than the speed of light, the Lorentz transformations reduce to theGalilean transformation $${\begin{aligned}t'&\approx t\\x'&\approx x-vt\end{aligned}}$$ in accordance with thecorrespondence principle.It is sometimes said that nonrelativistic physics is a physics of "instantaneous action at a distance".

Three counterintuitive, but correct, predictions of the transformations are:

Relativity of simultaneity

Suppose two events occur along the x axis simultaneously (Δt= 0) inF,but separated by a nonzero displacementΔx.Then inF′,we find that$\Delta t'=\gamma {\frac {-v\,\Delta x}{c^{2}}}$,so the events are no longer simultaneous according to a moving observer.

Time dilation

Suppose there is a clock at rest inF.If a time interval is measured at the same point in that frame, so thatΔx= 0,then the transformations give this interval inF′byΔt′ =γΔt.Conversely, suppose there is a clock at rest inF′.If an interval is measured at the same point in that frame, so thatΔx′ = 0,then the transformations give this interval in F byΔt=γΔt′.Either way, each observer measures the time interval between ticks of a moving clock to be longer by a factorγthan the time interval between ticks of his own clock.

Length contraction

Suppose there is a rod at rest inFaligned along the x axis, with lengthΔx.InF′,the rod moves with velocity-v,so its length must be measured by taking two simultaneous (Δt′ = 0) measurements at opposite ends. Under these conditions, the inverse Lorentz transform shows thatΔx=γΔx′.InFthe two measurements are no longer simultaneous, but this does not matter because the rod is at rest inF.So each observer measures the distance between the end points of a moving rod to be shorter by a factor1/γthan the end points of an identical rod at rest in his own frame. Length contraction affects any geometric quantity related to lengths, so from the perspective of a moving observer, areas and volumes will also appear to shrink along the direction of motion.

Vector transformations

Further information:Euclidean vectorandvector projection

An observer in frameFobservesF′to move with velocityv,whileF′observesFto move with velocity−v.The coordinate axes of each frame are still paralleland orthogonal. The position vector as measured in each frame is split into components parallel and perpendicular to the relative velocity vectorv.
Left:Standard configuration.Right:Inverse configuration.

The use of vectors allows positions and velocities to be expressed in arbitrary directions compactly. A single boost in any direction depends on the full relativevelocity vectorvwith a magnitude|v| =vthat cannot equal or exceedc,so that0 ≤v<c.

Only time and the coordinates parallel to the direction of relative motion change, while those coordinates perpendicular do not. With this in mind, split the spatialposition vectorras measured inF,andr′as measured inF′,each into components perpendicular (⊥) and parallel ( ‖ ) tov, $$\mathbf {r} =\mathbf {r} _{\perp }+\mathbf {r} _{\|}\,,\quad \mathbf {r} '=\mathbf {r} _{\perp }'+\mathbf {r} _{\|}'\,,$$ then the transformations are $${\begin{aligned}t'&=\gamma \left(t-{\frac {\mathbf {r} _{\parallel }\cdot \mathbf {v} }{c^{2}}}\right)\\\mathbf {r} _{\|}'&=\gamma (\mathbf {r} _{\|}-\mathbf {v} t)\\\mathbf {r} _{\perp }'&=\mathbf {r} _{\perp }\end{aligned}}$$ where·is thedot product.The Lorentz factorγretains its definition for a boost in any direction, since it depends only on the magnitude of the relative velocity. The definitionβ=v/cwith magnitude0 ≤β< 1is also used by some authors.

Introducing aunit vectorn=v/v=β/βin the direction of relative motion, the relative velocity isv=vnwith magnitudevand directionn,andvector projectionand rejection give respectively $$\mathbf {r} _{\parallel }=(\mathbf {r} \cdot \mathbf {n} )\mathbf {n} \,,\quad \mathbf {r} _{\perp }=\mathbf {r} -(\mathbf {r} \cdot \mathbf {n} )\mathbf {n}$$

Accumulating the results gives the full transformations,

Lorentz boost(in directionnwith magnitudev)

${\begin{aligned}t'&=\gamma \left(t-{\frac {v\mathbf {n} \cdot \mathbf {r} }{c^{2}}}\right)\,,\\\mathbf {r} '&=\mathbf {r} +(\gamma -1)(\mathbf {r} \cdot \mathbf {n} )\mathbf {n} -\gamma tv\mathbf {n} \,.\end{aligned}}$

The projection and rejection also applies tor′.For the inverse transformations, exchangerandr′to switch observed coordinates, and negate the relative velocityv→ −v(or simply the unit vectorn→ −nsince the magnitudevis always positive) to obtain

Inverse Lorentz boost(in directionnwith magnitudev)

${\begin{aligned}t&=\gamma \left(t'+{\frac {\mathbf {r} '\cdot v\mathbf {n} }{c^{2}}}\right)\,,\\\mathbf {r} &=\mathbf {r} '+(\gamma -1)(\mathbf {r} '\cdot \mathbf {n} )\mathbf {n} +\gamma t'v\mathbf {n} \,,\end{aligned}}$

The unit vector has the advantage of simplifying equations for a single boost, allows eithervorβto be reinstated when convenient, and the rapidity parametrization is immediately obtained by replacingβandβγ.It is not convenient for multiple boosts.

The vectorial relation between relative velocity and rapidity is $${\boldsymbol {\beta }}=\beta \mathbf {n} =\mathbf {n} \tanh \zeta \,,$$ and the "rapidity vector" can be defined as $${\boldsymbol {\zeta }}=\zeta \mathbf {n} =\mathbf {n} \tanh ^{-1}\beta \,,$$ each of which serves as a useful abbreviation in some contexts. The magnitude ofζis the absolute value of the rapidity scalar confined to0 ≤ζ< ∞,which agrees with the range0 ≤β< 1.

Transformation of velocities

Further information:differential of a functionandvelocity addition formula

The transformation of velocities provides the definitionrelativistic velocity addition⊕,the ordering of vectors is chosen to reflect the ordering of the addition of velocities; firstv(the velocity of F′ relative to F) thenu′(the velocity of X relative to F′) to obtainu=v⊕u′(the velocity of X relative to F).

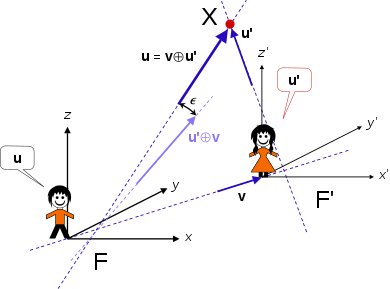
Defining the coordinate velocities and Lorentz factor by

$\mathbf {u} ={\frac {d\mathbf {r} }{dt}}\,,\quad \mathbf {u} '={\frac {d\mathbf {r} '}{dt'}}\,,\quad \gamma _{\mathbf {v} }={\frac {1}{\sqrt {1-{\dfrac {\mathbf {v} \cdot \mathbf {v} }{c^{2}}}}}}$

taking the differentials in the coordinates and time of the vector transformations, then dividing equations, leads to

$\mathbf {u} '={\frac {1}{1-{\frac {\mathbf {v} \cdot \mathbf {u} }{c^{2}}}}}\left[{\frac {\mathbf {u} }{\gamma _{\mathbf {v} }}}-\mathbf {v} +{\frac {1}{c^{2}}}{\frac {\gamma _{\mathbf {v} }}{\gamma _{\mathbf {v} }+1}}\left(\mathbf {u} \cdot \mathbf {v} \right)\mathbf {v} \right]$

The velocitiesuandu′are the velocity of some massive object. They can also be for a third inertial frame (sayF′′), in which case they must beconstant.Denote either entity by X. Then X moves with velocityurelative to F, or equivalently with velocityu′relative to F′, in turn F′ moves with velocityvrelative to F. The inverse transformations can be obtained in a similar way, or as with position coordinates exchangeuandu′,and changevto−v.

The transformation of velocity is useful instellar aberration,theFizeau experiment,and therelativistic Doppler effect.

TheLorentz transformations of accelerationcan be similarly obtained by taking differentials in the velocity vectors, and dividing these by the time differential.

Transformation of other quantities

In general, given four quantitiesAandZ= (Z_x,Z_y,Z_z)and their Lorentz-boosted counterpartsA′andZ′ = (Z′_x,Z′_y,Z′_z),a relation of the form $$A^{2}-\mathbf {Z} \cdot \mathbf {Z} ={A'}^{2}-\mathbf {Z} '\cdot \mathbf {Z} '$$ implies the quantities transform under Lorentz transformations similar to the transformation of spacetime coordinates; $${\begin{aligned}A'&=\gamma \left(A-{\frac {v\mathbf {n} \cdot \mathbf {Z} }{c}}\right)\,,\\\mathbf {Z} '&=\mathbf {Z} +(\gamma -1)(\mathbf {Z} \cdot \mathbf {n} )\mathbf {n} -{\frac {\gamma Av\mathbf {n} }{c}}\,.\end{aligned}}$$

The decomposition ofZ(andZ′) into components perpendicular and parallel tovis exactly the same as for the position vector, as is the process of obtaining the inverse transformations (exchange(A,Z)and(A′,Z′)to switch observed quantities, and reverse the direction of relative motion by the substitutionn↦ −n).

The quantities(A,Z)collectively make up afour-vector,whereAis the "timelike component", andZthe "spacelike component". Examples ofAandZare the following:

Four-vector	A	Z
Positionfour-vector	Time(multiplied byc),ct	Position vector,r
Four-momentum	Energy(divided byc),E/c	Momentum,p
Four-wave vector	angular frequency(divided byc),ω/c	wave vector,k
Four-spin	(No name),st	Spin,s
Four-current	Charge density(multiplied byc),ρc	Current density,j
Electromagnetic four-potential	Electric potential(divided byc),φ/c	Magnetic vector potential,A

For a given object (e.g., particle, fluid, field, material), ifAorZcorrespond to properties specific to the object like itscharge density,mass density,spin,etc., its properties can be fixed in the rest frame of that object. Then the Lorentz transformations give the corresponding properties in a frame moving relative to the object with constant velocity. This breaks some notions taken for granted in non-relativistic physics. For example, the energyEof an object is a scalar in non-relativistic mechanics, but not in relativistic mechanics because energy changes under Lorentz transformations; its value is different for various inertial frames. In the rest frame of an object, it has arest energyand zero momentum. In a boosted frame its energy is different and it appears to have a momentum. Similarly, in non-relativistic quantum mechanics the spin of a particle is a constant vector, but inrelativistic quantum mechanicsspinsdepends on relative motion. In the rest frame of the particle, the spin pseudovector can be fixed to be its ordinary non-relativistic spin with a zero timelike quantitys_t,however a boosted observer will perceive a nonzero timelike component and an altered spin.

Not all quantities are invariant in the form as shown above, for example orbitalangular momentumLdoes not have a timelike quantity, and neither does theelectric fieldEnor themagnetic fieldB.The definition of angular momentum isL=r×p,and in a boosted frame the altered angular momentum isL′ =r′ ×p′.Applying this definition using the transformations of coordinates and momentum leads to the transformation of angular momentum. It turns outLtransforms with another vector quantityN= (E/c²)r−tprelated to boosts, seerelativistic angular momentumfor details. For the case of theEandBfields, the transformations cannot be obtained as directly using vector algebra. TheLorentz forceis the definition of these fields, and inFit isF=q(E+v×B)while inF′it isF′ =q(E′ +v′ ×B′).A method of deriving the EM field transformations in an efficient way which also illustrates the unit of the electromagnetic field uses tensor algebra,given below.

Mathematical formulation

Main article:Lorentz group

Further information:Matrix (mathematics),matrix product,linear algebra,androtation formalisms in three dimensions

Throughout, italic non-bold capital letters are 4×4 matrices, while non-italic bold letters are 3×3 matrices.

Homogeneous Lorentz group

Writing the coordinates in column vectors and theMinkowski metricηas a square matrix $$X'={\begin{bmatrix}c\,t'\\x'\\y'\\z'\end{bmatrix}}\,,\quad \eta ={\begin{bmatrix}-1&0&0&0\\0&1&0&0\\0&0&1&0\\0&0&0&1\end{bmatrix}}\,,\quad X={\begin{bmatrix}c\,t\\x\\y\\z\end{bmatrix}}$$ the spacetime interval takes the form (superscriptTdenotestranspose) $$X\cdot X=X^{\mathrm {T} }\eta X={X'}^{\mathrm {T} }\eta {X'}$$ and isinvariantunder a Lorentz transformation $$X'=\Lambda X$$ whereΛis a square matrix which can depend on parameters.

Thesetof all Lorentz transformations$\Lambda$in this article is denoted${\mathcal {L}}$.This set together with matrix multiplication forms agroup,in this context known as theLorentz group.Also, the above expressionX·Xis aquadratic formof signature (3,1) on spacetime, and the group of transformations which leaves this quadratic form invariant is theindefinite orthogonal groupO(3,1), aLie group.In other words, the Lorentz group is O(3,1). As presented in this article, any Lie groups mentioned arematrix Lie groups.In this context the operation of composition amounts tomatrix multiplication.

From the invariance of the spacetime interval it follows $$\eta =\Lambda ^{\mathrm {T} }\eta \Lambda$$ and this matrix equation contains the general conditions on the Lorentz transformation to ensure invariance of the spacetime interval. Taking thedeterminantof the equation using the product rulegives immediately $$\left[\det(\Lambda )\right]^{2}=1\quad \Rightarrow \quad \det(\Lambda )=\pm 1$$

Writing the Minkowski metric as a block matrix, and the Lorentz transformation in the most general form, $$\eta ={\begin{bmatrix}-1&0\\0&\mathbf {I} \end{bmatrix}}\,,\quad \Lambda ={\begin{bmatrix}\Gamma &-\mathbf {a} ^{\mathrm {T} }\\-\mathbf {b} &\mathbf {M} \end{bmatrix}}\,,$$ carrying out the block matrix multiplications obtains general conditions onΓ,a,b,Mto ensure relativistic invariance. Not much information can be directly extracted from all the conditions, however one of the results $$\Gamma ^{2}=1+\mathbf {b} ^{\mathrm {T} }\mathbf {b}$$ is useful;b^Tb≥ 0always so it follows that $$\Gamma ^{2}\geq 1\quad \Rightarrow \quad \Gamma \leq -1\,,\quad \Gamma \geq 1$$

The negative inequality may be unexpected, becauseΓmultiplies the time coordinate and this has an effect ontime symmetry.If the positive equality holds, thenΓis the Lorentz factor.

The determinant and inequality provide four ways to classifyLorentzTransformations (hereinLTs for brevity). Any particular LT has only one determinant signandonly one inequality. There are four sets which include every possible pair given by theintersections( "n" -shaped symbol meaning "and" ) of these classifying sets.

Intersection, ∩	Antichronous(or non-orthochronous) LTs ${\mathcal {L}}^{\downarrow }=\{\Lambda \,:\,\Gamma \leq -1\}$	OrthochronousLTs ${\mathcal {L}}^{\uparrow }=\{\Lambda \,:\,\Gamma \geq 1\}$
ProperLTs ${\mathcal {L}}_{+}=\{\Lambda \,:\,\det(\Lambda )=+1\}$	Proper antichronousLTs ${\mathcal {L}}_{+}^{\downarrow }={\mathcal {L}}_{+}\cap {\mathcal {L}}^{\downarrow }$	Proper orthochronousLTs ${\mathcal {L}}_{+}^{\uparrow }={\mathcal {L}}_{+}\cap {\mathcal {L}}^{\uparrow }$
ImproperLTs ${\mathcal {L}}_{-}=\{\Lambda \,:\,\det(\Lambda )=-1\}$	Improper antichronousLTs ${\mathcal {L}}_{-}^{\downarrow }={\mathcal {L}}_{-}\cap {\mathcal {L}}^{\downarrow }$	Improper orthochronousLTs ${\mathcal {L}}_{-}^{\uparrow }={\mathcal {L}}_{-}\cap {\mathcal {L}}^{\uparrow }$

where "+" and "−" indicate the determinant sign, while "↑" for ≥ and "↓" for ≤ denote the inequalities.

The full Lorentz group splits into theunion( "u" -shaped symbol meaning "or" ) of fourdisjoint sets $${\mathcal {L}}={\mathcal {L}}_{+}^{\uparrow }\cup {\mathcal {L}}_{-}^{\uparrow }\cup {\mathcal {L}}_{+}^{\downarrow }\cup {\mathcal {L}}_{-}^{\downarrow }$$

Asubgroupof a group must beclosedunder the same operation of the group (here matrix multiplication). In other words, for two Lorentz transformationsΛandLfrom a particular subgroup, the composite Lorentz transformationsΛLandLΛmust be in the same subgroup asΛandL.This is not always the case: the composition of two antichronous Lorentz transformations is orthochronous, and the composition of two improper Lorentz transformations is proper. In other words, while the sets${\mathcal {L}}_{+}^{\uparrow }$,${\mathcal {L}}_{+}$,${\mathcal {L}}^{\uparrow }$,and${\mathcal {L}}_{0}={\mathcal {L}}_{+}^{\uparrow }\cup {\mathcal {L}}_{-}^{\downarrow }$all form subgroups, the sets containing improper and/or antichronous transformations without enough proper orthochronous transformations (e.g.${\mathcal {L}}_{+}^{\downarrow }$,${\mathcal {L}}_{-}^{\downarrow }$,${\mathcal {L}}_{-}^{\uparrow }$) do not form subgroups.

Proper transformations

If a Lorentz covariant 4-vector is measured in one inertial frame with result$X$,and the same measurement made in another inertial frame (with the same orientation and origin) gives result$X'$,the two results will be related by $$X'=B(\mathbf {v} )X$$ where the boost matrix$B(\mathbf {v} )$represents the rotation-free Lorentz transformation between the unprimed and primed frames and$\mathbf {v}$is the velocity of the primed frame as seen from the unprimed frame. The matrix is given by $$B(\mathbf {v} )={\begin{bmatrix}\gamma &-\gamma v_{x}/c&-\gamma v_{y}/c&-\gamma v_{z}/c\\-\gamma v_{x}/c&1+(\gamma -1){\dfrac {v_{x}^{2}}{v^{2}}}&(\gamma -1){\dfrac {v_{x}v_{y}}{v^{2}}}&(\gamma -1){\dfrac {v_{x}v_{z}}{v^{2}}}\\-\gamma v_{y}/c&(\gamma -1){\dfrac {v_{y}v_{x}}{v^{2}}}&1+(\gamma -1){\dfrac {v_{y}^{2}}{v^{2}}}&(\gamma -1){\dfrac {v_{y}v_{z}}{v^{2}}}\\-\gamma v_{z}/c&(\gamma -1){\dfrac {v_{z}v_{x}}{v^{2}}}&(\gamma -1){\dfrac {v_{z}v_{y}}{v^{2}}}&1+(\gamma -1){\dfrac {v_{z}^{2}}{v^{2}}}\end{bmatrix}}={\begin{bmatrix}\gamma &-\gamma {\vec {\beta }}^{T}\\-\gamma {\vec {\beta }}&I+(\gamma -1){\dfrac {{\vec {\beta }}{\vec {\beta }}^{T}}{\beta ^{2}}}\end{bmatrix}},$$

where${\textstyle v={\sqrt {v_{x}^{2}+v_{y}^{2}+v_{z}^{2}}}}$is the magnitude of the velocity and${\textstyle \gamma ={\frac {1}{\sqrt {1-{\frac {v^{2}}{c^{2}}}}}}}$is the Lorentz factor. This formula represents a passive transformation, as it describes how the coordinates of the measured quantity changes from the unprimed frame to the primed frame. The active transformation is given by$B(-\mathbf {v} )$.

If a frameF′is boosted with velocityurelative to frameF,and another frameF′′is boosted with velocityvrelative toF′,the separate boosts are $$X''=B(\mathbf {v} )X'\,,\quad X'=B(\mathbf {u} )X$$ and the composition of the two boosts connects the coordinates inF′′andF, $$X''=B(\mathbf {v} )B(\mathbf {u} )X\,.$$ Successive transformations act on the left. Ifuandvarecollinear(parallel or antiparallel along the same line of relative motion), the boost matricescommute:B(v)B(u) =B(u)B(v).This composite transformation happens to be another boost,B(w),wherewis collinear withuandv.

Ifuandvare not collinear but in different directions, the situation is considerably more complicated. Lorentz boosts along different directions do not commute:B(v)B(u)andB(u)B(v)are not equal. Although each of these compositions isnota single boost, each composition is still a Lorentz transformation as it preserves the spacetime interval. It turns out the composition of any two Lorentz boosts is equivalent to a boost followed or preceded by a rotation on the spatial coordinates, in the form ofR(ρ)B(w)orB(w)R(ρ).Thewandwarecomposite velocities,whileρandρare rotation parameters (e.g.axis-anglevariables,Euler angles,etc.). The rotation inblock matrixform is simply $$\quad R({\boldsymbol {\rho }})={\begin{bmatrix}1&0\\0&\mathbf {R} ({\boldsymbol {\rho }})\end{bmatrix}}\,,$$ whereR(ρ)is a 3drotation matrix,which rotates any 3d vector in one sense (active transformation), or equivalently the coordinate frame in the opposite sense (passive transformation). It isnotsimple to connectwandρ(orwandρ) to the original boost parametersuandv.In a composition of boosts, theRmatrix is named theWigner rotation,and gives rise to theThomas precession.These articles give the explicit formulae for the composite transformation matrices, including expressions forw,ρ,w,ρ.

In this article theaxis-angle representationis used forρ.The rotation is about an axis in the direction of aunit vectore,through angleθ(positive anticlockwise, negative clockwise, according to theright-hand rule). The "axis-angle vector" $${\boldsymbol {\theta }}=\theta \mathbf {e}$$ will serve as a useful abbreviation.

Spatial rotations alone are also Lorentz transformations since they leave the spacetime interval invariant. Like boosts, successive rotations about different axes do not commute. Unlike boosts, the composition of any two rotations is equivalent to a single rotation. Some other similarities and differences between the boost and rotation matrices include:

• inverses:B(v)⁻¹=B(−v)(relative motion in the opposite direction), andR(θ)⁻¹=R(−θ)(rotation in the opposite sense about the same axis)
• identity transformationfor no relative motion/rotation:B(0) =R(0) =I
• unitdeterminant:det(B) = det(R) = +1.This property makes them proper transformations.
• matrix symmetry:Bis symmetric (equalstranspose), whileRis nonsymmetric butorthogonal(transpose equalsinverse,R^T=R⁻¹).

The most general proper Lorentz transformationΛ(v,θ)includes a boost and rotation together, and is a nonsymmetric matrix. As special cases,Λ(0,θ) =R(θ)andΛ(v,0) =B(v).An explicit form of the general Lorentz transformation is cumbersome to write down and will not be given here. Nevertheless, closed form expressions for the transformation matrices will be given below using group theoretical arguments. It will be easier to use the rapidity parametrization for boosts, in which case one writesΛ(ζ,θ)andB(ζ).

The Lie group SO⁺(3,1)

The set of transformations $$\{B({\boldsymbol {\zeta }}),R({\boldsymbol {\theta }}),\Lambda ({\boldsymbol {\zeta }},{\boldsymbol {\theta }})\}$$ with matrix multiplication as the operation of composition forms a group, called the "restricted Lorentz group", and is thespecial indefinite orthogonal groupSO⁺(3,1). (The plus sign indicates that it preserves the orientation of the temporal dimension).

For simplicity, look at the infinitesimal Lorentz boost in the x direction (examining a boost in any other direction, or rotation about any axis, follows an identical procedure). The infinitesimal boost is a small boost away from the identity, obtained by theTaylor expansionof the boost matrix to first order aboutζ= 0, $$B_{x}=I+\zeta \left.{\frac {\partial B_{x}}{\partial \zeta }}\right|_{\zeta =0}+\cdots$$ where the higher order terms not shown are negligible becauseζis small, andB_xis simply the boost matrix in thexdirection. Thederivative of the matrixis the matrix of derivatives (of the entries, with respect to the same variable), and it is understood the derivatives are found first then evaluated atζ= 0, $$\left.{\frac {\partial B_{x}}{\partial \zeta }}\right|_{\zeta =0}=-K_{x}\,.$$

For now,K_xis defined by this result (its significance will be explained shortly). In the limit of an infinite number of infinitely small steps, the finite boost transformation in the form of amatrix exponentialis obtained $$B_{x}=\lim _{N\to \infty }\left(I-{\frac {\zeta }{N}}K_{x}\right)^{N}=e^{-\zeta K_{x}}$$ where thelimit definition of the exponentialhas been used (see alsocharacterizations of the exponential function). More generally

$$B({\boldsymbol {\zeta }})=e^{-{\boldsymbol {\zeta }}\cdot \mathbf {K} }\,,\quad R({\boldsymbol {\theta }})=e^{{\boldsymbol {\theta }}\cdot \mathbf {J} }\,.$$

The axis-angle vectorθand rapidity vectorζare altogether six continuous variables which make up the group parameters (in this particular representation), and the generators of the group areK= (K_x,K_y,K_z)andJ= (J_x,J_y,J_z),each vectors of matrices with the explicit forms

$${\begin{alignedat}{3}K_{x}&={\begin{bmatrix}0&1&0&0\\1&0&0&0\\0&0&0&0\\0&0&0&0\\\end{bmatrix}}\,,\quad &K_{y}&={\begin{bmatrix}0&0&1&0\\0&0&0&0\\1&0&0&0\\0&0&0&0\end{bmatrix}}\,,\quad &K_{z}&={\begin{bmatrix}0&0&0&1\\0&0&0&0\\0&0&0&0\\1&0&0&0\end{bmatrix}}\\[10mu]J_{x}&={\begin{bmatrix}0&0&0&0\\0&0&0&0\\0&0&0&-1\\0&0&1&0\\\end{bmatrix}}\,,\quad &J_{y}&={\begin{bmatrix}0&0&0&0\\0&0&0&1\\0&0&0&0\\0&-1&0&0\end{bmatrix}}\,,\quad &J_{z}&={\begin{bmatrix}0&0&0&0\\0&0&-1&0\\0&1&0&0\\0&0&0&0\end{bmatrix}}\end{alignedat}}$$

These are all defined in an analogous way toK_xabove, although the minus signs in the boost generators are conventional. Physically, the generators of the Lorentz group correspond to important symmetries in spacetime:Jare therotation generatorswhich correspond toangular momentum,andKare theboost generatorswhich correspond to the motion of the system in spacetime. The derivative of any smooth curveC(t)withC(0) =Iin the group depending on some group parametertwith respect to that group parameter, evaluated att= 0,serves as a definition of a corresponding group generatorG,and this reflects an infinitesimal transformation away from the identity. The smooth curve can always be taken as an exponential as the exponential will always mapGsmoothly back into the group viat→ exp(tG)for allt;this curve will yieldGagain when differentiated att= 0.

Expanding the exponentials in their Taylor series obtains $$B({\boldsymbol {\zeta }})=I-\sinh \zeta (\mathbf {n} \cdot \mathbf {K} )+(\cosh \zeta -1)(\mathbf {n} \cdot \mathbf {K} )^{2}$$ $$R({\boldsymbol {\theta }})=I+\sin \theta (\mathbf {e} \cdot \mathbf {J} )+(1-\cos \theta )(\mathbf {e} \cdot \mathbf {J} )^{2}\,.$$ which compactly reproduce the boost and rotation matrices as given in the previous section.

It has been stated that the general proper Lorentz transformation is a product of a boost and rotation. At theinfinitesimallevel the product $${\begin{aligned}\Lambda &=(I-{\boldsymbol {\zeta }}\cdot \mathbf {K} +\cdots )(I+{\boldsymbol {\theta }}\cdot \mathbf {J} +\cdots )\\&=(I+{\boldsymbol {\theta }}\cdot \mathbf {J} +\cdots )(I-{\boldsymbol {\zeta }}\cdot \mathbf {K} +\cdots )\\&=I-{\boldsymbol {\zeta }}\cdot \mathbf {K} +{\boldsymbol {\theta }}\cdot \mathbf {J} +\cdots \end{aligned}}$$ is commutative because only linear terms are required (products like(θ·J)(ζ·K)and(ζ·K)(θ·J)count as higher order terms and are negligible). Taking the limit as before leads to the finite transformation in the form of an exponential $$\Lambda ({\boldsymbol {\zeta }},{\boldsymbol {\theta }})=e^{-{\boldsymbol {\zeta }}\cdot \mathbf {K} +{\boldsymbol {\theta }}\cdot \mathbf {J} }.$$

The converse is also true, but the decomposition of a finite general Lorentz transformation into such factors is nontrivial. In particular, $$e^{-{\boldsymbol {\zeta }}\cdot \mathbf {K} +{\boldsymbol {\theta }}\cdot \mathbf {J} }\neq e^{-{\boldsymbol {\zeta }}\cdot \mathbf {K} }e^{{\boldsymbol {\theta }}\cdot \mathbf {J} },$$ because the generators do not commute. For a description of how to find the factors of a general Lorentz transformation in terms of a boost and a rotationin principle(this usually does not yield an intelligible expression in terms of generatorsJandK), seeWigner rotation.If, on the other hand,the decomposition is givenin terms of the generators, and one wants to find the product in terms of the generators, then theBaker–Campbell–Hausdorff formulaapplies.

The Lie algebra so(3,1)

Lorentz generators can be added together, or multiplied by real numbers, to obtain more Lorentz generators. In other words, thesetof all Lorentz generators $$V=\{{\boldsymbol {\zeta }}\cdot \mathbf {K} +{\boldsymbol {\theta }}\cdot \mathbf {J} \}$$ together with the operations of ordinarymatrix additionandmultiplication of a matrix by a number,forms avector spaceover the real numbers.The generatorsJ_x,J_y,J_z,K_x,K_y,K_zform abasisset ofV,and the components of the axis-angle and rapidity vectors,θ_x,θ_y,θ_z,ζ_x,ζ_y,ζ_z,are thecoordinatesof a Lorentz generator with respect to this basis.

Three of thecommutation relationsof the Lorentz generators are $$[J_{x},J_{y}]=J_{z}\,,\quad [K_{x},K_{y}]=-J_{z}\,,\quad [J_{x},K_{y}]=K_{z}\,,$$ where the bracket[A,B] =AB−BAis known as thecommutator,and the other relations can be found by takingcyclic permutationsof x, y, z components (i.e. change x to y, y to z, and z to x, repeat).

These commutation relations, and the vector space of generators, fulfill the definition of theLie algebra${\mathfrak {so}}(3,1)$.In summary, a Lie algebra is defined as avector spaceVover afieldof numbers, and with abinary operation[, ] (called aLie bracketin this context) on the elements of the vector space, satisfying the axioms ofbilinearity,alternatization,and theJacobi identity.Here the operation [, ] is the commutator which satisfies all of these axioms, the vector space is the set of Lorentz generatorsVas given previously, and the field is the set of real numbers.

Linking terminology used in mathematics and physics: A group generator is any element of the Lie algebra. A group parameter is a component of a coordinate vector representing an arbitrary element of the Lie algebra with respect to some basis. A basis, then, is a set of generators being a basis of the Lie algebra in the usual vector space sense.

Theexponential mapfrom the Lie algebra to the Lie group, $$\exp \,:\,{\mathfrak {so}}(3,1)\to \mathrm {SO} (3,1),$$ provides a one-to-one correspondence between small enough neighborhoods of the origin of the Lie algebra and neighborhoods of the identity element of the Lie group. In the case of the Lorentz group, the exponential map is just thematrix exponential.Globally, the exponential map is not one-to-one, but in the case of the Lorentz group, it issurjective(onto). Hence any group element in the connected component of the identity can be expressed as an exponential of an element of the Lie algebra.

Improper transformations

Lorentz transformations also includeparity inversion $$P={\begin{bmatrix}1&0\\0&-\mathbf {I} \end{bmatrix}}$$ which negates all the spatial coordinates only, andtime reversal $$T={\begin{bmatrix}-1&0\\0&\mathbf {I} \end{bmatrix}}$$ which negates the time coordinate only, because these transformations leave the spacetime interval invariant. HereIis the 3didentity matrix.These are both symmetric, they are their own inverses (seeinvolution (mathematics)), and each have determinant −1. This latter property makes them improper transformations.

IfΛis a proper orthochronous Lorentz transformation, thenTΛis improper antichronous,PΛis improper orthochronous, andTPΛ =PTΛis proper antichronous.

Inhomogeneous Lorentz group

Two other spacetime symmetries have not been accounted for. In order for the spacetime interval to be invariant, it can be shownthat it is necessary and sufficient for the coordinate transformation to be of the form $$X'=\Lambda X+C$$ whereCis a constant column containing translations in time and space. IfC≠ 0, this is aninhomogeneous Lorentz transformationorPoincaré transformation.IfC= 0, this is ahomogeneous Lorentz transformation.Poincaré transformations are not dealt further in this article.

Tensor formulation

Main article:Representation theory of the Lorentz group

For the notation used, seeRicci calculus.

Contravariant vectors

Writing the general matrix transformation of coordinates as the matrix equation $${\begin{bmatrix}{x'}^{0}\\{x'}^{1}\\{x'}^{2}\\{x'}^{3}\end{bmatrix}}={\begin{bmatrix}{\Lambda ^{0}}_{0}&{\Lambda ^{0}}_{1}&{\Lambda ^{0}}_{2}&{\Lambda ^{0}}_{3}\\{\Lambda ^{1}}_{0}&{\Lambda ^{1}}_{1}&{\Lambda ^{1}}_{2}&{\Lambda ^{1}}_{3}\\{\Lambda ^{2}}_{0}&{\Lambda ^{2}}_{1}&{\Lambda ^{2}}_{2}&{\Lambda ^{2}}_{3}\\{\Lambda ^{3}}_{0}&{\Lambda ^{3}}_{1}&{\Lambda ^{3}}_{2}&{\Lambda ^{3}}_{3}\\\end{bmatrix}}{\begin{bmatrix}x^{0}\\x^{1}\\x^{2}\\x^{3}\end{bmatrix}}$$ allows the transformation of other physical quantities that cannot be expressed as four-vectors; e.g.,tensorsorspinorsof any order in 4d spacetime, to be defined. In the correspondingtensor index notation,the above matrix expression is $${x'}^{\nu }={\Lambda ^{\nu }}_{\mu }x^{\mu },$$

where lower and upper indices labelcovariant and contravariant componentsrespectively,and thesummation conventionis applied. It is a standard convention to useGreekindices that take the value 0 for time components, and 1, 2, 3 for space components, whileLatinindices simply take the values 1, 2, 3, for spatial components (the opposite for Landau and Lifshitz). Note that the first index (reading left to right) corresponds in the matrix notation to arow index.The second index corresponds to the column index.

The transformation matrix is universal for allfour-vectors,not just 4-dimensional spacetime coordinates. IfAis any four-vector, then intensor index notation$${A'}^{\nu }={\Lambda ^{\nu }}_{\mu }A^{\mu }\,.$$

Alternatively, one writes$$A^{\nu '}={\Lambda ^{\nu '}}_{\mu }A^{\mu }\,.$$in which the primed indices denote the indices of A in the primed frame. For a generaln-component object one may write$${X'}^{\alpha }={\Pi (\Lambda )^{\alpha }}_{\beta }X^{\beta }\,,$$whereΠis the appropriaterepresentation of the Lorentz group,ann×nmatrix for everyΛ.In this case, the indices shouldnotbe thought of as spacetime indices (sometimes called Lorentz indices), and they run from1ton.E.g., ifXis abispinor,then the indices are calledDirac indices.

Covariant vectors

There are also vector quantities with covariant indices. They are generally obtained from their corresponding objects with contravariant indices by the operation oflowering an index;e.g., $$x_{\nu }=\eta _{\mu \nu }x^{\mu },$$ whereηis themetric tensor.(The linked article also provides more information about what the operation of raising and lowering indices really is mathematically.) The inverse of this transformation is given by $$x^{\mu }=\eta ^{\mu \nu }x_{\nu },$$ where, when viewed as matrices,η^μνis the inverse ofη_μν.As it happens,η^μν=η_μν.This is referred to asraising an index.To transform a covariant vectorA_μ,first raise its index, then transform it according to the same rule as for contravariant4-vectors, then finally lower the index; $${A'}_{\nu }=\eta _{\rho \nu }{\Lambda ^{\rho }}_{\sigma }\eta ^{\mu \sigma }A_{\mu }.$$

But$$\eta _{\rho \nu }{\Lambda ^{\rho }}_{\sigma }\eta ^{\mu \sigma }={\left(\Lambda ^{-1}\right)^{\mu }}_{\nu },$$

That is, it is the(μ,ν)-component of theinverseLorentz transformation. One defines (as a matter of notation), $${\Lambda _{\nu }}^{\mu }\equiv {\left(\Lambda ^{-1}\right)^{\mu }}_{\nu },$$ and may in this notation write $${A'}_{\nu }={\Lambda _{\nu }}^{\mu }A_{\mu }.$$

Now for a subtlety. The implied summation on the right hand side of $${A'}_{\nu }={\Lambda _{\nu }}^{\mu }A_{\mu }={\left(\Lambda ^{-1}\right)^{\mu }}_{\nu }A_{\mu }$$ is running overa row indexof the matrix representingΛ⁻¹.Thus, in terms of matrices, this transformation should be thought of as theinverse transposeofΛacting on the column vectorA_μ.That is, in pure matrix notation, $$A'=\left(\Lambda ^{-1}\right)^{\mathrm {T} }A.$$

This means exactly that covariant vectors (thought of as column matrices) transform according to thedual representationof the standard representation of the Lorentz group. This notion generalizes to general representations, simply replaceΛwithΠ(Λ).

Tensors

IfAandBare linear operators on vector spacesUandV,then a linear operatorA⊗Bmay be defined on thetensor productofUandV,denotedU⊗Vaccording to

$(A\otimes B)(u\otimes v)=Au\otimes Bv,\qquad u\in U,v\in V,u\otimes v\in U\otimes V.$(T1)

From this it is immediately clear that ifuandvare a four-vectors inV,thenu⊗v∈T₂V≡V⊗Vtransforms as

$u\otimes v\rightarrow \Lambda u\otimes \Lambda v={\Lambda ^{\mu }}_{\nu }u^{\nu }\otimes {\Lambda ^{\rho }}_{\sigma }v^{\sigma }={\Lambda ^{\mu }}_{\nu }{\Lambda ^{\rho }}_{\sigma }u^{\nu }\otimes v^{\sigma }\equiv {\Lambda ^{\mu }}_{\nu }{\Lambda ^{\rho }}_{\sigma }w^{\nu \sigma }.$(T2)

The second step uses the bilinearity of the tensor product and the last step defines a 2-tensor on component form, or rather, it just renames the tensoru⊗v.

These observations generalize in an obvious way to more factors, and using the fact that a general tensor on a vector spaceVcan be written as a sum of a coefficient (component!) times tensor products of basis vectors and basis covectors, one arrives at the transformation law for anytensorquantityT.It is given by

$T_{\theta '\iota '\cdots \kappa '}^{\alpha '\beta '\cdots \zeta '}={\Lambda ^{\alpha '}}_{\mu }{\Lambda ^{\beta '}}_{\nu }\cdots {\Lambda ^{\zeta '}}_{\rho }{\Lambda _{\theta '}}^{\sigma }{\Lambda _{\iota '}}^{\upsilon }\cdots {\Lambda _{\kappa '}}^{\zeta }T_{\sigma \upsilon \cdots \zeta }^{\mu \nu \cdots \rho },$(T3)

whereΛ_χ′^ψis defined above. This form can generally be reduced to the form for generaln-component objects given above with a single matrix (Π(Λ)) operating on column vectors. This latter form is sometimes preferred; e.g., for the electromagnetic field tensor.

Transformation of the electromagnetic field

Lorentz boost of an electric charge, the charge is at rest in one frame or the other.

Main article:Electromagnetic tensor

Further information:classical electromagnetism and special relativity

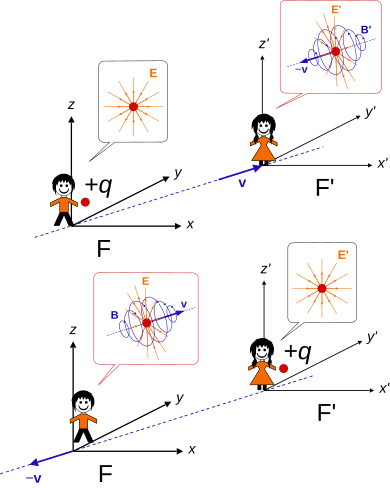
Lorentz transformations can also be used to illustrate that themagnetic fieldBandelectric fieldEare simply different aspects of the same force — theelectromagnetic force,as a consequence of relative motion betweenelectric chargesand observers.The fact that the electromagnetic field shows relativistic effects becomes clear by carrying out a simple thought experiment.

• An observer measures a charge at rest in frame F. The observer will detect a static electric field. As the charge is stationary in this frame, there is no electric current, so the observer does not observe any magnetic field.
• The other observer in frame F′ moves at velocityvrelative to F and the charge.Thisobserver sees a different electric field because the charge moves at velocity−vin their rest frame. The motion of the charge corresponds to anelectric current,and thus the observer in frame F′ also sees a magnetic field.

The electric and magnetic fields transform differently from space and time, but exactly the same way as relativistic angular momentum and the boost vector.

The electromagnetic field strength tensor is given by $$F^{\mu \nu }={\begin{bmatrix}0&-{\frac {1}{c}}E_{x}&-{\frac {1}{c}}E_{y}&-{\frac {1}{c}}E_{z}\\{\frac {1}{c}}E_{x}&0&-B_{z}&B_{y}\\{\frac {1}{c}}E_{y}&B_{z}&0&-B_{x}\\{\frac {1}{c}}E_{z}&-B_{y}&B_{x}&0\end{bmatrix}}{\text{(SI units, signature }}(+,-,-,-){\text{)}}.$$ inSI units.In relativity, theGaussian system of unitsis often preferred over SI units, even in texts whose main choice of units is SI units, because in it the electric fieldEand the magnetic inductionBhave the same units making the appearance of theelectromagnetic field tensormore natural.Consider a Lorentz boost in thex-direction. It is given by $${\Lambda ^{\mu }}_{\nu }={\begin{bmatrix}\gamma &-\gamma \beta &0&0\\-\gamma \beta &\gamma &0&0\\0&0&1&0\\0&0&0&1\\\end{bmatrix}},\qquad F^{\mu \nu }={\begin{bmatrix}0&E_{x}&E_{y}&E_{z}\\-E_{x}&0&B_{z}&-B_{y}\\-E_{y}&-B_{z}&0&B_{x}\\-E_{z}&B_{y}&-B_{x}&0\end{bmatrix}}{\text{(Gaussian units, signature }}(-,+,+,+){\text{)}},$$ where the field tensor is displayed side by side for easiest possible reference in the manipulations below.

The general transformation law(T3)becomes $$F^{\mu '\nu '}={\Lambda ^{\mu '}}_{\mu }{\Lambda ^{\nu '}}_{\nu }F^{\mu \nu }.$$

For the magnetic field one obtains $${\begin{aligned}B_{x'}&=F^{2'3'}={\Lambda ^{2}}_{\mu }{\Lambda ^{3}}_{\nu }F^{\mu \nu }={\Lambda ^{2}}_{2}{\Lambda ^{3}}_{3}F^{23}=1\times 1\times B_{x}\\&=B_{x},\\B_{y'}&=F^{3'1'}={\Lambda ^{3}}_{\mu }{\Lambda ^{1}}_{\nu }F^{\mu \nu }={\Lambda ^{3}}_{3}{\Lambda ^{1}}_{\nu }F^{3\nu }={\Lambda ^{3}}_{3}{\Lambda ^{1}}_{0}F^{30}+{\Lambda ^{3}}_{3}{\Lambda ^{1}}_{1}F^{31}\\&=1\times (-\beta \gamma )(-E_{z})+1\times \gamma B_{y}=\gamma B_{y}+\beta \gamma E_{z}\\&=\gamma \left(\mathbf {B} -{\boldsymbol {\beta }}\times \mathbf {E} \right)_{y}\\B_{z'}&=F^{1'2'}={\Lambda ^{1}}_{\mu }{\Lambda ^{2}}_{\nu }F^{\mu \nu }={\Lambda ^{1}}_{\mu }{\Lambda ^{2}}_{2}F^{\mu 2}={\Lambda ^{1}}_{0}{\Lambda ^{2}}_{2}F^{02}+{\Lambda ^{1}}_{1}{\Lambda ^{2}}_{2}F^{12}\\&=(-\gamma \beta )\times 1\times E_{y}+\gamma \times 1\times B_{z}=\gamma B_{z}-\beta \gamma E_{y}\\&=\gamma \left(\mathbf {B} -{\boldsymbol {\beta }}\times \mathbf {E} \right)_{z}\end{aligned}}$$

For the electric field results $${\begin{aligned}E_{x'}&=F^{0'1'}={\Lambda ^{0}}_{\mu }{\Lambda ^{1}}_{\nu }F^{\mu \nu }={\Lambda ^{0}}_{1}{\Lambda ^{1}}_{0}F^{10}+{\Lambda ^{0}}_{0}{\Lambda ^{1}}_{1}F^{01}\\&=(-\gamma \beta )(-\gamma \beta )(-E_{x})+\gamma \gamma E_{x}=-\gamma ^{2}\beta ^{2}(E_{x})+\gamma ^{2}E_{x}=E_{x}(1-\beta ^{2})\gamma ^{2}\\&=E_{x},\\E_{y'}&=F^{0'2'}={\Lambda ^{0}}_{\mu }{\Lambda ^{2}}_{\nu }F^{\mu \nu }={\Lambda ^{0}}_{\mu }{\Lambda ^{2}}_{2}F^{\mu 2}={\Lambda ^{0}}_{0}{\Lambda ^{2}}_{2}F^{02}+{\Lambda ^{0}}_{1}{\Lambda ^{2}}_{2}F^{12}\\&=\gamma \times 1\times E_{y}+(-\beta \gamma )\times 1\times B_{z}=\gamma E_{y}-\beta \gamma B_{z}\\&=\gamma \left(\mathbf {E} +{\boldsymbol {\beta }}\times \mathbf {B} \right)_{y}\\E_{z'}&=F^{0'3'}={\Lambda ^{0}}_{\mu }{\Lambda ^{3}}_{\nu }F^{\mu \nu }={\Lambda ^{0}}_{\mu }{\Lambda ^{3}}_{3}F^{\mu 3}={\Lambda ^{0}}_{0}{\Lambda ^{3}}_{3}F^{03}+{\Lambda ^{0}}_{1}{\Lambda ^{3}}_{3}F^{13}\\&=\gamma \times 1\times E_{z}-\beta \gamma \times 1\times (-B_{y})=\gamma E_{z}+\beta \gamma B_{y}\\&=\gamma \left(\mathbf {E} +{\boldsymbol {\beta }}\times \mathbf {B} \right)_{z}.\end{aligned}}$$

Here,β= (β,0, 0)is used. These results can be summarized by $${\begin{aligned}\mathbf {E} _{\parallel '}&=\mathbf {E} _{\parallel }\\\mathbf {B} _{\parallel '}&=\mathbf {B} _{\parallel }\\\mathbf {E} _{\bot '}&=\gamma \left(\mathbf {E} _{\bot }+{\boldsymbol {\beta }}\times \mathbf {B} _{\bot }\right)=\gamma \left(\mathbf {E} +{\boldsymbol {\beta }}\times \mathbf {B} \right)_{\bot },\\\mathbf {B} _{\bot '}&=\gamma \left(\mathbf {B} _{\bot }-{\boldsymbol {\beta }}\times \mathbf {E} _{\bot }\right)=\gamma \left(\mathbf {B} -{\boldsymbol {\beta }}\times \mathbf {E} \right)_{\bot },\end{aligned}}$$ and are independent of the metric signature. For SI units, substituteE→E⁄c.Misner, Thorne & Wheeler (1973)refer to this last form as the3 + 1view as opposed to thegeometric viewrepresented by the tensor expression $$F^{\mu '\nu '}={\Lambda ^{\mu '}}_{\mu }{\Lambda ^{\nu '}}_{\nu }F^{\mu \nu },$$ and make a strong point of the ease with which results that are difficult to achieve using the3 + 1view can be obtained and understood. Only objects that have well defined Lorentz transformation properties (in fact underanysmooth coordinate transformation) are geometric objects. In the geometric view, the electromagnetic field is a six-dimensional geometric object inspacetimeas opposed to two interdependent, but separate, 3-vector fields inspaceandtime.The fieldsE(alone) andB(alone) do not have well defined Lorentz transformation properties. The mathematical underpinnings are equations(T1)and(T2)that immediately yield(T3).One should note that the primed and unprimed tensors refer to thesame event in spacetime.Thus the complete equation with spacetime dependence is $$F^{\mu '\nu '}\left(x'\right)={\Lambda ^{\mu '}}_{\mu }{\Lambda ^{\nu '}}_{\nu }F^{\mu \nu }\left(\Lambda ^{-1}x'\right)={\Lambda ^{\mu '}}_{\mu }{\Lambda ^{\nu '}}_{\nu }F^{\mu \nu }(x).$$

Length contraction has an effect oncharge densityρandcurrent densityJ,and time dilation has an effect on the rate of flow of charge (current), so charge and current distributions must transform in a related way under a boost. It turns out they transform exactly like the space-time and energy-momentum four-vectors, $${\begin{aligned}\mathbf {j} '&=\mathbf {j} -\gamma \rho v\mathbf {n} +\left(\gamma -1\right)(\mathbf {j} \cdot \mathbf {n} )\mathbf {n} \\\rho '&=\gamma \left(\rho -\mathbf {j} \cdot {\frac {v\mathbf {n} }{c^{2}}}\right),\end{aligned}}$$

or, in the simpler geometric view, $$j^{\mu '}={\Lambda ^{\mu '}}_{\mu }j^{\mu }.$$

Charge density transforms as the time component of a four-vector. It is a rotational scalar. The current density is a 3-vector.

TheMaxwell equationsare invariant under Lorentz transformations.

Spinors

Equation(T1)hold unmodified for any representation of the Lorentz group, including thebispinorrepresentation. In(T2)one simply replaces all occurrences ofΛby the bispinor representationΠ(Λ),

${\begin{aligned}u\otimes v\rightarrow \Pi (\Lambda )u\otimes \Pi (\Lambda )v&={\Pi (\Lambda )^{\alpha }}_{\beta }u^{\beta }\otimes {\Pi (\Lambda )^{\rho }}_{\sigma }v^{\sigma }\\&={\Pi (\Lambda )^{\alpha }}_{\beta }{\Pi (\Lambda )^{\rho }}_{\sigma }u^{\beta }\otimes v^{\sigma }\\&\equiv {\Pi (\Lambda )^{\alpha }}_{\beta }{\Pi (\Lambda )^{\rho }}_{\sigma }w^{\beta \sigma }\end{aligned}}$(T4)

The above equation could, for instance, be the transformation of a state inFock spacedescribing two free electrons.

Transformation of general fields

A generalnoninteractingmulti-particle state (Fock space state) inquantum field theorytransforms according to the rule

$${\begin{aligned}&U(\Lambda,a)\Psi _{p_{1}\sigma _{1}n_{1};p_{2}\sigma _{2}n_{2};\cdots }\\={}&e^{-ia_{\mu }\left[(\Lambda p_{1})^{\mu }+(\Lambda p_{2})^{\mu }+\cdots \right]}{\sqrt {\frac {(\Lambda p_{1})^{0}(\Lambda p_{2})^{0}\cdots }{p_{1}^{0}p_{2}^{0}\cdots }}}\left(\sum _{\sigma _{1}'\sigma _{2}'\cdots }D_{\sigma _{1}'\sigma _{1}}^{(j_{1})}\left[W(\Lambda,p_{1})\right]D_{\sigma _{2}'\sigma _{2}}^{(j_{2})}\left[W(\Lambda,p_{2})\right]\cdots \right)\Psi _{\Lambda p_{1}\sigma _{1}'n_{1};\Lambda p_{2}\sigma _{2}'n_{2};\cdots },\end{aligned}}$$ (1)

whereW(Λ,p)is theWigner's little groupandD^(j)is the(2j+ 1)-dimensionalrepresentation ofSO(3).

See also

• Ricci calculus
• Electromagnetic field
• Galilean transformation
• Hyperbolic rotation
• Lorentz group
• Representation theory of the Lorentz group
• Principle of relativity
• Velocity-addition formula
• Algebra of physical space
• Relativistic aberration
• Prandtl–Glauert transformation
• Split-complex number
• Gyrovector space

References

Websites

• O'Connor, John J.; Robertson, Edmund F. (1996),A History of Special Relativity
• Brown, Harvey R. (2003),Michelson, FitzGerald and Lorentz: the Origins of Relativity Revisited

Papers

• Cushing, J. T. (1967)."Vector Lorentz transformations".American Journal of Physics.35(9): 858–862.Bibcode:1967AmJPh..35..858C.doi:10.1119/1.1974267.
• Macfarlane, A. J. (1962). "On the Restricted Lorentz Group and Groups Homomorphically Related to It".Journal of Mathematical Physics.3(6): 1116–1129.Bibcode:1962JMP.....3.1116M.doi:10.1063/1.1703854.hdl:2027/mdp.39015095220474.
• Rothman, Tony (2006),"Lost in Einstein's Shadow"(PDF),American Scientist,94(2): 112f
• Darrigol, Olivier (2005),"The Genesis of the theory of relativity"(PDF),Séminaire Poincaré,1:1–22,Bibcode:2006eins.book....1D,doi:10.1007/3-7643-7436-5_1,ISBN978-3-7643-7435-8
• Macrossan, Michael N. (1986),"A Note on Relativity Before Einstein",Br. J. Philos. Sci.,37(2): 232–34,CiteSeerX10.1.1.679.5898,doi:10.1093/bjps/37.2.232,archived fromthe originalon 2013-10-29,retrieved2007-04-02
• Poincaré, Henri(1905),"On the Dynamics of the Electron",Comptes Rendus Hebdomadaires des Séances de l'Académie des Sciences,140:1504–1508
• Einstein, Albert (1905),"Zur Elektrodynamik bewegter Körper"(PDF),Annalen der Physik,322(10): 891–921,Bibcode:1905AnP...322..891E,doi:10.1002/andp.19053221004.See also:English translation.
• Lorentz, Hendrik Antoon (1904)."Electromagnetic phenomena in a system moving with any velocity smaller than that of light".Proceedings of the Royal Netherlands Academy of Arts and Sciences.6:809–831.
• Einstein, A.(1916).Relativity: The Special and General Theory.Retrieved2012-01-23.Einstein, A.(1916).Relativity: The Special and General Theory.New York: Three Rivers Press (published 1995).ISBN978-0-517-88441-6– via Albert Einstein Reference Archive.
• Ungar, A. A. (1988). "Thomas rotation and the parameterization of the Lorentz transformation group".Foundations of Physics Letters.1(1): 55–89.Bibcode:1988FoPhL...1...57U.doi:10.1007/BF00661317.ISSN0894-9875.S2CID121240925.eqn (55).
• Ungar, A. A. (1989). "The relativistic velocity composition paradox and the Thomas rotation".Foundations of Physics.19(11): 1385–1396.Bibcode:1989FoPh...19.1385U.doi:10.1007/BF00732759.S2CID55561589.
• Ungar, A. A. (2000). "The relativistic composite-velocity reciprocity principle".Foundations of Physics.30(2): 331–342.CiteSeerX10.1.1.35.1131.doi:10.1023/A:1003653302643.S2CID118634052.
• Mocanu, C. I. (1986). "Some difficulties within the framework of relativistic electrodynamics".Archiv für Elektrotechnik.69(2): 97–110.doi:10.1007/bf01574845.S2CID123543303.
• Mocanu, C. I. (1992). "On the relativistic velocity composition paradox and the Thomas rotation".Foundations of Physics.5(5): 443–456.Bibcode:1992FoPhL...5..443M.doi:10.1007/bf00690425.S2CID122472788.
• Weinberg, S.(2002).The Quantum Theory of Fields, vol I.Cambridge University Press.ISBN978-0-521-55001-7.

Books

• Barut, Asim Orhan (1964).Electrodynamics and Classical Theory of Fields and Particles.Macmillan.ISBN978-0-486-64038-9.
• Dennery, Philippe; Krzywicki, André (2012).Mathematics for Physicists.Courier Corporation.ISBN978-0-486-15712-2.
• Cottingham, W. N.; Greenwood, D. A. (2007).An Introduction to the Standard Model of Particle Physics(2nd ed.). Cambridge University Press.ISBN978-1-139-46221-1.
• Young, H. D.; Freedman, R. A. (2008).University Physics – With Modern Physics(12th ed.). Pearson Addison-Wesley.ISBN978-0-321-50130-1.
• Halpern, A. (1988).3000 Solved Problems in Physics.Schaum Series. Mc Graw Hill. p. 688.ISBN978-0-07-025734-4.
• Forshaw, J. R.; Smith, A. G. (2009).Dynamics and Relativity.Manchester Physics Series. John Wiley & Sons Ltd. pp. 124–126.ISBN978-0-470-01460-8.
• Wheeler, J. A.;Taylor, E. F(1971).Spacetime Physics.Freeman.ISBN978-0-7167-0336-5.
• Wheeler, J. A.;Thorne, K. S.;Misner, C. W.(1973).Gravitation.Freeman.ISBN978-0-7167-0344-0.
• Carroll, S. M.(2004).Spacetime and Geometry: An Introduction to General Relativity(illustrated ed.). Addison Wesley. p. 22.ISBN978-0-8053-8732-2.
• Grant, I. S.; Phillips, W. R. (2008). "14".Electromagnetism.Manchester Physics (2nd ed.). John Wiley & Sons.ISBN978-0-471-92712-9.
• Griffiths, D. J.(2007).Introduction to Electrodynamics(3rd ed.). Pearson Education, Dorling Kindersley.ISBN978-81-7758-293-2.
• Hall, Brian C. (2003).Lie Groups, Lie Algebras, and Representations An Elementary Introduction.Springer.ISBN978-0-387-40122-5.
• Weinberg, Steven (1972).Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity.Wiley.ISBN978-0-471-92567-5.
• Weinberg, S.(2008),Cosmology,Wiley,ISBN978-0-19-852682-7
• Weinberg, S.(2005),The quantum theory of fields (3 vol.),vol. 1, Cambridge University Press,ISBN978-0-521-67053-1
• Ohlsson, T.(2011),Relativistic Quantum Physics,Cambridge University Press,ISBN978-0-521-76726-2
• Goldstein, H.(1980) [1950].Classical Mechanics(2nd ed.). Reading MA:Addison-Wesley.ISBN978-0-201-02918-5.
• Jackson, J. D.(1975) [1962]."Chapter 11".Classical Electrodynamics(2nd ed.).John Wiley & Sons.pp.542–545.ISBN978-0-471-43132-9.
• Landau, L. D.;Lifshitz, E. M.(2002) [1939].The Classical Theory of Fields.Course of Theoretical Physics.Vol. 2 (4th ed.).Butterworth–Heinemann.pp. 9–12.ISBN0-7506-2768-9.
• Feynman, R. P.;Leighton, R. B.;Sands, M.(1977) [1963]. "15".The Feynman Lectures on Physics.Vol. 1. Addison Wesley.ISBN978-0-201-02117-2.
• Feynman, R. P.;Leighton, R. B.;Sands, M.(1977) [1964]. "13".The Feynman Lectures on Physics.Vol. 2. Addison Wesley.ISBN978-0-201-02117-2.
• Misner, Charles W.;Thorne, Kip S.;Wheeler, John Archibald(1973).Gravitation.San Francisco:W. H. Freeman.ISBN978-0-7167-0344-0.
• Rindler, W.(2006) [2001]. "Chapter 9".Relativity Special, General and Cosmological(2nd ed.). Dallas:Oxford University Press.ISBN978-0-19-856732-5.
• Ryder, L. H.(1996) [1985].Quantum Field Theory(2nd ed.). Cambridge:Cambridge University Press.ISBN978-0521478144.
• Sard, R. D. (1970).Relativistic Mechanics - Special Relativity and Classical Particle Dynamics.New York: W. A. Benjamin.ISBN978-0805384918.
• Sexl, R. U.; Urbantke, H. K. (2001) [1992].Relativity, Groups Particles. Special Relativity and Relativistic Symmetry in Field and Particle Physics.Springer.ISBN978-3211834435.
• Gourgoulhon, Eric (2013).Special Relativity in General Frames: From Particles to Astrophysics.Springer. p. 213.ISBN978-3-642-37276-6.
• Chaichian, Masud; Hagedorn, Rolf (1997).Symmetry in quantum mechanics:From angular momentum to supersymmetry.IoP. p. 239.ISBN978-0-7503-0408-5.
• Landau, L.D.;Lifshitz, E.M.(2002) [1939].The Classical Theory of Fields.Course of Theoretical Physics. Vol. 2 (4th ed.).Butterworth–Heinemann.ISBN0-7506-2768-9.

Further reading

• Ernst, A.; Hsu, J.-P. (2001),"First proposal of the universal speed of light by Voigt 1887"(PDF),Chinese Journal of Physics,39(3): 211–230,Bibcode:2001ChJPh..39..211E,archived fromthe original(PDF)on 2011-07-16
• Thornton, Stephen T.; Marion, Jerry B. (2004),Classical dynamics of particles and systems(5th ed.), Belmont, [CA.]: Brooks/Cole, pp. 546–579,ISBN978-0-534-40896-1
• Voigt, Woldemar(1887), "Über das Doppler'sche princip",Nachrichten von der Königlicher Gesellschaft den Wissenschaft zu Göttingen,2:41–51

External links

• Derivation of the Lorentz transformations.This web page contains a more detailed derivation of the Lorentz transformation with special emphasis on group properties.
• The Paradox of Special Relativity.This webpage poses a problem, the solution of which is the Lorentz transformation, which is presented graphically in its next page.
• RelativityArchived2011-08-29 at theWayback Machine– a chapter from an online textbook
• Warp Special Relativity Simulator.A computer program demonstrating the Lorentz transformations on everyday objects.
• Animation cliponYouTubevisualizing the Lorentz transformation.
• MinutePhysics videoonYouTubeexplaining and visualizing the Lorentz transformation with a mechanical Minkowski diagram
• Interactive graphonDesmos (graphing)showing Lorentz transformations with a virtual Minkowski diagram
• Interactive graphon Desmos showing Lorentz transformations with points and hyperbolas
• Lorentz Frames Animatedfrom John de Pillis.Online Flash animations of Galilean and Lorentz frames, various paradoxes, EM wave phenomena,etc.