Phonon

Not to be confused withphoton,Phonon (software),orPhonon (company).

Aphononis acollective excitationin a periodic,elasticarrangement ofatomsormoleculesincondensed matter,specifically insolidsand someliquids.A type ofquasiparticleinphysics,a phonon is anexcited statein thequantum mechanicalquantizationof themodes of vibrationsfor elastic structures of interacting particles. Phonons can be thought of as quantizedsound waves,similar tophotonsas quantizedlight waves.

The study of phonons is an important part of condensed matter physics. They play a major role in many of the physical properties of condensed matter systems, such asthermal conductivityandelectrical conductivity,as well as in models ofneutron scatteringand related effects.

The concept of phonons was introduced in 1932 bySovietphysicistIgor Tamm.The namephononcomes from theGreekwordφωνή(phonē), which translates tosoundorvoice,because long-wavelength phonons give rise tosound.The name emphasizes the analogy to the wordphoton,in that phonons representwave-particle dualityforsound wavesin the same way that photons represent wave-particle duality forlight waves.Solids with more than one atom in the smallestunit cellexhibit bothacousticandopticalphonons.

Definition

A phonon is thequantum mechanicaldescription of an elementaryvibrationalmotion in which alatticeof atoms or molecules uniformly oscillates at a singlefrequency.Inclassical mechanicsthis designates anormal modeof vibration. Normal modes are important because any arbitrary lattice vibration can be considered to be asuperpositionof theseelementaryvibration modes (cf.Fourier analysis). While normal modes arewave-likephenomena in classical mechanics, phonons haveparticle-likeproperties too, in a way related to thewave–particle dualityof quantum mechanics.

Lattice dynamics

The equations in this section do not useaxiomsof quantum mechanics but instead use relations for which there exists a directcorrespondencein classical mechanics.

For example: a rigid regular,crystalline(notamorphous) lattice is composed ofNparticles. These particles may be atoms or molecules.Nis a large number, say of the order of 10²³,or on the order of theAvogadro numberfor a typical sample of a solid. Since the lattice is rigid, the atoms must be exertingforceson one another to keep each atom near its equilibrium position. These forces may beVan der Waals forces,covalent bonds,electrostatic attractions,and others, all of which are ultimately due to theelectricforce.Magneticandgravitationalforces are generally negligible. The forces between each pair of atoms may be characterized by apotential energyfunctionVthat depends on the distance of separation of the atoms. The potential energy of the entire lattice is the sum of all pairwise potential energies multiplied by a factor of 1/2 to compensate for double counting:

${\frac {1}{2}}\sum _{i\neq j}V\left(r_{i}-r_{j}\right)$

wherer_iis thepositionof theith atom, andVis the potential energy between two atoms.

It is difficult to solve thismany-body problemexplicitly in either classical or quantum mechanics. In order to simplify the task, two importantapproximationsare usually imposed. First, the sum is only performed over neighboring atoms. Although the electric forces in real solids extend to infinity, this approximation is still valid because the fields produced by distant atoms are effectivelyscreened.Secondly, the potentialsVare treated asharmonic potentials.This is permissible as long as the atoms remain close to their equilibrium positions. Formally, this is accomplished byTaylor expandingVabout its equilibrium value to quadratic order, givingVproportional to the displacementx²and the elastic force simply proportional tox.The error in ignoring higher order terms remains small ifxremains close to the equilibrium position.

The resulting lattice may be visualized as a system of balls connected by springs. The following figure shows a cubic lattice, which is a good model for many types of crystalline solid. Other lattices include a linear chain, which is a very simple lattice which we will shortly use for modeling phonons. (For other common lattices, seecrystal structure.)

The potential energy of the lattice may now be written as

$\sum _{\{ij\}(\mathrm {nn} )}{\tfrac {1}{2}}m\omega ^{2}\left(R_{i}-R_{j}\right)^{2}.$

Here,ωis thenatural frequencyof the harmonic potentials, which are assumed to be the same since the lattice is regular.R_iis the position coordinate of theith atom, which we now measure from its equilibrium position. The sum over nearest neighbors is denoted (nn).

It is important to mention that the mathematical treatment given here is highly simplified in order to make it accessible to non-experts. The simplification has been achieved by making two basic assumptions in the expression for the total potential energy of the crystal. These assumptions are that (i) the total potential energy can be written as a sum of pairwise interactions, and (ii) each atom interacts with only its nearest neighbors. These are used only sparingly in modern lattice dynamics.A more general approach is to express the potential energy in terms of force constants.See, for example, the Wiki article onmultiscale Green's functions.

Lattice waves

Phonon propagating through a square lattice (atom displacements greatly exaggerated)

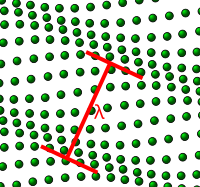
Due to the connections between atoms, the displacement of one or more atoms from their equilibrium positions gives rise to a set of vibrationwavespropagating through the lattice. One such wave is shown in the figure to the right. Theamplitudeof the wave is given by the displacements of the atoms from their equilibrium positions. Thewavelengthλis marked.

There is a minimum possible wavelength, given by twice the equilibrium separationabetween atoms. Any wavelength shorter than this can be mapped onto a wavelength longer than 2a,due to the periodicity of the lattice. This can be thought as one consequence ofNyquist–Shannon sampling theorem,the lattice points being viewed as the "sampling points" of a continuous wave.

Not every possible lattice vibration has a well-defined wavelength and frequency. However, thenormal modesdo possess well-defined wavelengths andfrequencies.

One-dimensional lattice

Animation showing 6 normal modes of a one-dimensional lattice: a linear chain of particles. The shortest wavelength is at top, with progressively longer wavelengths below. In the lowest lines the motion of the waves to the right can be seen.

In order to simplify the analysis needed for a 3-dimensional lattice of atoms, it is convenient to model a 1-dimensional lattice or linear chain. This model is complex enough to display the salient features of phonons.

Classical treatment

The forces between the atoms are assumed to be linear and nearest-neighbour, and they are represented by an elastic spring. Each atom is assumed to be a point particle and the nucleus and electrons move in step (adiabatic theorem):

n− 1nn+ 1←a→

···o++++++o++++++o++++++o++++++o++++++o++++++o++++++o++++++o++++++o···

→→→→→→

u_{n− 1}u_nu_{n+ 1}

wherenlabels thenth atom out of a total ofN,ais the distance between atoms when the chain is in equilibrium, andu_nthe displacement of thenth atom from its equilibrium position.

IfCis the elastic constant of the spring andmthe mass of the atom, then the equation of motion of thenth atom is

$-2Cu_{n}+C\left(u_{n+1}+u_{n-1}\right)=m{\frac {d^{2}u_{n}}{dt^{2}}}.$

This is a set of coupled equations.

Since the solutions are expected to be oscillatory, new coordinates are defined by adiscrete Fourier transform,in order to decouple them.

Put

$u_{n}=\sum _{Nak/2\pi =1}^{N}Q_{k}e^{ikna}.$

Here,nacorresponds and devolves to the continuous variablexof scalar field theory. TheQ_kare known as thenormal coordinates,continuum field modesφ_k.

Substitution into the equation of motion produces the followingdecoupled equations(this requires a significant manipulation using the orthonormality and completeness relations of the discrete Fourier transform),

$2C(\cos {ka-1})Q_{k}=m{\frac {d^{2}Q_{k}}{dt^{2}}}.$

These are the equations for decoupledharmonic oscillatorswhich have the solution

$Q_{k}=A_{k}e^{i\omega _{k}t};\qquad \omega _{k}={\sqrt {{\frac {2C}{m}}(1-\cos {ka})}}.$

Each normal coordinateQ_krepresents an independent vibrational mode of the lattice with wavenumberk,which is known as anormal mode.

The second equation, forω_k,is known as thedispersion relationbetween theangular frequencyand thewavenumber.

In thecontinuum limit,a→0,N→∞, withNaheld fixed,u_n→φ(x),a scalar field, and$\omega (k)\propto ka$.This amounts to classical freescalar field theory,an assembly of independent oscillators.

Quantum treatment

A one-dimensional quantum mechanical harmonic chain consists ofNidentical atoms. This is the simplest quantum mechanical model of a lattice that allows phonons to arise from it. The formalism for this model is readily generalizable to two and three dimensions.

In some contrast to the previous section, the positions of the masses are not denoted byu_i,but, instead, byx₁,x₂..., as measured from their equilibrium positions (i.e.x_i= 0 if particleiis at its equilibrium position.) In two or more dimensions, thex_iare vector quantities. TheHamiltonianfor this system is

${\mathcal {H}}=\sum _{i=1}^{N}{\frac {p_{i}^{2}}{2m}}+{\frac {1}{2}}m\omega ^{2}\sum _{\{ij\}(\mathrm {nn} )}\left(x_{i}-x_{j}\right)^{2}$

wheremis the mass of each atom (assuming it is equal for all), andx_iandp_iare the position andmomentumoperators, respectively, for theith atom and the sum is made over the nearest neighbors (nn). However one expects that in a lattice there could also appear waves that behave like particles. It is customary to deal withwavesinFourier spacewhich usesnormal modesof thewavevectoras variables instead coordinates of particles. The number of normal modes is same as the number of particles. Still, the Fourier space is very useful given theperiodicityof the system.

A set ofN"normal coordinates"Q_kmay be introduced, defined as thediscrete Fourier transformsof thex_kandN"conjugate momenta"Π_kdefined as the Fourier transforms of thep_k:

${\begin{aligned}Q_{k}&={\frac {1}{\sqrt {N}}}\sum _{l}e^{ikal}x_{l}\\\Pi _{k}&={\frac {1}{\sqrt {N}}}\sum _{l}e^{-ikal}p_{l}.\end{aligned}}$

The quantityk_nturns out to be thewavenumberof the phonon, i.e. 2πdivided by thewavelength.

This choice retains the desired commutation relations in either real space or wavevector space

${\begin{aligned}\left[x_{l},p_{m}\right]&=i\hbar \delta _{l,m}\\\left[Q_{k},\Pi _{k'}\right]&={\frac {1}{N}}\sum _{l,m}e^{ikal}e^{-ik'am}\left[x_{l},p_{m}\right]\\&={\frac {i\hbar }{N}}\sum _{l}e^{ial\left(k-k'\right)}=i\hbar \delta _{k,k'}\\\left[Q_{k},Q_{k'}\right]&=\left[\Pi _{k},\Pi _{k'}\right]=0\end{aligned}}$

From the general result

${\begin{aligned}\sum _{l}x_{l}x_{l+m}&={\frac {1}{N}}\sum _{kk'}Q_{k}Q_{k'}\sum _{l}e^{ial\left(k+k'\right)}e^{iamk'}=\sum _{k}Q_{k}Q_{-k}e^{iamk}\\\sum _{l}{p_{l}}^{2}&=\sum _{k}\Pi _{k}\Pi _{-k}\end{aligned}}$

The potential energy term is

${\tfrac {1}{2}}m\omega ^{2}\sum _{j}\left(x_{j}-x_{j+1}\right)^{2}={\tfrac {1}{2}}m\omega ^{2}\sum _{k}Q_{k}Q_{-k}(2-e^{ika}-e^{-ika})={\tfrac {1}{2}}\sum _{k}m{\omega _{k}}^{2}Q_{k}Q_{-k}$

where

$\omega _{k}={\sqrt {2\omega ^{2}\left(1-\cos {ka}\right)}}=2\omega \left|\sin {\frac {ka}{2}}\right|$

The Hamiltonian may be written in wavevector space as

${\mathcal {H}}={\frac {1}{2m}}\sum _{k}\left(\Pi _{k}\Pi _{-k}+m^{2}\omega _{k}^{2}Q_{k}Q_{-k}\right)$

The couplings between the position variables have been transformed away; if theQandΠwereHermitian(which they are not), the transformed Hamiltonian would describeNuncoupled harmonic oscillators.

The form of the quantization depends on the choice of boundary conditions; for simplicity,periodicboundary conditions are imposed, defining the (N+ 1)th atom as equivalent to the first atom. Physically, this corresponds to joining the chain at its ends. The resulting quantization is

$k=k_{n}={\frac {2\pi n}{Na}}\quad {\mbox{for }}n=0,\pm 1,\pm 2,\ldots \pm {\frac {N}{2}}.\$

The upper bound toncomes from the minimum wavelength, which is twice the lattice spacinga,as discussed above.

The harmonic oscillator eigenvalues or energy levels for the modeω_kare:

$E_{n}=\left({\tfrac {1}{2}}+n\right)\hbar \omega _{k}\qquad n=0,1,2,3\ldots$

The levels are evenly spaced at:

${\tfrac {1}{2}}\hbar \omega,\ {\tfrac {3}{2}}\hbar \omega,\ {\tfrac {5}{2}}\hbar \omega \ \cdots$

where⁠1/2⁠ħωis thezero-point energyof aquantum harmonic oscillator.

Anexactamount ofenergyħωmust be supplied to the harmonic oscillator lattice to push it to the next energy level. By analogy to thephotoncase when theelectromagnetic fieldis quantized, the quantum of vibrational energy is called a phonon.

All quantum systems show wavelike and particlelike properties simultaneously. The particle-like properties of the phonon are best understood using the methods ofsecond quantizationand operator techniques described later.

See also:Canonical quantization § Real scalar field

Three-dimensional lattice

This may be generalized to a three-dimensional lattice. The wavenumberkis replaced by a three-dimensionalwavevectork.Furthermore, eachkis now associated with three normal coordinates.

The new indicess= 1, 2, 3 label thepolarizationof the phonons. In the one-dimensional model, the atoms were restricted to moving along the line, so the phonons corresponded tolongitudinal waves.In three dimensions, vibration is not restricted to the direction of propagation, and can also occur in the perpendicular planes, liketransverse waves.This gives rise to the additional normal coordinates, which, as the form of the Hamiltonian indicates, we may view as independent species of phonons.

Dispersion relation

Dispersion curves in linear diatomic chain

Optical and acoustic vibrations in a linear diatomic chain.

Vibrations of the diatomic chain at different frequencies.

Dispersion relationω=ω(k) for some waves corresponding to lattice vibrations in GaAs.

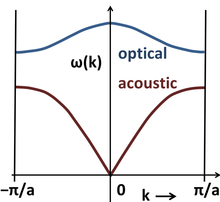
For a one-dimensional alternating array of two types of ion or atom of massm₁,m₂repeated periodically at a distancea,connected by springs of spring constantK,two modes of vibration result:

$\omega _{\pm }^{2}=K\left({\frac {1}{m_{1}}}+{\frac {1}{m_{2}}}\right)\pm K{\sqrt {\left({\frac {1}{m_{1}}}+{\frac {1}{m_{2}}}\right)^{2}-{\frac {4\sin ^{2}{\frac {ka}{2}}}{m_{1}m_{2}}}}},$

wherekis the wavevector of the vibration related to its wavelength by $k={\tfrac {2\pi }{\lambda }}$.

The connection between frequency and wavevector,ω=ω(k), is known as adispersion relation.The plus sign results in the so-calledopticalmode, and the minus sign to theacousticmode. In the optical mode two adjacent different atoms move against each other, while in the acoustic mode they move together.

The speed of propagation of an acoustic phonon, which is also thespeed of soundin the lattice, is given by the slope of the acoustic dispersion relation,⁠∂ω_k/∂k⁠(seegroup velocity.) At low values ofk(i.e. long wavelengths), the dispersion relation is almost linear, and the speed of sound is approximatelyωa,independent of the phonon frequency. As a result, packets of phonons with different (but long) wavelengths can propagate for large distances across the lattice without breaking apart. This is the reason that sound propagates through solids without significant distortion. This behavior fails at large values ofk,i.e. short wavelengths, due to the microscopic details of the lattice.

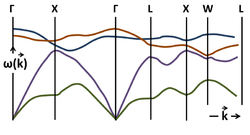
For a crystal that has at least two atoms in itsprimitive cell,the dispersion relations exhibit two types of phonons, namely, optical and acoustic modes corresponding to the upper blue and lower red curve in the diagram, respectively. The vertical axis is the energy or frequency of phonon, while the horizontal axis is thewavevector.The boundaries at −⁠π/a⁠and⁠π/a⁠are those of the firstBrillouin zone.A crystal withN≥ 2 different atoms in theprimitive cellexhibits three acoustic modes: onelongitudinal acoustic modeand twotransverse acoustic modes.The number of optical modes is 3N– 3. The lower figure shows the dispersion relations for several phonon modes inGaAsas a function of wavevectorkin theprincipal directionsof its Brillouin zone.

The modes are also referred to as the branches of phonon dispersion. In general, if there are p atoms (denoted by N earlier) in the primitive unit cell, there will be 3p branches of phonon dispersion in a 3-dimensional crystal. Out of these, 3 branches correspond to acoustic modes and the remaining 3p-3 branches will correspond to optical modes. In some special directions, some branches coincide due to symmetry. These branches are called degenerate. In acoustic modes, all the p atoms vibrate in phase. So there is no change in the relative displacements of these atoms during the wave propagation.

Study of phonon dispersion is useful for modeling propagation of sound waves in solids, which is characterized by phonons. The energy of each phonon, as given earlier, isħω.The velocity of the wave also is given in terms ofωand k.The direction of the wave vector is the direction of the wave propagation and the phonon polarization vector gives the direction in which the atoms vibrate. Actually, in general, the wave velocity in a crystal is different for different directions of k. In other words, most crystals are anisotropic for phonon propagation.

A wave is longitudinal if the atoms vibrate in the same direction as the wave propagation. In a transverse wave, the atoms vibrate perpendicular to the wave propagation. However, except for isotropic crystals, waves in a crystal are not exactly longitudinal or transverse. For general anisotropic crystals, the phonon waves are longitudinal or transverse only in certain special symmetry directions. In other directions, they can be nearly longitudinal or nearly transverse. It is only for labeling convenience, that they are often called longitudinal or transverse but are actually quasi-longitudinal or quasi-transverse. Note that in the three-dimensional case, there are two directions perpendicular to a straight line at each point on the line. Hence, there are always two (quasi) transverse waves for each (quasi) longitudinal wave.

Many phonon dispersion curves have been measured byinelastic neutron scattering.

The physics of sound influidsdiffers from the physics of sound in solids, although both are density waves: sound waves in fluids only have longitudinal components, whereas sound waves in solids have longitudinal and transverse components. This is because fluids cannot supportshear stresses(but seeviscoelasticfluids, which only apply to high frequencies).

Interpretation of phonons using second quantization techniques

The above-derived Hamiltonian may look like a classical Hamiltonian function, but if it is interpreted as anoperator,then it describes aquantum field theoryof non-interactingbosons. Thesecond quantizationtechnique, similar to theladder operatormethod used forquantum harmonic oscillators,is a means of extracting energyeigenvalueswithout directly solving the differential equations. Given the Hamiltonian,${\mathcal {H}}$,as well as the conjugate position,$Q_{k}$,and conjugate momentum$\Pi _{k}$defined in the quantum treatment section above, we can definecreation and annihilation operators:

$b_{k}={\sqrt {\frac {m\omega _{k}}{2\hbar }}}\left(Q_{k}+{\frac {i}{m\omega _{k}}}\Pi _{-k}\right)$and${b_{k}}^{\dagger }={\sqrt {\frac {m\omega _{k}}{2\hbar }}}\left(Q_{-k}-{\frac {i}{m\omega _{k}}}\Pi _{k}\right)$

The following commutators can be easily obtained by substituting in thecanonical commutation relation:

$\left[b_{k},{b_{k'}}^{\dagger }\right]=\delta _{k,k'},\quad {\Big [}b_{k},b_{k'}{\Big ]}=\left[{b_{k}}^{\dagger },{b_{k'}}^{\dagger }\right]=0$

Using this, the operatorsb_k^†andb_kcan be inverted to redefine the conjugate position and momentum as:

$Q_{k}={\sqrt {\frac {\hbar }{2m\omega _{k}}}}\left({b_{k}}^{\dagger }+b_{-k}\right)$and$\Pi _{k}=i{\sqrt {\frac {\hbar m\omega _{k}}{2}}}\left({b_{k}}^{\dagger }-b_{-k}\right)$

Directly substituting these definitions for$Q_{k}$and$\Pi _{k}$into the wavevector space Hamiltonian, as it is defined above, and simplifying then results in the Hamiltonian taking the form:

${\mathcal {H}}=\sum _{k}\hbar \omega _{k}\left({b_{k}}^{\dagger }b_{k}+{\tfrac {1}{2}}\right)$

This is known as the second quantization technique, also known as the occupation number formulation, wheren_k=b_k^†b_kis the occupation number. This can be seen to be a sum of N independent oscillator Hamiltonians, each with a unique wave vector, and compatible with the methods used for the quantum harmonic oscillator (note thatn_kishermitian).When a Hamiltonian can be written as a sum of commuting sub-Hamiltonians, the energy eigenstates will be given by the products of eigenstates of each of the separate sub-Hamiltonians. The correspondingenergyspectrumis then given by the sum of the individual eigenvalues of the sub-Hamiltonians.

As with the quantum harmonic oscillator, one can show thatb_k^†andb_krespectively create and destroy a single field excitation, a phonon, with an energy ofħω_k.

Three important properties of phonons may be deduced from this technique. First, phonons arebosons,since any number of identical excitations can be created by repeated application of the creation operatorb_k^†.Second, each phonon is a "collective mode" caused by the motion of every atom in the lattice. This may be seen from the fact that the creation and annihilation operators, defined here in momentum space, contains sums over the position and momentum operators of every atom when written in position space (Seeposition and momentum space).Finally, using theposition–positioncorrelation function,it can be shown that phonons act as waves of lattice displacement.

This technique is readily generalized to three dimensions, where the Hamiltonian takes the form:

${\mathcal {H}}=\sum _{k}\sum _{s=1}^{3}\hbar \,\omega _{k,s}\left({b_{k,s}}^{\dagger }b_{k,s}+{\tfrac {1}{2}}\right).$

Which can be interpreted as the sum of 3N independent oscillator Hamiltonians, one for each wave vector and polarization.

Acoustic and optical phonons

Solids with more than one atom in the smallestunit cellexhibit two types of phonons: acoustic phonons and optical phonons.

Acoustic phononsare coherent movements of atoms of the lattice out of their equilibrium positions. If the displacement is in the direction of propagation, then in some areas the atoms will be closer, in others farther apart, as in a sound wave in air (hence the name acoustic). Displacement perpendicular to the propagation direction is comparable to waves on a string. If the wavelength of acoustic phonons goes to infinity, this corresponds to a simple displacement of the whole crystal, and this costs zero deformation energy. Acoustic phonons exhibit a linear relationship between frequency and phonon wave-vector for long wavelengths. The frequencies of acoustic phonons tend to zero with longer wavelength. Longitudinal and transverse acoustic phonons are often abbreviated as LA and TA phonons, respectively.

Optical phononsare out-of-phase movements of the atoms in the lattice, one atom moving to the left, and its neighbor to the right. This occurs if the lattice basis consists of two or more atoms. They are calledopticalbecause in ionic crystals, such assodium chloride,fluctuations in displacement create an electrical polarization that couples to the electromagnetic field.Hence, they can be excited byinfrared radiation,the electric field of the light will move every positive sodium ion in the direction of the field, and every negative chloride ion in the other direction, causing the crystal to vibrate.

Optical phonons have a non-zero frequency at theBrillouin zonecenter and show no dispersion near that long wavelength limit. This is because they correspond to a mode of vibration where positive and negative ions at adjacent lattice sites swing against each other, creating a time-varyingelectrical dipole moment.Optical phonons that interact in this way with light are calledinfrared active.Optical phonons that areRaman activecan also interact indirectly with light, throughRaman scattering.Optical phonons are often abbreviated as LO and TO phonons, for the longitudinal and transverse modes respectively; the splitting between LO and TO frequencies is often described accurately by theLyddane–Sachs–Teller relation.

When measuring optical phonon energy experimentally, optical phonon frequencies are sometimes given in spectroscopicwavenumbernotation, where the symbolωrepresents ordinary frequency (not angular frequency), and is expressed in units ofcm⁻¹.The value is obtained by dividing the frequency by thespeed of light in vacuum.In other words, the wave-number in cm⁻¹units corresponds to the inverse of thewavelengthof aphotonin vacuum that has the same frequency as the measured phonon.

Crystal momentum

Main article:Crystal momentum

k-vectors exceeding the firstBrillouin zone(red) do not carry any more information than their counterparts (black) in the first Brillouin zone.

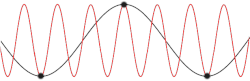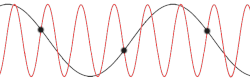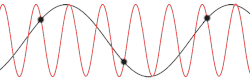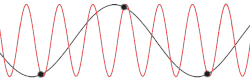
By analogy tophotonsandmatter waves,phonons have been treated withwavevectorkas though it has amomentumħk;however, this is not strictly correct, becauseħkis not actually a physical momentum; it is called thecrystal momentumorpseudomomentum.This is becausekis only determined up to addition of constant vectors (thereciprocal lattice vectorsand integer multiples thereof). For example, in the one-dimensional model, the normal coordinatesQandΠare defined so that

$Q_{k}{\stackrel {\mathrm {def} }{=}}Q_{k+K};\quad \Pi _{k}{\stackrel {\mathrm {def} }{=}}\Pi _{k+K}$

where

$K={\frac {2n\pi }{a}}$

for any integern.A phonon with wavenumberkis thus equivalent to an infinite family of phonons with wavenumbersk±⁠2π/a⁠,k±⁠4π/a⁠,and so forth. Physically, the reciprocal lattice vectors act as additional chunks of momentum which the lattice can impart to the phonon.Bloch electronsobey a similar set of restrictions.

Brillouin zones, (a) in a square lattice, and (b) in a hexagonal lattice

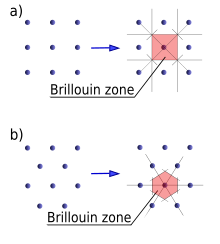
It is usually convenient to consider phonon wavevectorskwhich have the smallest magnitude |k| in their "family". The set of all such wavevectors defines thefirstBrillouin zone.Additional Brillouin zones may be defined as copies of the first zone, shifted by some reciprocal lattice vector.

Thermodynamics

Thethermodynamicproperties of a solid are directly related to its phonon structure. The entire set of all possible phonons that are described by the phonon dispersion relations combine in what is known as the phonondensity of stateswhich determines theheat capacityof a crystal. By the nature of this distribution, the heat capacity is dominated by the high-frequency part of the distribution, while thermal conductivity is primarily the result of the low-frequency region.

Atabsolute zerotemperature, a crystal lattice lies in itsground state,and contains no phonons. A lattice at a nonzerotemperaturehas an energy that is not constant, but fluctuatesrandomlyabout somemean value.These energy fluctuations are caused by random lattice vibrations, which can be viewed as a gas of phonons. Because these phonons are generated by the temperature of the lattice, they are sometimes designated thermal phonons.

Thermal phonons can be created and destroyed by random energy fluctuations. In the language of statistical mechanics this means that the chemical potential for adding a phonon is zero.This behavior is an extension of the harmonic potential into the anharmonic regime. The behavior of thermal phonons is similar to thephoton gasproduced by anelectromagnetic cavity,wherein photons may be emitted or absorbed by the cavity walls. This similarity is not coincidental, for it turns out that the electromagnetic field behaves like a set of harmonic oscillators, giving rise toblack-body radiation.Both gases obey theBose–Einstein statistics:in thermal equilibrium and within the harmonic regime, the probability of finding phonons or photons in a given state with a given angular frequency is:

$n\left(\omega _{k,s}\right)={\frac {1}{\exp \left({\dfrac {\hbar \omega _{k,s}}{k_{\mathrm {B} }T}}\right)-1}}$

whereω_k,sis the frequency of the phonons (or photons) in the state,k_Bis theBoltzmann constant,andTis the temperature.

Phonon tunneling

Phonons have been shown to exhibitquantum tunnelingbehavior (orphonon tunneling) where, across gaps up to a nanometer wide, heat can flow via phonons that "tunnel" between two materials.This type of heat transfer works between distances too large forconductionto occur but too small forradiationto occur and therefore cannot be explained by classicalheat transfermodels.

Operator formalism

The phonon Hamiltonian is given by

${\mathcal {H}}={\tfrac {1}{2}}\sum _{\alpha }\left(p_{\alpha }^{2}+\omega _{\alpha }^{2}q_{\alpha }^{2}-\hbar \omega _{\alpha }\right)$

In terms of thecreation and annihilation operators,these are given by

${\mathcal {H}}=\sum _{\alpha }\hbar \omega _{\alpha }{a_{\alpha }}^{\dagger }a_{\alpha }$

Here, in expressing theHamiltonianin operator formalism, we have not taken into account the⁠1/2⁠ħω_qterm as, given acontinuumorinfinite lattice,the⁠1/2⁠ħω_qterms will add up yielding aninfinite term.Because the difference in energy is what we measure and not the absolute value of it, the constant term⁠1/2⁠ħω_qcan be ignored without changing the equations of motion. Hence, the⁠1/2⁠ħω_qfactor is absent in the operator formalized expression for theHamiltonian.

The ground state, also called the "vacuum state",is the state composed of no phonons. Hence, the energy of the ground state is 0. When a system is in the state|n₁n₂n₃…⟩,we say there aren_αphonons of typeα,wheren_αis the occupation number of the phonons. The energy of a single phonon of typeαis given byħω_qand the total energy of a general phonon system is given byn₁ħω₁+n₂ħω₂+.... As there are no cross terms (e.g.n₁ħω₂), the phonons are said to be non-interacting. The action of the creation and annihilation operators is given by:

${a_{\alpha }}^{\dagger }{\Big |}n_{1}\ldots n_{\alpha -1}n_{\alpha }n_{\alpha +1}\ldots {\Big \rangle }={\sqrt {n_{\alpha }+1}}{\Big |}n_{1}\ldots,n_{\alpha -1},(n_{\alpha }+1),n_{\alpha +1}\ldots {\Big \rangle }$

and,

$a_{\alpha }{\Big |}n_{1}\ldots n_{\alpha -1}n_{\alpha }n_{\alpha +1}\ldots {\Big \rangle }={\sqrt {n_{\alpha }}}{\Big |}n_{1}\ldots,n_{\alpha -1},(n_{\alpha }-1),n_{\alpha +1},\ldots {\Big \rangle }$

The creation operator,a_α^†creates a phonon of typeαwhilea_αannihilates one. Hence, they are respectively the creation and annihilation operators for phonons. Analogous to thequantum harmonic oscillatorcase, we can defineparticle number operatoras

$N=\sum _{\alpha }{a_{\alpha }}^{\dagger }a_{\alpha }.$

The number operator commutes with a string of products of the creation and annihilation operators if and only if the number of creation operators is equal to number of annihilation operators.

It can be shown that phonons are symmetric under exchange (i.e.|α,β⟩=|β,α⟩), so therefore they are consideredbosons.

Nonlinearity

As well asphotons,phonons can interact viaparametric down conversionand formsqueezed coherent states.

Predicted properties

Recent research has shown that phonons androtonsmay have a non-negligible mass and be affected by gravity just as standard particles are.In particular, phonons are predicted to have a kind ofnegative massand negative gravity.This can be explained by how phonons are known to travel faster in denser materials. Because the part of a material pointing towards a gravitational source is closer to the object, it becomes denser on that end. From this, it is predicted that phonons would deflect away as it detects the difference in densities, exhibiting the qualities of a negative gravitational field.Although the effect would be too small to measure, it is possible that future equipment could lead to successful results.

Superconductivity

Superconductivityis a state of electronic matter in whichelectrical resistancevanishes andmagnetic fieldsare expelled from the material. In a superconductor, electrons are bound together intoCooper pairsby a weak attractive force. In a conventional superconductor, this attraction is caused by anexchangeof phonons between the electrons.The evidence that phonons, the vibrations of the ionic lattice, are relevant for superconductivity is provided by theisotope effect,the dependence of the superconducting critical temperature on the mass of the ions.

Other research

In 2019, researchers were able to isolate individual phonons without destroying them for the first time.

They have been also shown to form “phonon winds” where an electric current in a graphene surface is generated by a liquid flow above it due to the viscous forces at the liquid–solid interface.

See also

• Boson
• Brillouin scattering
• Fracton
• Linear elasticity
• Mechanical wave
• Phonon scattering
• Carrier scattering
• Phononic crystal
• Rayleigh wave
• Relativistic heat conduction
• Rigid unit modes
• SASER
• Second sound
• Surface acoustic wave
• Surface phonon
• Thermal conductivity
• Vibration

External links

• Quotations related toPhononat Wikiquote
• Explained: Phonons,MIT News, 2010.
• Optical and acoustic modes
• Phonons in a One Dimensional Microfluidic Crystal[1]and[2]with movies in[3].