Superellipse

Examples of superellipses for$a=1,\ b=0.75$

Asuperellipse,also known as aLamé curveafterGabriel Lamé,is a closed curve resembling theellipse,retaining the geometric features ofsemi-major axisandsemi-minor axis,and symmetry about them, but defined by an equation that allows for various shapes between a rectangle and an ellipse.

In two dimensionalCartesian coordinate system,a superellipse is defined as the set of all points$(x,y)$on the curve that satisfy the equation$$\left|{\frac {x}{a}}\right|^{n}\!\!+\left|{\frac {y}{b}}\right|^{n}\!=1,$$where$a$and$b$are positive numbers referred to assemi-diametersorsemi-axesof the superellipse, and$n$is a positive parameter that defines the shape. When$n=2$,the superellipse is an ordinary ellipse. For$n>2$,the shape is more rectangular with rounded corners, and for$0<n<2$,it is more pointed.

In thepolar coordinate system,the superellipse equation is (the set of all points$(r,\theta )$on the curve satisfy the equation):$$r=\left(\left|{\frac {\cos(\theta )}{a}}\right|^{n}\!\!+\left|{\frac {\sin(\theta )}{b}}\right|^{n}\!\right)^{-1/n}\!.$$

Specific cases

This formula defines aclosed curvecontained in therectangle−a≤x≤ +aand−b≤y≤ +b.The parameters$a$and$b$are the semi-diameters or semi-axes of the curve. The overall shape of the curve is determined by the value of the exponent$n$,as shown in the following table:

$0<n<1$	The superellipse looks like a four-armed star withconcave(inwards-curved) sides. For$n=1/2$,in particular, each of the four arcs is a segment of aparabola. Anastroidis the special case$a=b$,$n=2/3$	The superellipse withn=1⁄2,a=b= 1
$n=1$	The curve is arhombuswith corners ($\pm a,0$) and ($0,\pm b$).
$1<n<2$	The curve looks like a rhombus with the same corners but withconvex(outwards-curved) sides. Thecurvatureincreases withoutlimitas one approaches its extreme points.	The superellipse withn=3⁄2,a=b= 1
$n=2$	The curve is an ordinaryellipse(in particular, acircleif$a=b$).
$n>2$	The curve looks superficially like arectanglewith rounded corners. The curvature is zero at the points ($\pm a,0$) and ($0,\pm b$).	Squircle,the superellipse withn= 4,a=b= 1

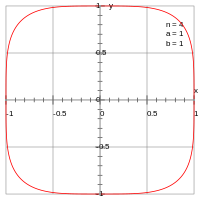If$n<2$,the figure is also called ahypoellipse;if$n>2$,ahyperellipse.When$n\geq 1$and$a=b$,the superellipse is the boundary of aballof$\mathbb {R} ^{2}$in the$n$-norm.The extreme points of the superellipse are ($\pm a,0$) and ($0,\pm b$), and its four "corners" are ($\pm s_{a}$,$\pm s_{b}$), where$s=2^{-1/n}$(sometimes called the "superness").

Mathematical properties

Whennis a positiverational number$p/q$(in lowest terms), then each quadrant of the superellipse is aplane algebraic curveof order$p/q$.In particular, when$a=b=1$andnis an even integer, then it is aFermat curveof degreen.In that case it is non-singular, but in general it will besingular.If the numerator is not even, then the curve is pieced together from portions of the same algebraic curve in different orientations.

The curve is given by theparametric equations(with parameter$t$having no elementary geometric interpretation)$$\left.{\begin{aligned}x\left(t\right)&=\pm a\cos ^{\frac {2}{n}}t\\y\left(t\right)&=\pm b\sin ^{\frac {2}{n}}t\end{aligned}}\right\}\qquad 0\leq t\leq {\frac {\pi }{2}}$$where each$\pm$can be chosen separately so that each value of$t$gives four points on the curve. Equivalently, letting$t$range over$0\leq t<2\pi,$$${\begin{aligned}x\left(t\right)&={\left|\cos t\right|}^{\frac {2}{n}}\cdot a\operatorname {sgn}(\cos t)\\y\left(t\right)&={\left|\sin t\right|}^{\frac {2}{n}}\cdot b\operatorname {sgn}(\sin t)\end{aligned}}$$where thesign functionis$$\operatorname {sgn}(w)={\begin{cases}-1,&w<0\\0,&w=0\\+1,&w>0.\end{cases}}$$Here$t$is not the angle between the positive horizontal axis and the ray from the origin to the point, since the tangent of this angle equals$y/x$while in the parametric expressions${\textstyle {\frac {y}{x}}={\frac {b}{a}}(\tan t)^{2/n}\neq \tan t.}$

Area

Theareainside the superellipse can be expressed in terms of thegamma functionas$$\mathrm {Area} =4ab{\frac {\left(\Gamma \left(1+{\tfrac {1}{n}}\right)\right)^{2}}{\Gamma \left(1+{\tfrac {2}{n}}\right)}},$$or in terms of thebeta functionas

$\mathrm {Area} ={\frac {4ab}{n}}\mathrm {B} \!\left({\frac {1}{n}},{\frac {1}{n}}+1\right).$

Perimeter

Theperimeterof a superellipse, like that of anellipse,does not admitclosed-form solutionpurely usingelementary functions.Exact solutions for the perimeter of a superellipse exist usinginfinite summations;these could be truncated to obtain approximate solutions.Numerical integrationis another option to obtain perimeter estimates at arbitrary precision.

A closed-form approximation obtained viasymbolic regressionis also an option that balances parsimony and accuracy. Consider a superellipse centered on the origin of a 2D plane. Now, imagine that the superellipse (with shape parameter$n$) is stretched such that the first quadrant (e.g.,$x>0$,$y>0$) is an arc from$(1,0)$to$(0,h)$,with$h\geq 1$.Then, the arc length of the superellipse within that single quadrant is approximated as the following function of$h$and$n$:

h + (((((n-0.88487077) * h + 0.2588574 / h) ^ exp(n / -0.90069205)) + h) + 0.09919785) ^ (-1.4812293 / n)

This single-quadrant arc length approximation is accurate to within ±0.2% for across all values of$n$,and can be used to efficiently estimate the total perimeter of a superellipse.

Pedal curve

Thepedal curveis relatively straightforward to compute. Specifically, the pedal of$$\left|{\frac {x}{a}}\right|^{n}\!+\left|{\frac {y}{b}}\right|^{n}\!=1,$$is given inpolar coordinatesby$$(a\cos \theta )^{\tfrac {n}{n-1}}+(b\sin \theta )^{\tfrac {n}{n-1}}=r^{\tfrac {n}{n-1}}.$$

Generalizations

The generalization of these shapes can involve several approaches.The generalizations of the superellipse in higher dimensions retain the fundamental mathematical structure of the superellipse while adapting it to different contexts and applications.

Higher dimensions

The generalizations of the superellipse in higher dimensions retain the fundamental mathematical structure of the superellipse while adapting it to different contexts and applications.

• Asuperellipsoidextends the superellipse into three dimensions, creating shapes that vary between ellipsoids and rectangular solids with rounded edges. The superellipsoid is defined as the set of all points$(x,y,z)$that satisfy the equation:$$\left|{\frac {x}{a}}\right|^{n}\!\!+\left|{\frac {y}{b}}\right|^{n}\!+\left|{\frac {z}{c}}\right|^{n}\!=1,$$where$a,b$and$c$are positive numbers referred to as the semi-axes of the superellipsoid, and$n$is a positive parameter that defines the shape.
• Ahyperellipsoidis the$d$-dimensional analogue of anellipsoid(and by extension, a superellipsoid). It is defined as the set of all points$(x_{1},x_{2},\ldots,x_{d})$that satisfy the equation:$$\left|{\frac {x_{1}}{a_{1}}}\right|^{n}\!\!+\left|{\frac {x_{2}}{a_{2}}}\right|^{n}\!+\ldots +\left|{\frac {x_{d}}{a_{d}}}\right|^{n}\!=1,$$where$a_{1},a_{2},\ldots,a_{d}$are positive numbers referred to as the semi-axes of the hyperellipsoid, and$n$is a positive parameter that defines the shape.

Different exponents

Variations of a superellipse with different exponents

Using different exponents for each term in the equation, allowing more flexibility in shape formation.

For two-dimentional case the equation is$\left|{\frac {x}{a}}\right|^{m}\!\!+\left|{\frac {y}{b}}\right|^{n}\!=1;m,n>0,$where$m$either equals to or differs from$n$.If$m=n$,it is the Lamé's superellipses. If$m\neq n$,the curve possesses more flexibility of behavior, and is better possible fit to describe some experimental information.

National Centre for the Performing Arts

For the three-dimensional case, three different positive powers$m$,$n$and$p$can be used in the equation$\left|{\frac {x}{a}}\right|^{m}\!\!+\left|{\frac {y}{b}}\right|^{n}\!+\left|{\frac {z}{c}}\right|^{p}\!=1$.If$m=n=p$,a super-ellipsoid is obtained. If any two or all three powers differ from each other, a solid is obtained that may possess more flexibility in representing real structural data than the super ellipsoid. A three-dimensional super-ellipsoid with$m=n=2.2$,$p=2.4$and the semi-diameters$a=b=1$,$c=0.5$represents the structure of the National Centre for the Performing Arts in China.

In the general$N$–dimensional case, the equation is$\left|{\frac {x_{1}}{a_{1}}}\right|^{N_{1}}\!\!+\left|{\frac {x_{2}}{a_{2}}}\right|^{N_{2}}\!+\ldots +\left|{\frac {x_{N}}{a_{N}}}\right|^{N_{N}}\!=1$,where In general,$n_{1},n_{2},\ldots,n_{N}$may differ from each other. It is the superellipsoid only if$n_{1}=n_{2}=\ldots =n_{N}=n$.

Related shapes

Superquadricsare a family of shapes that include superellipsoids as a special case. They are used in computer graphics and geometric modeling to create complex, smooth shapes with easily adjustable parameters.While not a direct generalization of superellipses,hyperspheresalso share the concept of extending geometric shapes into higher dimensions. These related shapes demonstrate the versatility and broad applicability of the fundamental principles underlying superellipses.

Anisotropic scaling

Anisotropicscaling involves scaling the shape differently along different axes, providing additional control over the geometry. This approach can be applied to superellipses, superellipsoids, and their higher-dimensional analogues to produce a wider variety of forms and better fit specific requirements in applications such as computer graphics, structural design, and data visualization. For instance, anisotropic scaling allows the creation of shapes that can model real-world objects more accurately by adjusting the proportions along each axis independently.

History

The general Cartesian notation of the form comes from the French mathematicianGabriel Lamé(1795–1870), who generalized the equation for the ellipse.

The outer outlines of the letters 'o' and 'O' in Zapf's Melior typeface are described by superellipses withn=log(1/2) / log (7/9)≈ 2.758

Hermann Zapf'stypefaceMelior,published in 1952, uses superellipses for letters such aso.Thirty years laterDonald Knuthwould build the ability to choose between true ellipses and superellipses (both approximated bycubic splines) into hisComputer Moderntype family.

The central fountain of Sergels Torg is outlined by a superellipse withn= 2.5 anda/b= 6/5.

The superellipse was named by theDanishpoet and scientistPiet Hein(1905–1996) though he did not discover it as it is sometimes claimed. In 1959, city planners inStockholm,Swedenannounced a design challenge for aroundaboutin their city squareSergels Torg.Piet Hein's winning proposal was based on a superellipse withn= 2.5 anda/b= 6/5.As he explained it:

Man is the animal that draws lines which he himself then stumbles over. In the whole pattern of civilization there have been two tendencies, one toward straight lines and rectangular patterns and one toward circular lines. There are reasons, mechanical and psychological, for both tendencies. Things made with straight lines fit well together and save space. And we can move easily — physically or mentally — around things made with round lines. But we are in a straitjacket, having to accept one or the other, when often some intermediate form would be better. To draw something freehand — such as the patchwork traffic circle they tried in Stockholm — will not do. It isn't fixed, isn't definite like a circle or square. You don't know what it is. It isn't esthetically satisfying. The super-ellipse solved the problem. It is neither round nor rectangular, but in between. Yet it is fixed, it is definite — it has a unity.

Sergels Torg was completed in 1967. Meanwhile, Piet Hein went on to use the superellipse in other artifacts, such as beds, dishes, tables, etc.By rotating a superellipse around the longest axis, he created thesuperegg,a solid egg-like shape that could stand upright on a flat surface, and was marketed as anovelty toy.

In 1968, when negotiators inParisfor theVietnam Warcould not agree on the shape of the negotiating table, Balinski,Kieron Underwoodand Holt suggested a superelliptical table in a letter to theNew York Times.The superellipse was used for the shape of the 1968Azteca Olympic Stadium,inMexico City.

The second floor of the originalWorld Trade Centerin New York City consisted of a large, superellipse-shaped overhanging balcony.

Waldo R. Toblerdeveloped amap projection,theTobler hyperelliptical projection,published in 1973,in which themeridiansare arcs of superellipses.

The logo for news companyThe Localconsists of a tilted superellipse matching the proportions of Sergels Torg. Three connected superellipses are used in the logo of thePittsburgh Steelers.

In computing, mobile operating systemiOSuses a superellipse curve for app icons, replacing therounded cornersstyle used up to version 6.

See also

• Astroid,the superellipse withn=2⁄3anda=b,is a hypocycloid with four cusps.
  • Deltoid curve,the hypocycloid ofthreecusps.
• Squircle,the superellipse withn= 4 anda=b,looks like "The Four-Cornered Wheel."
  • Reuleaux triangle,"The Three-Cornered Wheel."
• Superformula,a generalization of the superellipse.
• Superquadrics:superellipsoidsandsupertoroids,the three-dimensional "relatives" of superellipses.
• Superelliptic curve,equation of the formYⁿ=f(X).
• L^pspaces

External links

• Sokolov, D.D. (2001) [1994],"Lamé curve",Encyclopedia of Mathematics,EMS Press
• "Lamé Curve"at MathCurve.
• Weisstein, Eric W."Superellipse".MathWorld.
• O'Connor, John J.;Robertson, Edmund F.,"Lame Curves",MacTutor History of Mathematics Archive,University of St Andrews
• "Super Ellipse"on 2dcurves
• Superellipse Calculator & Template Generator
• Superellipse fitting toolbox in MATLAB
• C code for fitting superellipses