Pi

The number $π$ (/paɪ/;spelled out as "pi") is amathematical constantthat is theratioof acircle'scircumferenceto itsdiameter,approximately equal to 3.14159. The number $π$ appears in many formulae acrossmathematicsandphysics.It is anirrational number,meaning that it cannot be expressed exactly as a ratio of two integers, although fractions such as ${\tfrac {22}{7}}$ are commonlyused to approximate it.Consequently, itsdecimal representationnever ends, norenters a permanently repeating pattern.It is atranscendental number,meaning that it cannot be a solution of anequationinvolving only finite sums, products, powers, and integers. The transcendence of $π$ implies that it is impossible to solve the ancient challenge ofsquaring the circlewith acompass and straightedge.The decimal digits of $π$ appear to berandomly distributed,^[a]but no proof of thisconjecturehas been found.

For thousands of years, mathematicians have attempted to extend their understanding of $π$ ,sometimes by computing its value to a high degree of accuracy. Ancient civilizations, including theEgyptiansandBabylonians,required fairly accurate approximations of $π$ for practical computations. Around 250BC, theGreek mathematician Archimedescreated an algorithm to approximate $π$ with arbitrary accuracy. In the 5th century AD,Chinese mathematiciansapproximated $π$ to seven digits, whileIndian mathematiciansmade a five-digit approximation, both using geometrical techniques. The first computational formula for $π$ ,based oninfinite series,was discovered a millennium later.^[1]^[2]The earliest known use of the Greek letterπto represent the ratio of a circle's circumference to its diameter was by the Welsh mathematicianWilliam Jonesin 1706.^[3]

The invention ofcalculussoon led to the calculation of hundreds of digits of $π$ ,enough for all practical scientific computations. Nevertheless, in the 20th and 21st centuries, mathematicians andcomputer scientistshave pursued new approaches that, when combined with increasing computational power, extended the decimal representation of $π$ to many trillions of digits.^[4]^[5]These computations are motivated by the development of efficient algorithms to calculate numeric series, as well as the human quest to break records.^[6]^[7]The extensive computations involved have also been used to testsupercomputersas well as stress testing consumer computer hardware.

Because its definition relates to the circle, $π$ is found in many formulae intrigonometryandgeometry,especially those concerning circles, ellipses and spheres. It is also found in formulae from other topics in science, such ascosmology,fractals,thermodynamics,mechanics,andelectromagnetism.It also appears in areas having little to do with geometry, such asnumber theoryandstatistics,and in modernmathematical analysiscan be defined without any reference to geometry. The ubiquity of $π$ makes it one of the most widely known mathematical constants inside and outside of science. Several books devoted to $π$ have been published, and record-setting calculations of the digits of $π$ often result in news headlines.

Fundamentals

Name

The symbol used by mathematicians to represent the ratio of a circle's circumference to its diameter is the lowercaseGreek letter $π$ ,sometimes spelled out aspi.^[8]In English, $π$ ispronounced as "pie"(/paɪ/PY).^[9]In mathematical use, the lowercase letter $π$ is distinguished from its capitalized and enlarged counterpart $Π$ ,which denotes aproduct of a sequence,analogous to how $Σ$ denotessummation.

The choice of the symbol $π$ is discussed in the sectionAdoption of the symbol $π$ .

Definition

$π$ is commonly defined as theratioof acircle'scircumference $C$ to itsdiameter $d$ :^[10] $\pi ={\frac {C}{d}}$

The ratio ${\textstyle {\frac {C}{d}}}$ is constant, regardless of the circle's size. For example, if a circle has twice the diameter of another circle, it will also have twice the circumference, preserving the ratio ${\textstyle {\frac {C}{d}}}$ .This definition of $π$ implicitly makes use offlat (Euclidean) geometry;although the notion of a circle can be extended to anycurve (non-Euclidean) geometry,these new circles will no longer satisfy the formula ${\textstyle \pi ={\frac {C}{d}}}$ .^[10]

Here, the circumference of a circle is thearc lengtharound theperimeterof the circle, a quantity which can be formally defined independently of geometry usinglimits—a concept incalculus.^[11]For example, one may directly compute the arc length of the top half of the unit circle, given inCartesian coordinatesby the equation ${\textstyle x^{2}+y^{2}=1}$ ,as theintegral:^[12] $\pi =\int _{-1}^{1}{\frac {dx}{\sqrt {1-x^{2}}}}.$

An integral such as this was adopted as the definition of $π$ byKarl Weierstrass,who defined it directly as an integral in 1841.^[b]

Integration is no longer commonly used in a first analytical definition because, asRemmert 2012explains,differential calculustypically precedes integral calculus in the university curriculum, so it is desirable to have a definition of $π$ that does not rely on the latter. One such definition, due to Richard Baltzer^[13]and popularized byEdmund Landau,^[14]is the following: $π$ is twice the smallest positive number at which thecosinefunction equals 0.^[10]^[12]^[15] $π$ is also the smallest positive number at which thesinefunction equals zero, and the difference between consecutive zeroes of the sine function. The cosine and sine can be defined independently of geometry as apower series,^[16]or as the solution of adifferential equation.^[15]

In a similar spirit, $π$ can be defined using properties of thecomplex exponential, $exp z$ ,of acomplexvariable $z$ .Like the cosine, the complex exponential can be defined in one of several ways. The set of complex numbers at which $exp z$ is equal to one is then an (imaginary) arithmetic progression of the form: $\{\dots,-2\pi i,0,2\pi i,4\pi i,\dots \}=\{2\pi ki\mid k\in \mathbb {Z} \}$ and there is a unique positive real number $π$ with this property.^[12]^[17]

A variation on the same idea, making use of sophisticated mathematical concepts oftopologyandalgebra,is the following theorem:^[18]there is a unique (up to automorphism)continuous isomorphismfrom thegroupR/Zof real numbers under additionmodulointegers (thecircle group), onto the multiplicative group ofcomplex numbersofabsolute valueone. The number $π$ is then defined as half the magnitude of the derivative of this homomorphism.^[19]

Irrationality and normality

$π$ is anirrational number,meaning that it cannot be written as theratio of two integers.Fractions such as $.mw-parser-output .sfrac{white-space:nowrap}.mw-parser-output .sfrac.tion,.mw-parser-output .sfrac .tion{display:inline-block;vertical-align:-0.5em;font-size:85%;text-align:center}.mw-parser-output .sfrac .num{display:block;line-height:1em;margin:0.0em 0.1em;border-bottom:1px solid}.mw-parser-output .sfrac .den{display:block;line-height:1em;margin:0.1em 0.1em}.mw-parser-output .sr-only{border:0;clip:rect(0,0,0,0);clip-path:polygon(0px 0px,0px 0px,0px 0px);height:1px;margin:-1px;overflow:hidden;padding:0;position:absolute;width:1px}⁠22/7⁠$ and $⁠ 355 / 113 ⁠$ are commonly used to approximate $π$ ,but nocommon fraction(ratio of whole numbers) can be its exact value.^[20]Because $π$ is irrational, it has an infinite number of digits in itsdecimal representation,and does not settle into an infinitelyrepeating patternof digits. There are severalproofs that $π$ is irrational;they generally require calculus and rely on thereductio ad absurdumtechnique. The degree to which $π$ can be approximated byrational numbers(called theirrationality measure) is not precisely known; estimates have established that the irrationality measure is larger than the measure of $e$ or $ln 2$ but smaller than the measure ofLiouville numbers.^[21]

The digits of $π$ have no apparent pattern and have passed tests forstatistical randomness,including tests fornormality;a number of infinite length is called normal when all possible sequences of digits (of any given length) appear equally often. The conjecture that $π$ isnormalhas not been proven or disproven.^[22]

Since the advent of computers, a large number of digits of $π$ have been available on which to perform statistical analysis.Yasumasa Kanadahas performed detailed statistical analyses on the decimal digits of $π$ ,and found them consistent with normality; for example, the frequencies of the ten digits 0 to 9 were subjected tostatistical significance tests,and no evidence of a pattern was found.^[23]Any random sequence of digits contains arbitrarily long subsequences that appear non-random, by theinfinite monkey theorem.Thus, because the sequence of $π$ 's digits passes statistical tests for randomness, it contains some sequences of digits that may appear non-random, such as asequence of six consecutive 9sthat begins at the 762nd decimal place of the decimal representation of $π$ .^[24]This is also called the "Feynman point" inmathematical folklore,afterRichard Feynman,although no connection to Feynman is known.

Transcendence

In addition to being irrational, $π$ is also atranscendental number,which means that it is not thesolutionof any non-constantpolynomial equationwithrationalcoefficients, such as ${\textstyle {\frac {x^{5}}{120}}-{\frac {x^{3}}{6}}+x=0}$ .^[25]^[c]

The transcendence of $π$ has two important consequences: First, $π$ cannot be expressed using any finite combination of rational numbers and square roots orn-th roots(such as ${\sqrt[{3}]{31}}$ or ${\sqrt {10}}$ ). Second, since no transcendental number can beconstructedwithcompass and straightedge,it is not possible to "square the circle".In other words, it is impossible to construct, using compass and straightedge alone, a square whose area is exactly equal to the area of a given circle.^[26]Squaring a circle was one of the important geometry problems of theclassical antiquity.^[27]Amateur mathematicians in modern times have sometimes attempted to square the circle and claim success—despite the fact that it is mathematically impossible.^[28]^[29]

Continued fractions

As an irrational number, $π$ cannot be represented as acommon fraction.But every number, including $π$ ,can be represented by an infinite series of nested fractions, called acontinued fraction: $\pi =3+\textstyle {\cfrac {1}{7+\textstyle {\cfrac {1}{15+\textstyle {\cfrac {1}{1+\textstyle {\cfrac {1}{292+\textstyle {\cfrac {1}{1+\textstyle {\cfrac {1}{1+\textstyle {\cfrac {1}{1+\ddots }}}}}}}}}}}}}}$

Truncating the continued fraction at any point yields a rational approximation for $π$ ;the first four of these are $3$ , $⁠ 22 / 7 ⁠$ , $⁠ 333 / 106 ⁠$ ,and $⁠ 355 / 113 ⁠$ .These numbers are among the best-known and most widely used historical approximations of the constant. Each approximation generated in this way is a best rational approximation; that is, each is closer to $π$ than any other fraction with the same or a smaller denominator.^[30]Because $π$ is transcendental, it is by definition notalgebraicand so cannot be aquadratic irrational.Therefore, $π$ cannot have aperiodic continued fraction.Although the simple continued fraction for $π$ (shown above) also does not exhibit any other obvious pattern,^[31]^[32]severalgeneralized continued fractionsdo, such as:^[33] ${\begin{aligned}\pi &=3+{\cfrac {1^{2}}{6+{\cfrac {3^{2}}{6+{\cfrac {5^{2}}{6+{\cfrac {7^{2}}{6+\ddots }}}}}}}}={\cfrac {4}{1+{\cfrac {1^{2}}{2+{\cfrac {3^{2}}{2+{\cfrac {5^{2}}{2+\ddots }}}}}}}}={\cfrac {4}{1+{\cfrac {1^{2}}{3+{\cfrac {2^{2}}{5+{\cfrac {3^{2}}{7+\ddots }}}}}}}}\end{aligned}}$

The middle of these is due to the mid-17th century mathematicianWilliam Brouncker,see§ Brouncker's formula.

Approximate value and digits

Someapproximations ofpiinclude:

Integers:3
Fractions:Approximate fractions include (in order of increasing accuracy)⁠22/7⁠,⁠333/106⁠,⁠355/113⁠,⁠52163/16604⁠,⁠103993/33102⁠,⁠104348/33215⁠,and⁠245850922/78256779⁠.^[30](List is selected terms fromOEIS:A063674andOEIS:A063673.)
Digits:The first 50 decimal digits are3.14159265358979323846264338327950288419716939937510...^[34](seeOEIS:A000796)

Digits in other number systems

The first 48binary(base2) digits (calledbits) are11.001001000011111101101010100010001000010110100011...(seeOEIS:A004601)
The first 38 digits internary(base 3) are10.0102110122220102110021111102212222201...(seeOEIS:A004602)
The first 20 digits inhexadecimal(base 16) are3.243F6A8885A308D31319...^[35](seeOEIS:A062964)
The first fivesexagesimal(base 60) digits are 3;8,29,44,0,47^[36](seeOEIS:A060707)

Complex numbers and Euler's identity

Anycomplex number,say $z$ ,can be expressed using a pair ofreal numbers.In thepolar coordinate system,one number (radiusor $r$ ) is used to represent $z$ 's distance from theoriginof thecomplex plane,and the other (angle or $φ$ ) the counter-clockwiserotationfrom the positive real line:^[37] $z=r\cdot (\cos \varphi +i\sin \varphi ),$ where $i$ is theimaginary unitsatisfying $i^{2}=-1$ .The frequent appearance of $π$ incomplex analysiscan be related to the behaviour of theexponential functionof a complex variable, described byEuler's formula:^[38] $e^{i\varphi }=\cos \varphi +i\sin \varphi,$ wherethe constant $e$ is the base of thenatural logarithm.This formula establishes a correspondence between imaginary powers of $e$ and points on theunit circlecentred at the origin of the complex plane. Setting $\phi =\pi$ in Euler's formula results inEuler's identity,celebrated in mathematics due to it containing five important mathematical constants:^[38]^[39] $e^{i\pi }+1=0.$

There are $n$ differentcomplex numbers $z$ satisfying $z^{n}=1$ ,and these are called the " $n$ -throots of unity"^[40]and are given by the formula: $e^{2\pi ik/n}\qquad (k=0,1,2,\dots,n-1).$

History

Antiquity

The best-known approximations to $π$ datingbefore the Common Erawere accurate to two decimal places; this was improved upon inChinese mathematicsin particular by the mid-first millennium, to an accuracy of seven decimal places. After this, no further progress was made until the late medieval period.

The earliest written approximations of $π$ are found inBabylonand Egypt, both within one percent of the true value. In Babylon, aclay tabletdated 1900–1600 BC has a geometrical statement that, by implication, treats $π$ as⁠25/8⁠= 3.125.^[41]In Egypt, theRhind Papyrus,dated around 1650 BC but copied from a document dated to 1850 BC, has a formula for the area of a circle that treats $π$ as ${\textstyle {\bigl (}{\frac {16}{9}}{\bigr )}^{2}\approx 3.16}$ .^[32]^[41]Although somepyramidologistshave theorized that theGreat Pyramid of Gizawas built with proportions related to $π$ ,this theory is not widely accepted by scholars.^[42] In theShulba SutrasofIndian mathematics,dating to an oral tradition from the first or second millennium BC, approximations are given which have been variously interpreted as approximately 3.08831, 3.08833, 3.004, 3, or 3.125.^[43]

Polygon approximation era

The first recorded algorithm for rigorously calculating the value of $π$ was a geometrical approach using polygons, devised around 250 BC by the Greek mathematicianArchimedes,implementing themethod of exhaustion.^[44]This polygonal algorithm dominated for over 1,000 years, and as a result $π$ is sometimes referred to as Archimedes's constant.^[45]Archimedes computed upper and lower bounds of $π$ by drawing a regular hexagon inside and outside a circle, and successively doubling the number of sides until he reached a 96-sided regular polygon. By calculating the perimeters of these polygons, he proved that $⁠ 223 / 71 ⁠ < π < ⁠ 22 / 7 ⁠$ (that is, $3.1408 < π < 3.1429$ ).^[46]Archimedes' upper bound of $⁠ 22 / 7 ⁠$ may have led to a widespread popular belief that $π$ is equal to $⁠ 22 / 7 ⁠$ .^[47]Around 150 AD, Greek-Roman scientistPtolemy,in hisAlmagest,gave a value for $π$ of 3.1416, which he may have obtained from Archimedes or fromApollonius of Perga.^[48]^[49]Mathematicians using polygonal algorithms reached 39 digits of $π$ in 1630, a record only broken in 1699 when infinite series were used to reach 71 digits.^[50]

Inancient China,values for $π$ included 3.1547 (around 1 AD), ${\sqrt {10}}$ (100 AD, approximately 3.1623), and $⁠ 142 / 45 ⁠$ (3rd century, approximately 3.1556).^[51]Around 265 AD, theWei KingdommathematicianLiu Huicreated apolygon-based iterative algorithmand used it with a 3,072-sided polygon to obtain a value of $π$ of 3.1416.^[52]^[53]Liu later invented a faster method of calculating $π$ and obtained a value of 3.14 with a 96-sided polygon, by taking advantage of the fact that the differences in area of successive polygons form a geometric series with a factor of 4.^[52]The Chinese mathematicianZu Chongzhi,around 480 AD, calculated that $3.1415926<\pi <3.1415927$ and suggested the approximations ${\textstyle \pi \approx {\frac {355}{113}}=3.14159292035...}$ and ${\textstyle \pi \approx {\frac {22}{7}}=3.142857142857...}$ ,which he termed theMilü(''close ratio ") andYuelü( "approximate ratio" ), respectively, usingLiu Hui's algorithmapplied to a 12,288-sided polygon. With a correct value for its seven first decimal digits, this value remained the most accurate approximation of $π$ available for the next 800 years.^[54]

The Indian astronomerAryabhataused a value of 3.1416 in hisĀryabhaṭīya(499 AD).^[55]Fibonacciinc. 1220computed 3.1418 using a polygonal method, independent of Archimedes.^[56]Italian authorDanteapparently employed the value ${\textstyle 3+{\frac {\sqrt {2}}{10}}\approx 3.14142}$ .^[56]

The Persian astronomerJamshīd al-Kāshīproduced ninesexagesimaldigits, roughly the equivalent of 16 decimal digits, in 1424, using a polygon with ${\textstyle 3\times 2^{28}}$ sides,^[57]^[58]which stood as the world record for about 180 years.^[59]French mathematicianFrançois Viètein 1579 achieved nine digits with a polygon of ${\textstyle 3\times 2^{17}}$ sides.^[59]Flemish mathematicianAdriaan van Roomenarrived at 15 decimal places in 1593.^[59]In 1596, Dutch mathematicianLudolph van Ceulenreached 20 digits, a record he later increased to 35 digits (as a result, $π$ was called the "Ludolphian number" in Germany until the early 20th century).^[60]Dutch scientistWillebrord Snelliusreached 34 digits in 1621,^[61]and Austrian astronomerChristoph Grienbergerarrived at 38 digits in 1630 using 10⁴⁰sides.^[62]Christiaan Huygenswas able to arrive at 10 decimal places in 1654 using a slightly different method equivalent toRichardson extrapolation.^[63]^[64]

Infinite series

The calculation of $π$ was revolutionized by the development ofinfinite seriestechniques in the 16th and 17th centuries. An infinite series is the sum of the terms of an infinitesequence.Infinite series allowed mathematicians to compute $π$ with much greater precision thanArchimedesand others who used geometrical techniques.^[65]Although infinite series were exploited for $π$ most notably by European mathematicians such asJames GregoryandGottfried Wilhelm Leibniz,the approach also appeared in theKerala schoolsometime in the 14th or 15th century.^[66]^[67]Around 1500 AD, a written description of an infinite series that could be used to compute $π$ was laid out inSanskritverse inTantrasamgrahabyNilakantha Somayaji.^[66]The series are presented without proof, but proofs are presented in a later work,Yuktibhāṣā,from around 1530 AD. Several infinite series are described, including series for sine (which Nilakantha attributes toMadhava of Sangamagrama), cosine, and arctangent which are now sometimes referred to asMadhava series.The series for arctangent is sometimes calledGregory's seriesor the Gregory–Leibniz series.^[66]Madhava used infinite series to estimate $π$ to 11 digits around 1400.^[68]

In 1593,François Viètepublished what is now known asViète's formula,aninfinite product(rather than aninfinite sum,which is more typically used in $π$ calculations):^[69]^[70]^[71] ${\frac {2}{\pi }}={\frac {\sqrt {2}}{2}}\cdot {\frac {\sqrt {2+{\sqrt {2}}}}{2}}\cdot {\frac {\sqrt {2+{\sqrt {2+{\sqrt {2}}}}}}{2}}\cdots$

In 1655,John Wallispublished what is now known asWallis product,also an infinite product:^[69] ${\frac {\pi }{2}}={\Big (}{\frac {2}{1}}\cdot {\frac {2}{3}}{\Big )}\cdot {\Big (}{\frac {4}{3}}\cdot {\frac {4}{5}}{\Big )}\cdot {\Big (}{\frac {6}{5}}\cdot {\frac {6}{7}}{\Big )}\cdot {\Big (}{\frac {8}{7}}\cdot {\frac {8}{9}}{\Big )}\cdots$

A formal portrait of a man, with long hair — Isaac Newtonusedinfinite seriesto compute $π$ to 15 digits, later writing "I am ashamed to tell you to how many figures I carried these computations".^[72]

In the 1660s, the English scientistIsaac Newtonand German mathematicianGottfried Wilhelm Leibnizdiscoveredcalculus,which led to the development of many infinite series for approximating $π$ .Newton himself used an arcsine series to compute a 15-digit approximation of $π$ in 1665 or 1666, writing, "I am ashamed to tell you to how many figures I carried these computations, having no other business at the time."^[72]

In 1671,James Gregory,and independently, Leibniz in 1673, discovered theTaylor seriesexpansion forarctangent:^[66]^[73]^[74] $\arctan z=z-{\frac {z^{3}}{3}}+{\frac {z^{5}}{5}}-{\frac {z^{7}}{7}}+\cdots$

This series, sometimes called theGregory–Leibniz series,equals ${\textstyle {\frac {\pi }{4}}}$ when evaluated with $z=1$ .^[74]But for $z=1$ ,it converges impractically slowly(that is, approaches the answer very gradually), taking about ten times as many terms to calculate each additional digit.^[75]

In 1699, English mathematicianAbraham Sharpused the Gregory–Leibniz series for ${\textstyle z={\frac {1}{\sqrt {3}}}}$ to compute $π$ to 71 digits, breaking the previous record of 39 digits, which was set with a polygonal algorithm.^[76]

In 1706,John Machinused the Gregory–Leibniz series to produce an algorithm that converged much faster:^[3]^[77]^[78] ${\frac {\pi }{4}}=4\arctan {\frac {1}{5}}-\arctan {\frac {1}{239}}.$

Machin reached 100 digits of $π$ with this formula.^[79]Other mathematicians created variants, now known asMachin-like formulae,that were used to set several successive records for calculating digits of $π$ .^[80]^[79]

Isaac Newtonaccelerated the convergenceof the Gregory–Leibniz series in 1684 (in an unpublished work; others independently discovered the result):^[81]

\arctan x={\frac {x}{1+x^{2}}}+{\frac {2}{3}}{\frac {x^{3}}{(1+x^{2})^{2}}}+{\frac {2\cdot 4}{3\cdot 5}}{\frac {x^{5}}{(1+x^{2})^{3}}}+\cdots

Leonhard Eulerpopularized this series in his 1755 differential calculus textbook, and later used it with Machin-like formulae, including ${\textstyle {\tfrac {\pi }{4}}=5\arctan {\tfrac {1}{7}}+2\arctan {\tfrac {3}{79}},}$ with which he computed 20 digits of $π$ in one hour.^[82]

Machin-like formulae remained the best-known method for calculating $π$ well into the age of computers, and were used to set records for 250 years, culminating in a 620-digit approximation in 1946 by Daniel Ferguson – the best approximation achieved without the aid of a calculating device.^[83]

In 1844, a record was set byZacharias Dase,who employed a Machin-like formula to calculate 200 decimals of $π$ in his head at the behest of German mathematicianCarl Friedrich Gauss.^[84]

In 1853, British mathematicianWilliam Shankscalculated $π$ to 607 digits, but made a mistake in the 528th digit, rendering all subsequent digits incorrect. Though he calculated an additional 100 digits in 1873, bringing the total up to 707, his previous mistake rendered all the new digits incorrect as well.^[85]

Rate of convergence

Some infinite series for $π$ convergefaster than others. Given the choice of two infinite series for $π$ ,mathematicians will generally use the one that converges more rapidly because faster convergence reduces the amount of computation needed to calculate $π$ to any given accuracy.^[86]A simple infinite series for $π$ is theGregory–Leibniz series:^[87] $\pi ={\frac {4}{1}}-{\frac {4}{3}}+{\frac {4}{5}}-{\frac {4}{7}}+{\frac {4}{9}}-{\frac {4}{11}}+{\frac {4}{13}}-\cdots$

As individual terms of this infinite series are added to the sum, the total gradually gets closer to $π$ ,and – with a sufficient number of terms – can get as close to $π$ as desired. It converges quite slowly, though – after 500,000 terms, it produces only five correct decimal digits of $π$ .^[88]

An infinite series for $π$ (published by Nilakantha in the 15th century) that converges more rapidly than the Gregory–Leibniz series is:^[89]^[90] $\pi =3+{\frac {4}{2\times 3\times 4}}-{\frac {4}{4\times 5\times 6}}+{\frac {4}{6\times 7\times 8}}-{\frac {4}{8\times 9\times 10}}+\cdots$

The following table compares the convergence rates of these two series:

Infinite series for $π$	After 1st term	After 2nd term	After 3rd term	After 4th term	After 5th term	Converges to:
$\pi ={\frac {4}{1}}-{\frac {4}{3}}+{\frac {4}{5}}-{\frac {4}{7}}+{\frac {4}{9}}-{\frac {4}{11}}+{\frac {4}{13}}+\cdots$	4.0000	2.6666...	3.4666...	2.8952...	3.3396...	$π$ = 3.1415...
$\pi ={3}+{\frac {4}{2\times 3\times 4}}-{\frac {4}{4\times 5\times 6}}+{\frac {4}{6\times 7\times 8}}-\cdots$	3.0000	3.1666...	3.1333...	3.1452...	3.1396...	$π$ = 3.1415...

After five terms, the sum of the Gregory–Leibniz series is within 0.2 of the correct value of $π$ ,whereas the sum of Nilakantha's series is within 0.002 of the correct value. Nilakantha's series converges faster and is more useful for computing digits of $π$ .Series that converge even faster includeMachin's seriesandChudnovsky's series,the latter producing 14 correct decimal digits per term.^[86]

Irrationality and transcendence

Not all mathematical advances relating to $π$ were aimed at increasing the accuracy of approximations. When Euler solved theBasel problemin 1735, finding the exact value of the sum of the reciprocal squares, he established a connection between $π$ and theprime numbersthat later contributed to the development and study of theRiemann zeta function:^[91]

${\frac {\pi ^{2}}{6}}={\frac {1}{1^{2}}}+{\frac {1}{2^{2}}}+{\frac {1}{3^{2}}}+{\frac {1}{4^{2}}}+\cdots$

Swiss scientistJohann Heinrich Lambertin 1768 proved that $π$ isirrational,meaning it is not equal to the quotient of any two integers.^[20]Lambert's proofexploited a continued-fraction representation of the tangent function.^[92]French mathematicianAdrien-Marie Legendreproved in 1794 that $π$ ²is also irrational. In 1882, German mathematicianFerdinand von Lindemannproved that $π$ istranscendental,^[93]confirming a conjecture made by bothLegendreand Euler.^[94]^[95]Hardy and Wright states that "the proofs were afterwards modified and simplified by Hilbert, Hurwitz, and other writers".^[96]

Adoption of the symbol $π$

The earliest known use of the Greek letter π to represent the ratio of a circle's circumference to its diameter was by Welsh mathematicianWilliam Jonesin 1706

Leonhard Eulerpopularized the use of the Greek letter π in works he published in 1736 and 1748.

In the earliest usages, theGreek letter $π$ was used to denote thesemiperimeter(semiperipheriain Latin) of a circle^[8]and was combined in ratios with $δ$ (fordiameteror semidiameter) or $ρ$ (forradius) to form circle constants.^[97]^[98]^[99]^[100](Before then, mathematicians sometimes used letters such as $c$ or $p$ instead.^[101]) The first recorded use isOughtred's" $\delta.\pi$ ",to express the ratio of periphery and diameter in the 1647 and later editions ofClavis Mathematicae.^[102]^[101]Barrowlikewise used" ${\textstyle {\frac {\pi }{\delta }}}$ "to represent the constant $3.14...$ ,^[103]whileGregoryinstead used" ${\textstyle {\frac {\pi }{\rho }}}$ "to represent $6.28...$ .^[104]^[99]

The earliest known use of the Greek letter $π$ alone to represent the ratio of a circle's circumference to its diameter was by Welsh mathematicianWilliam Jonesin his 1706 workSynopsis Palmariorum Matheseos;or, a New Introduction to the Mathematics.^[3]^[105]The Greek letter appears on p. 243 in the phrase " ${\textstyle {\tfrac {1}{2}}}$ Periphery ( $π$ ) ", calculated for a circle with radius one. However, Jones writes that his equations for $π$ are from the "ready pen of the truly ingenious Mr.John Machin",leading to speculation that Machin may have employed the Greek letter before Jones.^[101]Jones' notation was not immediately adopted by other mathematicians, with the fraction notation still being used as late as 1767.^[97]^[106]

Eulerstarted using the single-letter form beginning with his 1727Essay Explaining the Properties of Air,though he used $π = 6.28...$ ,the ratio of periphery to radius, in this and some later writing.^[107]^[108]Euler first used $π$ = 3.14...in his 1736 workMechanica,^[109]and continued in his widely read 1748 workIntroductio in analysin infinitorum(he wrote: "for the sake of brevity we will write this number as $π$ ;thus $π$ is equal to half the circumference of a circle of radius $1$ ").^[110]Because Euler corresponded heavily with other mathematicians in Europe, the use of the Greek letter spread rapidly, and the practice was universally adopted thereafter in theWestern world,^[101]though the definition still varied between $3.14...$ and $6.28...$ as late as 1761.^[111]

Modern quest for more digits

Computer era and iterative algorithms

TheGauss–Legendre iterative algorithm:
Initialize $\textstyle a_{0}=1,\quad b_{0}={\frac {1}{\sqrt {2}}},\quad t_{0}={\frac {1}{4}},\quad p_{0}=1.$ Iterate $\textstyle a_{n+1}={\frac {a_{n}+b_{n}}{2}},\quad \quad b_{n+1}={\sqrt {a_{n}b_{n}}},$ $\textstyle t_{n+1}=t_{n}-p_{n}(a_{n}-a_{n+1})^{2},\quad \quad p_{n+1}=2p_{n}.$ Then an estimate for $π$ is given by $\textstyle \pi \approx {\frac {(a_{n}+b_{n})^{2}}{4t_{n}}}.$

The development of computers in the mid-20th century again revolutionized the hunt for digits of $π$ .MathematiciansJohn Wrenchand Levi Smith reached 1,120 digits in 1949 using a desk calculator.^[112]Using aninverse tangent(arctan) infinite series, a team led by George Reitwiesner andJohn von Neumannthat same year achieved 2,037 digits with a calculation that took 70 hours of computer time on theENIACcomputer.^[113]^[114]The record, always relying on an arctan series, was broken repeatedly (3089 digits in 1955,^[115]7,480 digits in 1957; 10,000 digits in 1958; 100,000 digits in 1961) until 1 million digits were reached in 1973.^[113]

Two additional developments around 1980 once again accelerated the ability to compute $π$ .First, the discovery of newiterative algorithmsfor computing $π$ ,which were much faster than the infinite series; and second, the invention offast multiplication algorithmsthat could multiply large numbers very rapidly.^[116]Such algorithms are particularly important in modern $π$ computations because most of the computer's time is devoted to multiplication.^[117]They include theKaratsuba algorithm,Toom–Cook multiplication,andFourier transform-based methods.^[118]

The iterative algorithms were independently published in 1975–1976 by physicistEugene Salaminand scientistRichard Brent.^[119]These avoid reliance on infinite series. An iterative algorithm repeats a specific calculation, each iteration using the outputs from prior steps as its inputs, and produces a result in each step that converges to the desired value. The approach was actually invented over 160 years earlier byCarl Friedrich Gauss,in what is now termed thearithmetic–geometric mean method(AGM method) orGauss–Legendre algorithm.^[119]As modified by Salamin and Brent, it is also referred to as the Brent–Salamin algorithm.

The iterative algorithms were widely used after 1980 because they are faster than infinite series algorithms: whereas infinite series typically increase the number of correct digits additively in successive terms, iterative algorithms generallymultiplythe number of correct digits at each step. For example, the Brent–Salamin algorithm doubles the number of digits in each iteration. In 1984, brothersJohnandPeter Borweinproduced an iterative algorithm that quadruples the number of digits in each step; and in 1987, one that increases the number of digits five times in each step.^[120]Iterative methods were used by Japanese mathematicianYasumasa Kanadato set several records for computing $π$ between 1995 and 2002.^[121]This rapid convergence comes at a price: the iterative algorithms require significantly more memory than infinite series.^[121]

Motives for computing $π$

For most numerical calculations involving $π$ ,a handful of digits provide sufficient precision. According to Jörg Arndt and Christoph Haenel, thirty-nine digits are sufficient to perform mostcosmologicalcalculations, because that is the accuracy necessary to calculate the circumference of theobservable universewith a precision of one atom. Accounting for additional digits needed to compensate for computationalround-off errors,Arndt concludes that a few hundred digits would suffice for any scientific application. Despite this, people have worked strenuously to compute $π$ to thousands and millions of digits.^[122]This effort may be partly ascribed to the human compulsion to break records, and such achievements with $π$ often make headlines around the world.^[123]^[124]They also have practical benefits, such as testingsupercomputers,testing numerical analysis algorithms (includinghigh-precision multiplication algorithms); and within pure mathematics itself, providing data for evaluating the randomness of the digits of $π$ .^[125]

Rapidly convergent series

Modern $π$ calculators do not use iterative algorithms exclusively. New infinite series were discovered in the 1980s and 1990s that are as fast as iterative algorithms, yet are simpler and less memory intensive.^[121]The fast iterative algorithms were anticipated in 1914, when Indian mathematicianSrinivasa Ramanujanpublished dozens of innovative new formulae for $π$ ,remarkable for their elegance, mathematical depth and rapid convergence.^[126]One of his formulae, based onmodular equations,is ${\frac {1}{\pi }}={\frac {2{\sqrt {2}}}{9801}}\sum _{k=0}^{\infty }{\frac {(4k)!(1103+26390k)}{k!^{4}\left(396^{4k}\right)}}.$

This series converges much more rapidly than most arctan series, including Machin's formula.^[127]Bill Gosperwas the first to use it for advances in the calculation of $π$ ,setting a record of 17 million digits in 1985.^[128]Ramanujan's formulae anticipated the modern algorithms developed by the Borwein brothers (JonathanandPeter) and theChudnovsky brothers.^[129]TheChudnovsky formuladeveloped in 1987 is ${\frac {1}{\pi }}={\frac {\sqrt {10005}}{4270934400}}\sum _{k=0}^{\infty }{\frac {(6k)!(13591409+545140134k)}{(3k)!\,k!^{3}(-640320)^{3k}}}.$

It produces about 14 digits of $π$ per term^[130]and has been used for several record-setting $π$ calculations, including the first to surpass 1 billion (10⁹) digits in 1989 by the Chudnovsky brothers, 10 trillion (10¹³) digits in 2011 by Alexander Yee and Shigeru Kondo,^[131]and 100 trillion digits byEmma Haruka Iwaoin 2022.^[132]For similar formulae, see also theRamanujan–Sato series.

In 2006, mathematicianSimon Plouffeused the PSLQinteger relation algorithm^[133]to generate several new formulae for $π$ ,conforming to the following template: $\pi ^{k}=\sum _{n=1}^{\infty }{\frac {1}{n^{k}}}\left({\frac {a}{q^{n}-1}}+{\frac {b}{q^{2n}-1}}+{\frac {c}{q^{4n}-1}}\right),$ where $q$ is $e π$ (Gelfond's constant), $k$ is anodd number,and $a, b, c$ are certain rational numbers that Plouffe computed.^[134]

Monte Carlo methods

Buffon's needle.Needlesaandbare dropped randomly.

Random dots are placed on a square and a circle inscribed inside.

Monte Carlo methods,which evaluate the results of multiple random trials, can be used to create approximations of $π$ .^[135]Buffon's needleis one such technique: If a needle of length $ℓ$ is dropped $n$ times on a surface on which parallel lines are drawn $t$ units apart, and if $x$ of those times it comes to rest crossing a line ( $x$ > 0), then one may approximate $π$ based on the counts:^[136] $\pi \approx {\frac {2n\ell }{xt}}.$

Another Monte Carlo method for computing $π$ is to draw a circle inscribed in a square, and randomly place dots in the square. The ratio of dots inside the circle to the total number of dots will approximately equal $π/4$ .^[137]

Another way to calculate $π$ using probability is to start with arandom walk,generated by a sequence of (fair) coin tosses: independentrandom variables $X k$ such that $X k \in {-1,1}$ with equal probabilities. The associated random walk is $W_{n}=\sum _{k=1}^{n}X_{k}$ so that, for each $n$ , $W n$ is drawn from a shifted and scaledbinomial distribution.As $n$ varies, $W n$ defines a (discrete)stochastic process.Then $π$ can be calculated by^[138] $\pi =\lim _{n\to \infty }{\frac {2n}{E[|W_{n}|]^{2}}}.$

This Monte Carlo method is independent of any relation to circles, and is a consequence of thecentral limit theorem,discussedbelow.

These Monte Carlo methods for approximating $π$ are very slow compared to other methods, and do not provide any information on the exact number of digits that are obtained. Thus they are never used to approximate $π$ when speed or accuracy is desired.^[139]

Spigot algorithms

Two algorithms were discovered in 1995 that opened up new avenues of research into $π$ .They are calledspigot algorithmsbecause, like water dripping from aspigot,they produce single digits of $π$ that are not reused after they are calculated.^[140]^[141]This is in contrast to infinite series or iterative algorithms, which retain and use all intermediate digits until the final result is produced.^[140]

MathematiciansStan Wagonand Stanley Rabinowitz produced a simple spigot algorithm in 1995.^[141]^[142]^[143]Its speed is comparable to arctan algorithms, but not as fast as iterative algorithms.^[142]

Another spigot algorithm, theBBP digit extraction algorithm,was discovered in 1995 by Simon Plouffe:^[144]^[145] $\pi =\sum _{k=0}^{\infty }{\frac {1}{16^{k}}}\left({\frac {4}{8k+1}}-{\frac {2}{8k+4}}-{\frac {1}{8k+5}}-{\frac {1}{8k+6}}\right).$

This formula, unlike others before it, can produce any individualhexadecimaldigit of $π$ without calculating all the preceding digits.^[144]Individual binary digits may be extracted from individual hexadecimal digits, andoctaldigits can be extracted from one or two hexadecimal digits. An important application of digit extraction algorithms is to validate new claims of record $π$ computations: After a new record is claimed, the decimal result is converted to hexadecimal, and then a digit extraction algorithm is used to calculate several randomly selected hexadecimal digits near the end; if they match, this provides a measure of confidence that the entire computation is correct.^[131]

Between 1998 and 2000, thedistributed computingprojectPiHexusedBellard's formula(a modification of the BBP algorithm) to compute the quadrillionth (10¹⁵th) bit of $π$ ,which turned out to be 0.^[146]In September 2010, aYahoo!employee used the company'sHadoopapplication on one thousand computers over a 23-day period to compute 256bitsof $π$ at the two-quadrillionth (2×10¹⁵th) bit, which also happens to be zero.^[147]

In 2022, Plouffe found a base-10 algorithm for calculating digits of $π$ .^[148]

Role and characterizations in mathematics

Because $π$ is closely related to the circle, it is found inmany formulaefrom the fields of geometry and trigonometry, particularly those concerning circles, spheres, or ellipses. Other branches of science, such as statistics, physics,Fourier analysis,and number theory, also include $π$ in some of their important formulae.

Geometry and trigonometry

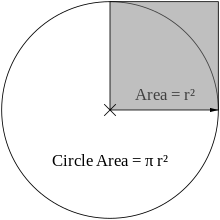
$π$ appears in formulae for areas and volumes of geometrical shapes based on circles, such asellipses,spheres,cones,andtori.Below are some of the more common formulae that involve $π$ .^[149]

The circumference of a circle with radius $r$ is $2π r$ .
Thearea of a circlewith radius $r$ is $π r 2$ .
The area of an ellipse with semi-major axis $a$ and semi-minor axis $b$ is $π ab$ .
The volume of a sphere with radius $r$ is $⁠ 4 / 3 ⁠ π r 3$ .
The surface area of a sphere with radius $r$ is $4π r 2$ .

Some of the formulae above are special cases of the volume of then-dimensional balland the surface area of its boundary, the(n−1)-dimensional sphere,givenbelow.

Apart from circles, there are othercurves of constant width.ByBarbier's theorem,every curve of constant width has perimeter $π$ times its width. TheReuleaux triangle(formed by the intersection of three circles with the sides of anequilateral triangleas their radii) has the smallest possible area for its width and the circle the largest. There also exist non-circularsmoothand evenalgebraic curvesof constant width.^[150]

Definite integralsthat describe circumference, area, or volume of shapes generated by circles typically have values that involve $π$ .For example, an integral that specifies half the area of a circle of radius one is given by:^[151] $\int _{-1}^{1}{\sqrt {1-x^{2}}}\,dx={\frac {\pi }{2}}.$

In that integral, the function ${\sqrt {1-x^{2}}}$ represents the height over the $x$ -axis of asemicircle(thesquare rootis a consequence of thePythagorean theorem), and the integral computes the area below the semicircle.

Units of angle

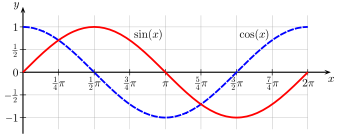
Thetrigonometric functionsrely on angles, and mathematicians generally use radians as units of measurement. $π$ plays an important role in angles measured inradians,which are defined so that a complete circle spans an angle of 2 $π$ radians. The angle measure of 180° is equal to $π$ radians, and1° = $π$ /180 radians.^[152]

Common trigonometric functions have periods that are multiples of $π$ ;for example, sine and cosine have period 2 $π$ ,^[153]so for any angle $θ$ and any integer $k$ ,^[153] $\sin \theta =\sin \left(\theta +2\pi k\right){\text{ and }}\cos \theta =\cos \left(\theta +2\pi k\right).$

Eigenvalues

Many of the appearances of $π$ in the formulae of mathematics and the sciences have to do with its close relationship with geometry. However, $π$ also appears in many natural situations having apparently nothing to do with geometry.

In many applications, it plays a distinguished role as aneigenvalue.For example, an idealizedvibrating stringcan be modelled as the graph of a function $f$ on the unit interval $[0, 1]$ ,withfixed ends $f (0) = f (1) = 0$ .The modes of vibration of the string are solutions of thedifferential equation $f''(x)+\lambda f(x)=0$ ,or $f''(t)=-\lambda f(x)$ .Thus $λ$ is an eigenvalue of the second derivativeoperator $f\mapsto f''$ ,and is constrained bySturm–Liouville theoryto take on only certain specific values. It must be positive, since the operator isnegative definite,so it is convenient to write $λ = ν 2$ ,where $ν > 0$ is called thewavenumber.Then $f (x) = sin(π x)$ satisfies the boundary conditions and the differential equation with $ν = π$ .^[154]

The value $π$ is, in fact, theleastsuch value of the wavenumber, and is associated with thefundamental modeof vibration of the string. One way to show this is by estimating theenergy,which satisfiesWirtinger's inequality:^[155]for a function $f:[0,1]\to \mathbb {C}$ with $f (0) = f (1) = 0$ and $f$ , $f'$ bothsquare integrable,we have: $\pi ^{2}\int _{0}^{1}|f(x)|^{2}\,dx\leq \int _{0}^{1}|f'(x)|^{2}\,dx,$ with equality precisely when $f$ is a multiple of $sin(π x)$ .Here $π$ appears as an optimal constant in Wirtinger's inequality, and it follows that it is the smallest wavenumber, using thevariational characterizationof the eigenvalue. As a consequence, $π$ is the smallestsingular valueof the derivative operator on the space of functions on $[0, 1]$ vanishing at both endpoints (theSobolev space $H_{0}^{1}[0,1]$ ).

Inequalities

The number $π$ serves appears in similar eigenvalue problems in higher-dimensional analysis. As mentionedabove,it can be characterized via its role as the best constant in theisoperimetric inequality:the area $A$ enclosed by a planeJordan curveof perimeter $P$ satisfies the inequality $4\pi A\leq P^{2},$ and equality is clearly achieved for the circle, since in that case $A = π r 2$ and $P = 2π r$ .^[157]

Ultimately, as a consequence of the isoperimetric inequality, $π$ appears in the optimal constant for the criticalSobolev inequalityinndimensions, which thus characterizes the role of $π$ in many physical phenomena as well, for example those of classicalpotential theory.^[158]^[159]^[160]In two dimensions, the critical Sobolev inequality is $2\pi \|f\|_{2}\leq \|\nabla f\|_{1}$ forfa smooth function with compact support in $R 2$ , $\nabla f$ is thegradientoff,and $\|f\|_{2}$ and $\|\nabla f\|_{1}$ refer respectively to the $L 2$ and $L 1$ -norm.The Sobolev inequality is equivalent to the isoperimetric inequality (in any dimension), with the same best constants.

Wirtinger's inequality also generalizes to higher-dimensionalPoincaré inequalitiesthat provide best constants for theDirichlet energyof ann-dimensional membrane. Specifically, $π$ is the greatest constant such that $\pi \leq {\frac {\left(\int _{G}|\nabla u|^{2}\right)^{1/2}}{\left(\int _{G}|u|^{2}\right)^{1/2}}}$ for allconvexsubsets $G$ of $R n$ of diameter 1, and square-integrable functionsuon $G$ of mean zero.^[161]Just as Wirtinger's inequality is thevariationalform of theDirichlet eigenvalueproblem in one dimension, the Poincaré inequality is the variational form of theNeumanneigenvalue problem, in any dimension.

Fourier transform and Heisenberg uncertainty principle

The constant $π$ also appears as a critical spectral parameter in theFourier transform.This is theintegral transform,that takes a complex-valued integrable function $f$ on the real line to the function defined as: ${\hat {f}}(\xi )=\int _{-\infty }^{\infty }f(x)e^{-2\pi ix\xi }\,dx.$

Although there are several different conventions for the Fourier transform and its inverse, any such convention must involve $π$ somewhere.The above is the most canonical definition, however, giving the unique unitary operator on $L 2$ that is also an algebra homomorphism of $L 1$ to $L \infty$ .^[162]

TheHeisenberg uncertainty principlealso contains the number $π$ .The uncertainty principle gives a sharp lower bound on the extent to which it is possible to localize a function both in space and in frequency: with our conventions for the Fourier transform, $\left(\int _{-\infty }^{\infty }x^{2}|f(x)|^{2}\,dx\right)\left(\int _{-\infty }^{\infty }\xi ^{2}|{\hat {f}}(\xi )|^{2}\,d\xi \right)\geq \left({\frac {1}{4\pi }}\int _{-\infty }^{\infty }|f(x)|^{2}\,dx\right)^{2}.$

The physical consequence, about the uncertainty in simultaneous position and momentum observations of aquantum mechanicalsystem, isdiscussed below.The appearance of $π$ in the formulae of Fourier analysis is ultimately a consequence of theStone–von Neumann theorem,asserting the uniqueness of theSchrödinger representationof theHeisenberg group.^[163]

Gaussian integrals

A graph of theGaussian function $ƒ (x) = e - x 2$ .The coloured region between the function and thex-axis has area $\sqrt π$ .

The fields ofprobabilityandstatisticsfrequently use thenormal distributionas a simple model for complex phenomena; for example, scientists generally assume that the observational error in most experiments follows a normal distribution.^[164]TheGaussian function,which is theprobability density functionof the normal distribution withmean $μ$ andstandard deviation $σ$ ,naturally contains $π$ :^[165] $f(x)={1 \over \sigma {\sqrt {2\pi }}}\,e^{-(x-\mu )^{2}/(2\sigma ^{2})}.$

The factor of ${\tfrac {1}{\sqrt {2\pi }}}$ makes the area under the graph of $f$ equal to one, as is required for a probability distribution. This follows from achange of variablesin theGaussian integral:^[165] $\int _{-\infty }^{\infty }e^{-u^{2}}\,du={\sqrt {\pi }}$ which says that the area under the basicbell curvein the figure is equal to the square root of $π$ .

Thecentral limit theoremexplains the central role of normal distributions, and thus of $π$ ,in probability and statistics. This theorem is ultimately connected with thespectral characterizationof $π$ as the eigenvalue associated with the Heisenberg uncertainty principle, and the fact that equality holds in the uncertainty principle only for the Gaussian function.^[166]Equivalently, $π$ is the unique constant making the Gaussian normal distribution $e -π x 2$ equal to its own Fourier transform.^[167]Indeed, according toHowe (1980),the "whole business" of establishing the fundamental theorems of Fourier analysis reduces to the Gaussian integral.^[163]

Topology

The constant $π$ appears in theGauss–Bonnet formulawhich relates thedifferential geometry of surfacesto theirtopology.Specifically, if acompactsurface $Σ$ hasGauss curvatureK,then $\int _{\Sigma }K\,dA=2\pi \chi (\Sigma )$ where $χ (Σ)$ is theEuler characteristic,which is an integer.^[168]An example is the surface area of a sphereSof curvature 1 (so that itsradius of curvature,which coincides with its radius, is also 1.) The Euler characteristic of a sphere can be computed from itshomology groupsand is found to be equal to two. Thus we have $A(S)=\int _{S}1\,dA=2\pi \cdot 2=4\pi$ reproducing the formula for the surface area of a sphere of radius 1.

The constant appears in many other integral formulae in topology, in particular, those involvingcharacteristic classesvia theChern–Weil homomorphism.^[169]

Cauchy's integral formula

Complex analytic functions can be visualized as a collection of streamlines and equipotentials, systems of curves intersecting at right angles. Here illustrated is the complex logarithm of the Gamma function.

One of the key tools incomplex analysisiscontour integrationof a function over a positively oriented (rectifiable)Jordan curve $γ$ .A form ofCauchy's integral formulastates that if a point $z 0$ is interior to $γ$ ,then^[170] $\oint _{\gamma }{\frac {dz}{z-z_{0}}}=2\pi i.$

Although the curve $γ$ is not a circle, and hence does not have any obvious connection to the constant $π$ ,a standard proof of this result usesMorera's theorem,which implies that the integral is invariant underhomotopyof the curve, so that it can be deformed to a circle and then integrated explicitly in polar coordinates. More generally, it is true that if a rectifiable closed curve $γ$ does not contain $z 0$ ,then the above integral is $2π i$ times thewinding numberof the curve.

The general form of Cauchy's integral formula establishes the relationship between the values of acomplex analytic function $f (z)$ on the Jordan curve $γ$ and the value of $f (z)$ at any interior point $z 0$ of $γ$ :^[171] $\oint _{\gamma }{f(z) \over z-z_{0}}\,dz=2\pi if(z_{0})$ provided $f (z)$ is analytic in the region enclosed by $γ$ and extends continuously to $γ$ .Cauchy's integral formula is a special case of theresidue theorem,that if $g (z)$ is ameromorphic functionthe region enclosed by $γ$ and is continuous in a neighbourhood of $γ$ ,then $\oint _{\gamma }g(z)\,dz=2\pi i\sum \operatorname {Res} (g,a_{k})$ where the sum is of theresiduesat thepolesof $g (z)$ .

Vector calculus and physics

The constant $π$ is ubiquitous invector calculusandpotential theory,for example inCoulomb's law,^[172]Gauss's law,Maxwell's equations,and even theEinstein field equations.^[173]^[174]Perhaps the simplest example of this is the two-dimensionalNewtonian potential,representing the potential of a point source at the origin, whose associated field has unit outwardfluxthrough any smooth and oriented closed surface enclosing the source: $\Phi (\mathbf {x} )={\frac {1}{2\pi }}\log |\mathbf {x} |.$ The factor of $1/2\pi$ is necessary to ensure that $\Phi$ is thefundamental solutionof thePoisson equationin $\mathbb {R} ^{2}$ :^[175] $\Delta \Phi =\delta$ where $\delta$ is theDirac delta function.

In higher dimensions, factors of $π$ are present because of a normalization by the n-dimensional volume of the unitn sphere.For example, in three dimensions, the Newtonian potential is:^[175] $\Phi (\mathbf {x} )=-{\frac {1}{4\pi |\mathbf {x} |}},$ which has the 2-dimensional volume (i.e., the area) of the unit 2-sphere in the denominator.

Total curvature

Inmathematicalstudy of thedifferential geometry of curves,thetotal curvatureof animmersed plane curveis theintegralofcurvaturealong a curve taken with respect toarc length:

\int _{a}^{b}k(s)\,ds=2\pi N.

The total curvature of a closed curve is always an integer multiple of 2

π

,whereNis called theindex of the curveorturning number– it is thewinding numberof the unittangent vectorabout the origin, or equivalently the degree of the map to theunit circleassigning to each point of the curve, the unit velocity vector at that point. This map is similar to theGauss mapfor surfaces.

The gamma function and Stirling's approximation

Thefactorialfunction $n!$ is the product of all of the positive integers through $n$ .Thegamma functionextends the concept offactorial(normally defined only for non-negative integers) to all complex numbers, except the negative real integers, with the identity $\Gamma (n)=(n-1)!$ .When the gamma function is evaluated at half-integers, the result contains $π$ .For example, $\Gamma (1/2)={\sqrt {\pi }}$ and ${\textstyle \Gamma (5/2)={\frac {3{\sqrt {\pi }}}{4}}}$ .^[176]

The gamma function is defined by itsWeierstrass productdevelopment:^[177] $\Gamma (z)={\frac {e^{-\gamma z}}{z}}\prod _{n=1}^{\infty }{\frac {e^{z/n}}{1+z/n}}$ where $γ$ is theEuler–Mascheroni constant.Evaluated at $z = 1/2$ and squared, the equation $Γ(1/2) 2 = π$ reduces to the Wallis product formula. The gamma function is also connected to theRiemann zeta functionand identities for thefunctional determinant,in which the constant $π$ plays an important role.

The gamma function is used to calculate the volume $V n (r)$ of then-dimensional ballof radiusrin Euclideann-dimensional space, and the surface area $S n -1 (r)$ of its boundary, the(n−1)-dimensional sphere:^[178] $V_{n}(r)={\frac {\pi ^{n/2}}{\Gamma \left({\frac {n}{2}}+1\right)}}r^{n},$ $S_{n-1}(r)={\frac {n\pi ^{n/2}}{\Gamma \left({\frac {n}{2}}+1\right)}}r^{n-1}.$

Further, it follows from thefunctional equationthat $2\pi r={\frac {S_{n+1}(r)}{V_{n}(r)}}.$

The gamma function can be used to create a simple approximation to the factorial function $n!$ for large $n$ : ${\textstyle n!\sim {\sqrt {2\pi n}}\left({\frac {n}{e}}\right)^{n}}$ which is known asStirling's approximation.^[179]Equivalently, $\pi =\lim _{n\to \infty }{\frac {e^{2n}n!^{2}}{2n^{2n+1}}}.$

As a geometrical application of Stirling's approximation, let $Δ n$ denote thestandard simplexinn-dimensional Euclidean space, and $(n + 1)Δ n$ denote the simplex having all of its sides scaled up by a factor of $n + 1$ .Then $\operatorname {Vol} ((n+1)\Delta _{n})={\frac {(n+1)^{n}}{n!}}\sim {\frac {e^{n+1}}{\sqrt {2\pi n}}}.$

Ehrhart's volume conjectureis that this is the (optimal) upper bound on the volume of aconvex bodycontaining only onelattice point.^[180]

Number theory and Riemann zeta function

Each prime has an associatedPrüfer group,which are arithmetic localizations of the circle. TheL-functionsof analytic number theory are also localized in each primep.

Solution of the Basel problem using theWeil conjecture:the value of $ζ (2)$ is thehyperbolicarea of a fundamental domain of themodular group,times $π /2$ .

TheRiemann zeta function $ζ (s)$ is used in many areas of mathematics. When evaluated at $s = 2$ it can be written as $\zeta (2)={\frac {1}{1^{2}}}+{\frac {1}{2^{2}}}+{\frac {1}{3^{2}}}+\cdots$

Finding asimple solutionfor this infinite series was a famous problem in mathematics called theBasel problem.Leonhard Eulersolved it in 1735 when he showed it was equal to $π 2 /6$ .^[91]Euler's result leads to thenumber theoryresult that the probability of two random numbers beingrelatively prime(that is, having no shared factors) is equal to $6/π 2$ .^[181]^[182]This probability is based on the observation that the probability that any number isdivisibleby a prime $p$ is $1/ p$ (for example, every 7th integer is divisible by 7.) Hence the probability that two numbers are both divisible by this prime is $1/ p 2$ ,and the probability that at least one of them is not is $1 - 1/ p 2$ .For distinct primes, these divisibility events are mutually independent; so the probability that two numbers are relatively prime is given by a product over all primes:^[183] ${\begin{aligned}\prod _{p}^{\infty }\left(1-{\frac {1}{p^{2}}}\right)&=\left(\prod _{p}^{\infty }{\frac {1}{1-p^{-2}}}\right)^{-1}\\[4pt]&={\frac {1}{1+{\frac {1}{2^{2}}}+{\frac {1}{3^{2}}}+\cdots }}\\[4pt]&={\frac {1}{\zeta (2)}}={\frac {6}{\pi ^{2}}}\approx 61\%.\end{aligned}}$

This probability can be used in conjunction with arandom number generatorto approximate $π$ using a Monte Carlo approach.^[184]

The solution to the Basel problem implies that the geometrically derived quantity $π$ is connected in a deep way to the distribution of prime numbers. This is a special case ofWeil's conjecture on Tamagawa numbers,which asserts the equality of similar such infinite products ofarithmeticquantities, localized at each primep,and ageometricalquantity: the reciprocal of the volume of a certainlocally symmetric space.In the case of the Basel problem, it is thehyperbolic 3-manifold $SL 2 (R) / SL 2 (Z)$ .^[185]

The zeta function also satisfies Riemann's functional equation, which involves $π$ as well as the gamma function: $\zeta (s)=2^{s}\pi ^{s-1}\ \sin \left({\frac {\pi s}{2}}\right)\ \Gamma (1-s)\ \zeta (1-s).$

Furthermore, the derivative of the zeta function satisfies $\exp(-\zeta '(0))={\sqrt {2\pi }}.$

A consequence is that $π$ can be obtained from thefunctional determinantof theharmonic oscillator.This functional determinant can be computed via a product expansion, and is equivalent to the Wallis product formula.^[186]The calculation can be recast inquantum mechanics,specifically thevariational approachto thespectrum of the hydrogen atom.^[187]

Fourier series

The constant $π$ also appears naturally inFourier seriesofperiodic functions.Periodic functions are functions on the group $T = R / Z$ of fractional parts of real numbers. The Fourier decomposition shows that a complex-valued function $f$ on $T$ can be written as an infinite linear superposition ofunitary charactersof $T$ .That is, continuousgroup homomorphismsfrom $T$ to thecircle group $U (1)$ of unit modulus complex numbers. It is a theorem that every character of $T$ is one of the complex exponentials $e_{n}(x)=e^{2\pi inx}$ .

There is a unique character on $T$ ,up to complex conjugation, that is a group isomorphism. Using theHaar measureon the circle group, the constant $π$ is half the magnitude of theRadon–Nikodym derivativeof this character. The other characters have derivatives whose magnitudes are positive integral multiples of 2 $π$ .^[19]As a result, the constant $π$ is the unique number such that the groupT,equipped with its Haar measure, isPontrjagin dualto thelatticeof integral multiples of 2 $π$ .^[189]This is a version of the one-dimensionalPoisson summation formula.

Modular forms and theta functions

The constant $π$ is connected in a deep way with the theory ofmodular formsandtheta functions.For example, theChudnovsky algorithminvolves in an essential way thej-invariantof anelliptic curve.

Modular formsareholomorphic functionsin theupper half planecharacterized by their transformation properties under themodular group $\mathrm {SL} _{2}(\mathbb {Z} )$ (or its various subgroups), a lattice in the group $\mathrm {SL} _{2}(\mathbb {R} )$ .An example is theJacobi theta function $\theta (z,\tau )=\sum _{n=-\infty }^{\infty }e^{2\pi inz+i\pi n^{2}\tau }$ which is a kind of modular form called aJacobi form.^[190]This is sometimes written in terms of thenome $q=e^{\pi i\tau }$ .

The constant $π$ is the unique constant making the Jacobi theta function anautomorphic form,which means that it transforms in a specific way. Certain identities hold for all automorphic forms. An example is $\theta (z+\tau,\tau )=e^{-\pi i\tau -2\pi iz}\theta (z,\tau ),$ which implies that $θ$ transforms as a representation under the discreteHeisenberg group.General modular forms and othertheta functionsalso involve $π$ ,once again because of theStone–von Neumann theorem.^[190]

Cauchy distribution and potential theory

TheCauchy distribution $g(x)={\frac {1}{\pi }}\cdot {\frac {1}{x^{2}+1}}$ is aprobability density function.The total probability is equal to one, owing to the integral: $\int _{-\infty }^{\infty }{\frac {1}{x^{2}+1}}\,dx=\pi.$

TheShannon entropyof the Cauchy distribution is equal to $ln(4π)$ ,which also involves $π$ .

The Cauchy distribution plays an important role inpotential theorybecause it is the simplestFurstenberg measure,the classicalPoisson kernelassociated with aBrownian motionin a half-plane.^[191]Conjugate harmonic functionsand so also theHilbert transformare associated with the asymptotics of the Poisson kernel. The Hilbert transformHis the integral transform given by theCauchy principal valueof thesingular integral $Hf(t)={\frac {1}{\pi }}\int _{-\infty }^{\infty }{\frac {f(x)\,dx}{x-t}}.$

The constant $π$ is the unique (positive) normalizing factor such thatHdefines alinear complex structureon the Hilbert space of square-integrable real-valued functions on the real line.^[192]The Hilbert transform, like the Fourier transform, can be characterized purely in terms of its transformation properties on the Hilbert space $L 2 (R)$ :up to a normalization factor, it is the unique bounded linear operator that commutes with positive dilations and anti-commutes with all reflections of the real line.^[193]The constant $π$ is the unique normalizing factor that makes this transformation unitary.

In the Mandelbrot set

An occurrence of $π$ in thefractalcalled theMandelbrot setwas discovered by David Boll in 1991.^[194]He examined the behaviour of the Mandelbrot set near the "neck" at $(-0.75, 0)$ .When the number of iterations until divergence for the point $(-0.75, ε)$ is multiplied by $ε$ ,the result approaches $π$ as $ε$ approaches zero. The point $(0.25 + ε,0)$ at the cusp of the large "valley" on the right side of the Mandelbrot set behaves similarly: the number of iterations until divergence multiplied by the square root of $ε$ tends to $π$ .^[194]^[195]

Projective geometry

Let $V$ be the set of all twice differentiable real functions $f:\mathbb {R} \to \mathbb {R}$ that satisfy theordinary differential equation $f''(x)+f(x)=0$ .Then $V$ is a two-dimensional realvector space,with two parameters corresponding to a pair ofinitial conditionsfor the differential equation. For any $t\in \mathbb {R}$ ,let $e_{t}:V\to \mathbb {R}$ be the evaluation functional, which associates to each $f\in V$ the value $e_{t}(f)=f(t)$ of the function $f$ at the real point $t$ .Then, for eacht,thekernelof $e_{t}$ is a one-dimensional linear subspace of $V$ .Hence $t\mapsto \ker e_{t}$ defines a function from $\mathbb {R} \to \mathbb {P} (V)$ from the real line to thereal projective line.This function is periodic, and the quantity $π$ can be characterized as the period of this map.^[196]This is notable in that the constant $π$ ,rather than 2 $π$ ,appears naturally in this context.

Outside mathematics

Describing physical phenomena

Although not aphysical constant, $π$ appears routinely in equations describing fundamental principles of the universe, often because of $π$ 's relationship to the circle and tospherical coordinate systems.A simple formula from the field ofclassical mechanicsgives the approximate period $T$ of a simplependulumof length $L$ ,swinging with a small amplitude ( $g$ is theearth's gravitational acceleration):^[197] $T\approx 2\pi {\sqrt {\frac {L}{g}}}.$

One of the key formulae ofquantum mechanicsisHeisenberg's uncertainty principle,which shows that the uncertainty in the measurement of a particle's position (Δ $x$ ) andmomentum(Δ $p$ ) cannot both be arbitrarily small at the same time (where $h$ is thePlanck constant):^[198] $\Delta x\,\Delta p\geq {\frac {h}{4\pi }}.$

The fact that $π$ is approximately equal to 3 plays a role in the relatively long lifetime oforthopositronium.The inverse lifetime to lowest order in thefine-structure constant $α$ is^[199] ${\frac {1}{\tau }}=2{\frac {\pi ^{2}-9}{9\pi }}m_{\text{e}}\ Alpha ^{6},$ where $m e$ is the mass of the electron.

$π$ is present in some structural engineering formulae, such as thebucklingformula derived by Euler, which gives the maximum axial load $F$ that a long, slender column of length $L$ ,modulus of elasticity $E$ ,andarea moment of inertia $I$ can carry without buckling:^[200] $F={\frac {\pi ^{2}EI}{L^{2}}}.$

The field offluid dynamicscontains $π$ inStokes' law,which approximates thefrictional force $F$ exerted on small,sphericalobjects of radius $R$ ,moving with velocity $v$ in afluidwithdynamic viscosity $η$ :^[201] $F=6\pi \eta Rv.$

In electromagnetics, thevacuum permeabilityconstantμ₀appears inMaxwell's equations,which describe the properties ofelectricandmagneticfields andelectromagnetic radiation.Before 20 May 2019, it was defined as exactly $\mu _{0}=4\pi \times 10^{-7}{\text{ H/m}}\approx 1.2566370614\ldots \times 10^{-6}{\text{ N/A}}^{2}.$

Memorizing digits

Piphilologyis the practice of memorizing large numbers of digits of $π$ ,^[202]and world-records are kept by theGuinness World Records.The record for memorizing digits of $π$ ,certified by Guinness World Records, is 70,000 digits, recited in India by Rajveer Meena in 9 hours and 27 minutes on 21 March 2015.^[203]In 2006,Akira Haraguchi,a retired Japanese engineer, claimed to have recited 100,000 decimal places, but the claim was not verified by Guinness World Records.^[204]

One common technique is to memorize a story or poem in which the word lengths represent the digits of $π$ :The first word has three letters, the second word has one, the third has four, the fourth has one, the fifth has five, and so on. Such memorization aids are calledmnemonics.An early example of a mnemonic for pi, originally devised by English scientistJames Jeans,is "How I want a drink, alcoholic of course, after the heavy lectures involving quantum mechanics."^[202]When a poem is used, it is sometimes referred to as apiem.^[205]Poems for memorizing $π$ have been composed in several languages in addition to English.^[202]Record-setting $π$ memorizers typically do not rely on poems, but instead use methods such as remembering number patterns and themethod of loci.^[206]

A few authors have used the digits of $π$ to establish a new form ofconstrained writing,where the word lengths are required to represent the digits of $π$ .TheCadaeic Cadenzacontains the first 3835 digits of $π$ in this manner,^[207]and the full-length bookNot a Wakecontains 10,000 words, each representing one digit of $π$ .^[208]

In popular culture

Perhaps because of the simplicity of its definition and its ubiquitous presence in formulae, $π$ has been represented in popular culture more than other mathematical constructs.^[209]

In thePalais de la Découverte(a science museum in Paris) there is a circular room known as thepi room.On its wall are inscribed 707 digits of $π$ .The digits are large wooden characters attached to the dome-like ceiling. The digits were based on an 1873 calculation by English mathematicianWilliam Shanks,which included an error beginning at the 528th digit. The error was detected in 1946 and corrected in 1949.^[210]

InCarl Sagan's 1985 novelContactit is suggested that the creator of the universe buried a message deep within the digits of $π$ .This part of the story was omitted from thefilmadaptation of the novel.^[211]^[212]The digits of $π$ have also been incorporated into the lyrics of the song "Pi" from the 2005 albumAerialbyKate Bush.^[213]In the 1967Star Trekepisode "Wolf in the Fold",an out-of-control computer is contained by being instructed to" Compute to the last digit the value of $π$ ".^[46]

In the United States,Pi Dayfalls on 14 March (written 3/14 in the US style), and is popular among students.^[46] $π$ and its digital representation are often used by self-described "mathgeeks"forinside jokesamong mathematically and technologically minded groups. Acollege cheervariously attributed to theMassachusetts Institute of Technologyor theRensselaer Polytechnic Instituteincludes "3.14159".^[214]^[215]Pi Day in 2015 was particularly significant because the date and time 3/14/15 9:26:53 reflected many more digits of pi.^[216]^[217]In parts of the world where dates are commonly noted in day/month/year format, 22 July represents "Pi Approximation Day", as 22/7 = 3.142857.^[218]

Some have proposed replacing $π$ by $τ = 2 π$ ,^[219]arguing that $τ$ ,as the number of radians in oneturnor the ratio of a circle's circumference to its radius, is more natural than $π$ and simplifies many formulae.^[220]^[221]This use of $τ$ has not made its way into mainstream mathematics,^[222]but since 2010 this has led to people celebrating Two Pi Day or Tau Day on June 28.^[223]

In 1897, an amateur mathematician attempted to persuade theIndiana legislatureto pass theIndiana Pi Bill,which described a method tosquare the circleand contained text that implied various incorrect values for $π$ ,including 3.2. The bill is notorious as an attempt to establish a value of mathematical constant by legislative fiat. The bill was passed by the Indiana House of Representatives, but rejected by the Senate, and thus it did not become a law.^[224]

In computer culture

In contemporaryinternet culture,individuals and organizations frequently pay homage to the number $π$ .For instance, thecomputer scientist Donald Knuthlet the version numbers of his programTeXapproach $π$ .The versions are 3, 3.1, 3.14, and so forth.^[225] $τ$ has been added to severalprogramming languagesas a predefined constant.^[226]^[227]

References

Explanatory notes

^In particular, $π$ is conjectured to be anormal number,which implies a specific kind of statistical randomness on its digits in all bases.
^The precise integral that Weierstrass used was $\pi =\int _{-\infty }^{\infty }{\frac {dx}{1+x^{2}}}.$ Remmert 2012,p. 148
^The polynomial shown is the first few terms of theTaylor seriesexpansion of thesinefunction.

Citations

^Andrews, Askey & Roy 1999,p. 59.
^Gupta, R. C. (1992). "On the remainder term in the Madhava–Leibniz's series".Ganita Bharati.14(1–4): 68–71.
^^a ^b ^cJones, William(1706).Synopsis Palmariorum Matheseos.London: J. Wale. pp.243,263.p. 263:There are various other ways of finding theLengths,orAreasof particularCurve LinesorPlanes,which may very much facilitate the Practice; as for instance, in theCircle,the Diameter is to Circumference as 1 to
${\overline {{\tfrac {16}{5}}-{\tfrac {4}{239}}}}-{\tfrac {1}{3}}{\overline {{\tfrac {16}{5^{3}}}-{\tfrac {4}{239^{3}}}}}+{\tfrac {1}{5}}{\overline {{\tfrac {16}{5^{5}}}-{\tfrac {4}{239^{5}}}}}-,\,\&c.=$
$3.14159, & c. = π$ .ThisSeries(among others for the same purpose, and drawn from the same Principle) I receiv'd from the Excellent Analyst, and my much Esteem'd Friend Mr.John Machin;and by means thereof,Van Ceulen's Number, or that in Art. 64.38. may be Examin'd with all desireable Ease and Dispatch.
Reprinted inSmith, David Eugene (1929)."William Jones: The First Use of $π$ for the Circle Ratio ".A Source Book in Mathematics.McGraw–Hill. pp. 346–347.
^"π^etrillion digits of π ".pi2e.ch.Archivedfrom the original on 6 December 2016.
^Haruka Iwao, Emma(14 March 2019)."Pi in the sky: Calculating a record-breaking 31.4 trillion digits of Archimedes' constant on Google Cloud".Google Cloud Platform.Archivedfrom the original on 19 October 2019.Retrieved12 April2019.
^Arndt & Haenel 2006,p. 17.
^Bailey, David H.; Plouffe, Simon M.; Borwein, Peter B.; Borwein, Jonathan M. (1997). "The quest for PI".The Mathematical Intelligencer.19(1): 50–56.CiteSeerX10.1.1.138.7085.doi:10.1007/BF03024340.ISSN 0343-6993.S2CID 14318695.
^^a ^bOughtred, William (1652).Theorematum in libris Archimedis de sphaera et cylindro declarario(in Latin). Excudebat L. Lichfield, Veneunt apud T. Robinson. $δ . π$ :: semidiameter. semiperipheria
^"pi".Dictionary.reference. 2 March 1993.Archivedfrom the original on 28 July 2014.Retrieved18 June2012.
^^a ^b ^cArndt & Haenel 2006,p. 8.
^Apostol, Tom(1967).Calculus.Vol. 1 (2nd ed.). Wiley. p. 102.From a logical point of view, this is unsatisfactory at the present stage because we have not yet discussed the concept of arc length
^^a ^b ^cRemmert 2012,p. 129.
^Baltzer, Richard (1870).Die Elemente der Mathematik[The Elements of Mathematics] (in German). Hirzel. p. 195.Archivedfrom the original on 14 September 2016.
^Landau, Edmund(1934).Einführung in die Differentialrechnung und Integralrechnung(in German). Noordoff. p. 193.
^^a ^bRudin, Walter (1976).Principles of Mathematical Analysis.McGraw-Hill. p. 183.ISBN 978-0-07-054235-8.
^Rudin, Walter (1986).Real and complex analysis.McGraw-Hill. p. 2.
^Ahlfors, Lars(1966).Complex analysis.McGraw-Hill. p. 46.
^Bourbaki, Nicolas(1981).Topologie generale.Springer. §VIII.2.
^^a ^bBourbaki, Nicolas(1979).Fonctions d'une variable réelle(in French). Springer. §II.3.
^^a ^bArndt & Haenel 2006,p. 5.
^Salikhov, V. (2008). "On the Irrationality Measure of pi".Russian Mathematical Surveys.53(3): 570–572.Bibcode:2008RuMaS..63..570S.doi:10.1070/RM2008v063n03ABEH004543.ISSN 0036-0279.S2CID 250798202.
^Arndt & Haenel 2006,pp. 22–23.
^Arndt & Haenel 2006,pp. 22, 28–30.
^Arndt & Haenel 2006,p. 3.
^Arndt & Haenel 2006,p. 6.
^Posamentier & Lehmann 2004,p. 25
^Eymard & Lafon 2004,p. 129
^Beckmann, Peter (1989) [1974].History of Pi.St. Martin's Press. p. 37.ISBN 978-0-88029-418-8.
^Schlager, Neil; Lauer, Josh (2001).Science and Its Times: Understanding the Social Significance of Scientific Discovery.Gale Group.ISBN 978-0-7876-3933-4.Archivedfrom the original on 13 December 2019.Retrieved19 December2019.,p. 185.
^^a ^bEymard & Lafon 2004,p. 78
^Arndt & Haenel 2006,p. 33.
^^a ^bMollin, R. A. (1999). "Continued fraction gems".Nieuw Archief voor Wiskunde.17(3): 383–405.MR 1743850.
^Lange, L.J. (May 1999). "An Elegant Continued Fraction for $π$ ".The American Mathematical Monthly.106(5): 456–458.doi:10.2307/2589152.JSTOR 2589152.
^Arndt & Haenel 2006,p. 240.
^Arndt & Haenel 2006,p. 242.
^Kennedy, E.S. (1978). "Abu-r-Raihan al-Biruni, 973–1048".Journal for the History of Astronomy.9:65.Bibcode:1978JHA.....9...65K.doi:10.1177/002182867800900106.S2CID 126383231.Ptolemyused a three-sexagesimal-digit approximation, andJamshīd al-Kāshīexpanded this to nine digits; seeAaboe, Asger(1964).Episodes from the Early History of Mathematics.New Mathematical Library. Vol. 13. New York: Random House. p. 125.ISBN 978-0-88385-613-0.Archivedfrom the original on 29 November 2016.
^Abramson 2014,Section 8.5: Polar form of complex numbers.
^^a ^bBronshteĭn & Semendiaev 1971,p. 592
^Maor, Eli (2009).E: The Story of a Number.Princeton University Press. p. 160.ISBN 978-0-691-14134-3.
^Andrews, Askey & Roy 1999,p. 14.
^^a ^bArndt & Haenel 2006,p. 167.
^Herz-Fischler, Roger (2000).The Shape of the Great Pyramid.Wilfrid Laurier University Press. pp. 67–77, 165–166.ISBN 978-0-88920-324-2.Archivedfrom the original on 29 November 2016.Retrieved5 June2013.
^Plofker, Kim (2009).Mathematics in India.Princeton University Press. p.27.ISBN 978-0691120676.
^Arndt & Haenel 2006,p. 170.
^Arndt & Haenel 2006,pp. 175, 205.
^^a ^b ^cBorwein, Jonathan M.(2014). "The life of $π$ :from Archimedes to ENIAC and beyond ". In Sidoli, Nathan; Van Brummelen, Glen (eds.).From Alexandria, through Baghdad: Surveys and studies in the ancient Greek and medieval Islamic mathematical sciences in honor of J. L. Berggren.Heidelberg: Springer. pp. 531–561.doi:10.1007/978-3-642-36736-6_24.ISBN 978-3-642-36735-9.MR 3203895.
^Arndt & Haenel 2006,p. 171.
^Arndt & Haenel 2006,p. 176.
^Boyer & Merzbach 1991,p. 168.
^Arndt & Haenel 2006,pp. 15–16, 175, 184–186, 205. Grienberger achieved 39 digits in 1630; Sharp 71 digits in 1699.
^Arndt & Haenel 2006,pp. 176–177.
^^a ^bBoyer & Merzbach 1991,p. 202
^Arndt & Haenel 2006,p. 177.
^Arndt & Haenel 2006,p. 178.
^Arndt & Haenel 2006,p. 179.
^^a ^bArndt & Haenel 2006,p. 180.
^Azarian, Mohammad K. (2010)."al-Risāla al-muhītīyya: A Summary".Missouri Journal of Mathematical Sciences.22(2): 64–85.doi:10.35834/mjms/1312233136.
^O'Connor, John J.; Robertson, Edmund F. (1999)."Ghiyath al-Din Jamshid Mas'ud al-Kashi".MacTutor History of Mathematics archive.Archivedfrom the original on 12 April 2011.Retrieved11 August2012.
^^a ^b ^cArndt & Haenel 2006,p. 182.
^Arndt & Haenel 2006,pp. 182–183.
^Arndt & Haenel 2006,p. 183.
^Grienbergerus, Christophorus(1630).Elementa Trigonometrica(PDF)(in Latin). Archived fromthe original(PDF)on 1 February 2014.His evaluation was 3.14159 26535 89793 23846 26433 83279 50288 4196 < $π$ < 3.14159 26535 89793 23846 26433 83279 50288 4199.
^Brezinski, C. (2009). "Some pioneers of extrapolation methods". InBultheel, Adhemar;Cools, Ronald (eds.).The Birth of Numerical Analysis.World Scientific. pp. 1–22.doi:10.1142/9789812836267_0001.ISBN 978-981-283-625-0.
^Yoder, Joella G. (1996)."Following in the footsteps of geometry: The mathematical world of Christiaan Huygens".De Zeventiende Eeuw.12:83–93 – viaDigital Library for Dutch Literature.
^Arndt & Haenel 2006,pp. 185–191
^^a ^b ^c ^dRoy, Ranjan (1990)."The Discovery of the Series Formula for $π$ by Leibniz, Gregory and Nilakantha "(PDF).Mathematics Magazine.63(5): 291–306.doi:10.1080/0025570X.1990.11977541.
^Arndt & Haenel 2006,pp. 185–186.
^Joseph, George Gheverghese (1991).The Crest of the Peacock: Non-European Roots of Mathematics.Princeton University Press. p. 264.ISBN 978-0-691-13526-7.
^^a ^bArndt & Haenel 2006,p. 187.
^OEIS:A060294
^Vieta, Franciscus (1593).Variorum de rebus mathematicis responsorum.Vol. VIII.
^^a ^bArndt & Haenel 2006,p. 188. Newton quoted by Arndt.
^Horvath, Miklos (1983)."On the Leibnizian quadrature of the circle"(PDF).Annales Universitatis Scientiarum Budapestiensis (Sectio Computatorica).4:75–83.
^^a ^bEymard & Lafon 2004,pp. 53–54
^Cooker, M.J. (2011)."Fast formulas for slowly convergent alternating series"(PDF).Mathematical Gazette.95(533): 218–226.doi:10.1017/S0025557200002928.S2CID 123392772.Archived from the original on 4 May 2019.Retrieved23 February2023.{{cite journal}}:CS1 maint: bot: original URL status unknown (link)
^Arndt & Haenel 2006,p. 189.
^Tweddle, Ian (1991). "John Machin and Robert Simson on Inverse-tangent Series for $π$ ".Archive for History of Exact Sciences.42(1): 1–14.doi:10.1007/BF00384331.JSTOR 41133896.S2CID 121087222.
^Arndt & Haenel 2006,pp. 192–193.
^^a ^bArndt & Haenel 2006,pp. 72–74
^Lehmer, D. H.(1938)."On Arccotangent Relations for $π$ "(PDF).American Mathematical Monthly.45(10): 657–664 Published by: Mathematical Association of America.doi:10.1080/00029890.1938.11990873.JSTOR 2302434.
^Roy, Ranjan (2021) [1st ed. 2011].Series and Products in the Development of Mathematics.Vol. 1 (2 ed.). Cambridge University Press. pp. 215–216, 219–220.
Newton, Isaac(1971).Whiteside, Derek Thomas(ed.).The Mathematical Papers of Isaac Newton.Vol. 4, 1674–1684. Cambridge University Press. pp. 526–653.
^ Sandifer, Ed (2009)."Estimating π"(PDF).How Euler Did It.Reprinted inHow Euler Did Even More.Mathematical Association of America. 2014. pp. 109–118.
Euler, Leonhard(1755)."§2.2.30".Institutiones Calculi Differentialis(in Latin). Academiae Imperialis Scientiarium Petropolitanae. p. 318.E 212.

Euler, Leonhard(1798) [written 1779]."Investigatio quarundam serierum, quae ad rationem peripheriae circuli ad diametrum vero proxime definiendam maxime sunt accommodatae".Nova Acta Academiae Scientiarum Petropolitinae.11:133–149, 167–168.E 705.

Chien-Lih, Hwang (2004). "88.38 Some Observations on the Method of Arctangents for the Calculation of $π$ ".Mathematical Gazette.88(512): 270–278.doi:10.1017/S0025557200175060.S2CID 123532808.

Chien-Lih, Hwang (2005). "89.67 An elementary derivation of Euler's series for the arctangent function".Mathematical Gazette.89(516): 469–470.doi:10.1017/S0025557200178404.S2CID 123395287.
^Arndt & Haenel 2006,pp. 192–196, 205.
^Arndt & Haenel 2006,pp. 194–196
^Hayes, Brian (September 2014)."Pencil, Paper, and Pi".American Scientist.Vol. 102, no. 5. p. 342.doi:10.1511/2014.110.342.Retrieved22 January2022.
^^a ^bBorwein, J.M.; Borwein, P.B. (1988). "Ramanujan and Pi".Scientific American.256(2): 112–117.Bibcode:1988SciAm.258b.112B.doi:10.1038/scientificamerican0288-112.
Arndt & Haenel 2006,pp. 15–17, 70–72, 104, 156, 192–197, 201–202
^Arndt & Haenel 2006,pp. 69–72.
^Borwein, J.M.; Borwein, P.B.; Dilcher, K. (1989). "Pi, Euler Numbers, and Asymptotic Expansions".American Mathematical Monthly.96(8): 681–687.doi:10.2307/2324715.hdl:1959.13/1043679.JSTOR 2324715.
^Arndt & Haenel 2006,Formula 16.10, p. 223.
^Wells, David (1997).The Penguin Dictionary of Curious and Interesting Numbers(revised ed.). Penguin. p. 35.ISBN 978-0-14-026149-3.
^^a ^bPosamentier & Lehmann 2004,p. 284
^Lambert, Johann, "Mémoire sur quelques propriétés remarquables des quantités transcendantes circulaires et logarithmiques", reprinted inBerggren, Borwein & Borwein 1997,pp. 129–140
^Lindemann, F.(1882)."Über die Ludolph'sche Zahl".Sitzungsberichte der Königlich Preussischen Akademie der Wissenschaften zu Berlin.2:679–682.
^Arndt & Haenel 2006,p. 196.
^Hardy and Wright 1938 and 2000: 177 footnote § 11.13–14 references Lindemann's proof as appearing atMath. Ann.20 (1882), 213–225.
^cf Hardy and Wright 1938 and 2000:177 footnote § 11.13–14. The proofs that e and π are transcendental can be found on pp. 170–176. They cite two sources of the proofs at Landau 1927 or Perron 1910; see the "List of Books" at pp. 417–419 for full citations.
^^a ^bCajori, Florian (2007).A History of Mathematical Notations: Vol. II.Cosimo, Inc. pp. 8–13.ISBN 978-1-60206-714-1.the ratio of the length of a circle to its diameter was represented in the fractional form by the use of two letters... J.A. Segner... in 1767, he represented $3.14159...$ by $δ : π$ ,as did Oughtred more than a century earlier
^Schepler, H.C. (1950) "The Chronology of Pi"Mathematics Magazine.23.
Part 1. Jan/Feb. (3): 165–170.doi:10.2307/3029284.
Part 2. Mar/Apr. (4): 216-228.doi:10.2307/3029832.
Part 3. May/Jun. (5): 279-283.doi:10.2307/3029000.
See p. 220:William Oughtredused the letter $π$ to represent the periphery (that is, the circumference) of a circle.
^^a ^bSmith, David E. (1958).History of Mathematics.Courier Corporation. p. 312.ISBN 978-0-486-20430-7.
^Archibald, R.C. (1921). "Historical Notes on the Relation $e -(π /2) = i i$ ".The American Mathematical Monthly.28(3): 116–121.doi:10.2307/2972388.JSTOR 2972388.It is noticeable that these letters areneverused separately, that is, $π$ isnotused for 'Semiperipheria'
^^a ^b ^c ^dArndt & Haenel 2006,p. 166.
^See, for example,Oughtred, William (1648).Clavis Mathematicæ[The key to mathematics] (in Latin). London: Thomas Harper. p.69.(English translation:Oughtred, William (1694).Key of the Mathematics.J. Salusbury.)
^Barrow, Isaac (1860)."Lecture XXIV".In Whewell, William (ed.).The mathematical works of Isaac Barrow(in Latin). Harvard University. Cambridge University press. p. 381.
^Gregorius, David (1695)."Ad Reverendum Virum D. Henricum Aldrich S.T.T. Decanum Aedis Christi Oxoniae"(PDF).Philosophical Transactions(in Latin).19(231): 637–652.Bibcode:1695RSPT...19..637G.doi:10.1098/rstl.1695.0114.JSTOR 102382.
^Arndt & Haenel 2006,p. 165: A facsimile of Jones' text is inBerggren, Borwein & Borwein 1997,pp. 108–109.
^Segner, Joannes Andreas (1756).Cursus Mathematicus(in Latin). Halae Magdeburgicae. p. 282.Archivedfrom the original on 15 October 2017.Retrieved15 October2017.
^Euler, Leonhard (1727)."Tentamen explicationis phaenomenorum aeris"(PDF).Commentarii Academiae Scientiarum Imperialis Petropolitana(in Latin).2:351.E007.Archived(PDF)from the original on 1 April 2016.Retrieved15 October2017.Sumatur pro ratione radii ad peripheriem, $I: π$ English translation by Ian Bruce Archived10 June 2016 at theWayback Machine:" $π$ is taken for the ratio of the radius to the periphery [note that in this work, Euler's $π$ is double our $π$ .] "
^Euler, Leonhard (1747). Henry, Charles (ed.).Lettres inédites d'Euler à d'Alembert.Bullettino di Bibliografia e di Storia delle Scienze Matematiche e Fisiche (in French). Vol. 19 (published 1886). p. 139.E858.Car, soit π la circonference d'un cercle, dout le rayon est $= 1$ English translation inCajori, Florian (1913). "History of the Exponential and Logarithmic Concepts".The American Mathematical Monthly.20(3): 75–84.doi:10.2307/2973441.JSTOR 2973441.Letting $π$ be the circumference (!) of a circle of unit radius
^Euler, Leonhard (1736)."Ch. 3 Prop. 34 Cor. 1".Mechanica sive motus scientia analytice exposita. (cum tabulis)(in Latin). Vol. 1. Academiae scientiarum Petropoli. p. 113.E015.Denotet $1: π$ rationem diametri ad peripheriamEnglish translation by Ian Bruce Archived10 June 2016 at theWayback Machine:"Let $1: π$ denote the ratio of the diameter to the circumference "
^Euler, Leonhard (1707–1783) (1922).Leonhardi Euleri opera omnia. 1, Opera mathematica. Volumen VIII, Leonhardi Euleri introductio in analysin infinitorum. Tomus primus / ediderunt Adolf Krazer et Ferdinand Rudio(in Latin). Lipsae: B.G. Teubneri. pp. 133–134.E101.Archivedfrom the original on 16 October 2017.Retrieved15 October2017.{{cite book}}:CS1 maint: numeric names: authors list (link)
^Segner, Johann Andreas von (1761).Cursus Mathematicus: Elementorum Analyseos Infinitorum Elementorum Analyseos Infinitorvm(in Latin). Renger. p. 374.Si autem $π$ notet peripheriam circuli, cuius diameter eſt $2$
^Arndt & Haenel 2006,p. 205.
^^a ^bArndt & Haenel 2006,p. 197.
^Reitwiesner, George (1950). "An ENIAC Determination of pi and e to 2000 Decimal Places".Mathematical Tables and Other Aids to Computation.4(29): 11–15.doi:10.2307/2002695.JSTOR 2002695.
^Nicholson, J. C.; Jeenel, J. (1955). "Some comments on a NORC Computation of π".Math. Tabl. Aids. Comp.9(52): 162–164.doi:10.2307/2002052.JSTOR 2002052.
^Arndt & Haenel 2006,pp. 15–17.
^Arndt & Haenel 2006,p. 131.
^Arndt & Haenel 2006,pp. 132, 140.
^^a ^bArndt & Haenel 2006,p. 87.
^Arndt & Haenel 2006,pp. 111 (5 times), pp. 113–114 (4 times). For details of algorithms, seeBorwein, Jonathan; Borwein, Peter (1987).Pi and the AGM: a Study in Analytic Number Theory and Computational Complexity.Wiley.ISBN 978-0-471-31515-5.
^^a ^b ^cBailey, David H. (16 May 2003)."Some Background on Kanada's Recent Pi Calculation"(PDF).Archived(PDF)from the original on 15 April 2012.Retrieved12 April2012.
^Arndt & Haenel 2006,pp. 17–19
^Schudel, Matt (25 March 2009). "John W. Wrench, Jr.: Mathematician Had a Taste for Pi".The Washington Post.p. B5.
^Connor, Steve (8 January 2010)."The Big Question: How close have we come to knowing the precise value of pi?".The Independent.London.Archivedfrom the original on 2 April 2012.Retrieved14 April2012.
^Arndt & Haenel 2006,p. 18.
^Arndt & Haenel 2006,pp. 103–104
^Arndt & Haenel 2006,p. 104
^Arndt & Haenel 2006,pp. 104, 206
^Arndt & Haenel 2006,pp. 110–111
^Eymard & Lafon 2004,p. 254
^^a ^bBailey, David H.;Borwein, Jonathan M.(2016)."15.2 Computational records".Pi: The Next Generation, A Sourcebook on the Recent History of Pi and Its Computation.Springer International Publishing. p. 469.doi:10.1007/978-3-319-32377-0.ISBN 978-3-319-32375-6.
^Cassel, David (11 June 2022)."How Google's Emma Haruka Iwao Helped Set a New Record for Pi".The New Stack.
^PSLQ means Partial Sum of Least Squares.
^Plouffe, Simon(April 2006)."Identities inspired by Ramanujan's Notebooks (part 2)"(PDF).Archived(PDF)from the original on 14 January 2012.Retrieved10 April2009.
^Arndt & Haenel 2006,p. 39
^Ramaley, J.F. (October 1969). "Buffon's Noodle Problem".The American Mathematical Monthly.76(8): 916–918.doi:10.2307/2317945.JSTOR 2317945.
^Arndt & Haenel 2006,pp. 39–40
Posamentier & Lehmann 2004,p. 105
^Grünbaum, B.(1960)."Projection Constants".Transactions of the American Mathematical Society.95(3): 451–465.doi:10.1090/s0002-9947-1960-0114110-9.
^Arndt & Haenel 2006,pp. 43
Posamentier & Lehmann 2004,pp. 105–108
^^a ^bArndt & Haenel 2006,pp. 77–84.
^^a ^bGibbons, Jeremy(2006)."Unbounded spigot algorithms for the digits of pi"(PDF).The American Mathematical Monthly.113(4): 318–328.doi:10.2307/27641917.JSTOR 27641917.MR 2211758.
^^a ^bArndt & Haenel 2006,p. 77.
^Rabinowitz, Stanley; Wagon, Stan (March 1995). "A spigot algorithm for the digits of Pi".American Mathematical Monthly.102(3): 195–203.doi:10.2307/2975006.JSTOR 2975006.
^^a ^bArndt & Haenel 2006,pp. 117, 126–128.
^Bailey, David H.;Borwein, Peter B.;Plouffe, Simon(April 1997)."On the Rapid Computation of Various Polylogarithmic Constants"(PDF).Mathematics of Computation.66(218): 903–913.Bibcode:1997MaCom..66..903B.CiteSeerX10.1.1.55.3762.doi:10.1090/S0025-5718-97-00856-9.S2CID 6109631.Archived(PDF)from the original on 22 July 2012.
^Arndt & Haenel 2006,p. 20
Bellards formula in:Bellard, Fabrice."A new formula to compute the n^thbinary digit of pi ".Archived fromthe originalon 12 September 2007.Retrieved27 October2007.
^Palmer, Jason (16 September 2010)."Pi record smashed as team finds two-quadrillionth digit".BBC News.Archivedfrom the original on 17 March 2011.Retrieved26 March2011.
^Plouffe, Simon (2022). "A formula for the $n$ th decimal digit or binary of $π$ and powers of $π$ ".arXiv:2201.12601[math.NT].
^Bronshteĭn & Semendiaev 1971,pp. 200, 209
^Martini, Horst; Montejano, Luis;Oliveros, Déborah(2019).Bodies of Constant Width: An Introduction to Convex Geometry with Applications.Birkhäuser.doi:10.1007/978-3-030-03868-7.ISBN 978-3-030-03866-3.MR 3930585.S2CID 127264210.

See Barbier's theorem, Corollary 5.1.1, p. 98; Reuleaux triangles, pp. 3, 10; smooth curves such as an analytic curve due to Rabinowitz, § 5.3.3, pp. 111–112.
^Herman, Edwin;Strang, Gilbert(2016)."Section 5.5, Exercise 316".Calculus.Vol. 1.OpenStax.p. 594.
^Abramson 2014,Section 5.1: Angles.
^^a ^bBronshteĭn & Semendiaev 1971,pp. 210–211
^Hilbert, David;Courant, Richard(1966).Methods of mathematical physics, volume 1.Wiley. pp. 286–290.
^Dym & McKean 1972,p. 47.
^Thompson, William(1894). "Isoperimetrical problems".Nature Series: Popular Lectures and Addresses.II:571–592.
^Chavel, Isaac (2001).Isoperimetric inequalities.Cambridge University Press.
^Talenti, Giorgio (1976). "Best constant in Sobolev inequality".Annali di Matematica Pura ed Applicata.110(1): 353–372.CiteSeerX10.1.1.615.4193.doi:10.1007/BF02418013.ISSN 1618-1891.S2CID 16923822.
^L. Esposito; C. Nitsch; C. Trombetti (2011). "Best constants in Poincaré inequalities for convex domains".arXiv:1110.2960[math.AP].
^Del Pino, M.; Dolbeault, J. (2002). "Best constants for Gagliardo–Nirenberg inequalities and applications to nonlinear diffusions".Journal de Mathématiques Pures et Appliquées.81(9): 847–875.CiteSeerX10.1.1.57.7077.doi:10.1016/s0021-7824(02)01266-7.S2CID 8409465.
^Payne, L.E.; Weinberger, H.F. (1960). "An optimal Poincaré inequality for convex domains".Archive for Rational Mechanics and Analysis.5(1): 286–292.Bibcode:1960ArRMA...5..286P.doi:10.1007/BF00252910.ISSN 0003-9527.S2CID 121881343.
^Folland, Gerald(1989).Harmonic analysis in phase space.Princeton University Press. p. 5.
^^a ^bHowe, Roger (1980)."On the role of the Heisenberg group in harmonic analysis".Bulletin of the American Mathematical Society.3(2): 821–844.doi:10.1090/S0273-0979-1980-14825-9.MR 0578375.
^Feller, W.An Introduction to Probability Theory and Its Applications, Vol. 1,Wiley, 1968, pp. 174–190.
^^a ^bBronshteĭn & Semendiaev 1971,pp. 106–107, 744, 748
^Dym & McKean 1972,Section 2.7.
^Stein, Elias;Weiss, Guido (1971).Fourier analysis on Euclidean spaces.Princeton University Press. p. 6.;Theorem 1.13.
^Spivak, Michael(1999).A Comprehensive Introduction to Differential Geometry.Vol. 3. Publish or Perish Press.;Chapter 6.
^Kobayashi, Shoshichi; Nomizu, Katsumi (1996).Foundations of Differential Geometry.Vol. 2 (New ed.).Wiley Interscience.p. 293.;Chapter XIICharacteristic classes
^Ahlfors, Lars(1966).Complex analysis.McGraw-Hill. p. 115.
^Joglekar, S. D. (2005).Mathematical Physics.Universities Press. p. 166.ISBN 978-81-7371-422-1.
^Schey, H. M. (1996).Div, Grad, Curl, and All That: An Informal Text on Vector Calculus.W.W. Norton.ISBN 0-393-96997-5.
^Yeo, Adrian (2006).The pleasures of pi, e and other interesting numbers.World Scientific Pub. p. 21.ISBN 978-981-270-078-0.
^Ehlers, Jürgen (2000).Einstein's Field Equations and Their Physical Implications.Springer. p. 7.ISBN 978-3-540-67073-5.
^^a ^bGilbarg, D.;Trudinger, Neil(1983),Elliptic Partial Differential Equations of Second Order,New York: Springer,ISBN 3-540-41160-7
^Bronshteĭn & Semendiaev 1971,pp. 191–192
^Artin, Emil(1964).The Gamma Function.Athena series; selected topics in mathematics (1st ed.). Holt, Rinehart and Winston.
^Evans, Lawrence (1997).Partial Differential Equations.AMS. p. 615.
^Bronshteĭn & Semendiaev 1971,p. 190
^Benjamin Nill; Andreas Paffenholz (2014). "On the equality case in Erhart's volume conjecture".Advances in Geometry.14(4): 579–586.arXiv:1205.1270.doi:10.1515/advgeom-2014-0001.ISSN 1615-7168.S2CID 119125713.
^Arndt & Haenel 2006,pp. 41–43
^This theorem was proved byErnesto Cesàroin 1881. For a more rigorous proof than the intuitive and informal one given here, seeHardy, G. H. (2008).An Introduction to the Theory of Numbers.Oxford University Press. Theorem 332.ISBN 978-0-19-921986-5.
^Ogilvy, C. S.;Anderson, J. T. (1988).Excursions in Number Theory.Dover Publications Inc. pp. 29–35.ISBN 0-486-25778-9.
^Arndt & Haenel 2006,p. 43
^Platonov, Vladimir; Rapinchuk, Andrei (1994).Algebraic Groups and Number Theory.Academic Press. pp. 262–265.
^Sondow, J. (1994). "Analytic continuation of Riemann's zeta function and values at negative integers via Euler's transformation of series".Proceedings of the American Mathematical Society.120(2): 421–424.CiteSeerX10.1.1.352.5774.doi:10.1090/s0002-9939-1994-1172954-7.S2CID 122276856.
^T. Friedmann; C.R. Hagen (2015). "Quantum mechanical derivation of the Wallis formula for pi".Journal of Mathematical Physics.56(11): 112101.arXiv:1510.07813.Bibcode:2015JMP....56k2101F.doi:10.1063/1.4930800.S2CID 119315853.
^Tate, John T. (1950). "Fourier analysis in number fields, and Hecke's zeta-functions". In Cassels, J. W. S.; Fröhlich, A. (eds.).Algebraic Number Theory (Proc. Instructional Conf., Brighton, 1965).Thompson, Washington, DC. pp. 305–347.ISBN 978-0-9502734-2-6.MR 0217026.
^Dym & McKean 1972,Chapter 4.
^^a ^bMumford, David(1983).Tata Lectures on Theta I.Boston: Birkhauser. pp. 1–117.ISBN 978-3-7643-3109-2.
^Port, Sidney; Stone, Charles (1978).Brownian motion and classical potential theory.Academic Press. p. 29.
^Titchmarsh, E.(1948).Introduction to the Theory of Fourier Integrals(2nd ed.). Oxford University: Clarendon Press (published 1986).ISBN 978-0-8284-0324-5.
^Stein, Elias(1970).Singular Integrals and Differentiability Properties of Functions.Princeton University Press.;Chapter II.
^^a ^bKlebanoff, Aaron (2001)."Pi in the Mandelbrot set"(PDF).Fractals.9(4): 393–402.doi:10.1142/S0218348X01000828.Archived fromthe original(PDF)on 27 October 2011.Retrieved14 April2012.
^Peitgen, Heinz-Otto (2004).Chaos and fractals: new frontiers of science.Springer. pp. 801–803.ISBN 978-0-387-20229-7.
^Ovsienko, V.; Tabachnikov, S. (2004). "Section 1.3".Projective Differential Geometry Old and New: From the Schwarzian Derivative to the Cohomology of Diffeomorphism Groups.Cambridge Tracts in Mathematics. Cambridge University Press.ISBN 978-0-521-83186-4.
^Halliday, David; Resnick, Robert; Walker, Jearl (1997).Fundamentals of Physics(5th ed.). John Wiley & Sons. p. 381.ISBN 0-471-14854-7.
^Urone, Paul Peter; Hinrichs, Roger (2022)."29.7 Probability: The Heisenberg Uncertainty Principle".College Physics 2e.OpenStax.
^Itzykson, C.;Zuber, J.-B.(1980).Quantum Field Theory(2005 ed.). Mineola, NY: Dover Publications.ISBN 978-0-486-44568-7.LCCN 2005053026.OCLC 61200849.
^Low, Peter (1971).Classical Theory of Structures Based on the Differential Equation.Cambridge University Press. pp. 116–118.ISBN 978-0-521-08089-7.
^Batchelor, G. K. (1967).An Introduction to Fluid Dynamics.Cambridge University Press. p. 233.ISBN 0-521-66396-2.
^^a ^b ^cArndt & Haenel 2006,pp. 44–45
^"Most Pi Places Memorized"Archived14 February 2016 at theWayback Machine,Guinness World Records.
^Otake, Tomoko (17 December 2006)."How can anyone remember 100,000 numbers?".The Japan Times.Archivedfrom the original on 18 August 2013.Retrieved27 October2007.
^Danesi, Marcel (January 2021). "Chapter 4: Pi in Popular Culture".Pi ( $π$ ) in Nature, Art, and Culture.Brill. p.97.doi:10.1163/9789004433397.ISBN 9789004433373.S2CID 224869535.
^Raz, A.; Packard, M.G. (2009)."A slice of pi: An exploratory neuroimaging study of digit encoding and retrieval in a superior memorist".Neurocase.15(5): 361–372.doi:10.1080/13554790902776896.PMC4323087.PMID 19585350.
^Keith, Mike."Cadaeic Cadenza Notes & Commentary".Archivedfrom the original on 18 January 2009.Retrieved29 July2009.
^Keith, Michael; Diana Keith (17 February 2010).Not A Wake: A dream embodying (pi)'s digits fully for 10,000 decimals.Vinculum Press.ISBN 978-0-9630097-1-5.
^For instance, Pickover calls π "the most famous mathematical constant of all time", and Peterson writes, "Of all known mathematical constants, however, pi continues to attract the most attention", citing theGivenchyπ perfume,Pi (film),andPi Dayas examples. See:Pickover, Clifford A.(1995).Keys to Infinity.Wiley & Sons. p.59.ISBN 978-0-471-11857-2.Peterson, Ivars(2002).Mathematical Treks: From Surreal Numbers to Magic Circles.MAA spectrum. Mathematical Association of America. p. 17.ISBN 978-0-88385-537-9.Archivedfrom the original on 29 November 2016.
^Posamentier & Lehmann 2004,p. 118
Arndt & Haenel 2006,p. 50
^Arndt & Haenel 2006,p. 14
^Polster, Burkard;Ross, Marty (2012).Math Goes to the Movies.Johns Hopkins University Press. pp. 56–57.ISBN 978-1-421-40484-4.
^Gill, Andy (4 November 2005)."Review of Aerial".The Independent.Archivedfrom the original on 15 October 2006.the almost autistic satisfaction of the obsessive-compulsive mathematician fascinated by 'Pi' (which affords the opportunity to hear Bush slowly sing vast chunks of the number in question, several dozen digits long)
^Rubillo, James M. (January 1989). "Disintegrate 'em".The Mathematics Teacher.82(1): 10.JSTOR 27966082.
^Petroski, Henry(2011).Title An Engineer's Alphabet: Gleanings from the Softer Side of a Profession.Cambridge University Press. p. 47.ISBN 978-1-139-50530-7.
^"Happy Pi Day! Watch these stunning videos of kids reciting 3.14".USAToday.14 March 2015.Archivedfrom the original on 15 March 2015.Retrieved14 March2015.
^Rosenthal, Jeffrey S. (February 2015)."Pi Instant".Math Horizons.22(3): 22.doi:10.4169/mathhorizons.22.3.22.S2CID 218542599.
^Griffin, Andrew."Pi Day: Why some mathematicians refuse to celebrate 14 March and won't observe the dessert-filled day".The Independent.Archivedfrom the original on 24 April 2019.Retrieved2 February2019.
^Freiberger, Marianne; Thomas, Rachel (2015)."Tau – the new $π$ ".Numericon: A Journey through the Hidden Lives of Numbers.Quercus. p. 159.ISBN 978-1-62365-411-5.
^Abbott, Stephen (April 2012)."My Conversion to Tauism"(PDF).Math Horizons.19(4): 34.doi:10.4169/mathhorizons.19.4.34.S2CID 126179022.Archived(PDF)from the original on 28 September 2013.
^Palais, Robert (2001)." $π$ Is Wrong! "(PDF).The Mathematical Intelligencer.23(3): 7–8.doi:10.1007/BF03026846.S2CID 120965049.Archived(PDF)from the original on 22 June 2012.
^"Life of pi in no danger – Experts cold-shoulder campaign to replace with tau".Telegraph India.30 June 2011. Archived fromthe originalon 13 July 2013.
^"Forget Pi Day. We should be celebrating Tau Day | Science News".Retrieved2 May2023.
^Arndt & Haenel 2006,pp. 211–212
Posamentier & Lehmann 2004,pp. 36–37
Hallerberg, Arthur (May 1977). "Indiana's squared circle".Mathematics Magazine.50(3): 136–140.doi:10.2307/2689499.JSTOR 2689499.
^Knuth, Donald(3 October 1990)."The Future of TeX and Metafont"(PDF).TeX Mag.5(1): 145.Archived(PDF)from the original on 13 April 2016.Retrieved17 February2017.
^"PEP 628 – Add math.tau".
^"Crate tau".Retrieved6 December2022.

General and cited sources

Abramson, Jay (2014).Precalculus.OpenStax.
Andrews, George E.; Askey, Richard; Roy, Ranjan (1999).Special Functions.Cambridge: University Press.ISBN 978-0-521-78988-2.
Arndt, Jörg; Haenel, Christoph (2006).Pi Unleashed.Springer-Verlag.ISBN 978-3-540-66572-4.Retrieved5 June2013.English translation by Catriona and David Lischka.
Berggren, Lennart;Borwein, Jonathan;Borwein, Peter(1997).Pi: a Source Book.Springer-Verlag.ISBN 978-0-387-20571-7.
Boyer, Carl B.;Merzbach, Uta C.(1991).A History of Mathematics(2 ed.). Wiley.ISBN 978-0-471-54397-8.
Bronshteĭn, Ilia; Semendiaev, K.A. (1971).A Guide Book to Mathematics.Verlag Harri Deutsch.ISBN 978-3-87144-095-3.
Dym, H.; McKean, H. P. (1972).Fourier series and integrals.Academic Press.
Eymard, Pierre; Lafon, Jean Pierre (2004).The Number $π$ .Translated by Wilson, Stephen. American Mathematical Society.ISBN 978-0-8218-3246-2.English translation ofAutour du nombre $π$ (in French). Hermann. 1999.
Posamentier, Alfred S.; Lehmann, Ingmar (2004). $π$ :A Biography of the World's Most Mysterious Number.Prometheus Books.ISBN 978-1-59102-200-8.
Remmert, Reinhold (2012)."Ch. 5 What is π?".In Heinz-Dieter Ebbinghaus; Hans Hermes; Friedrich Hirzebruch; Max Koecher; Klaus Mainzer; Jürgen Neukirch; Alexander Prestel; Reinhold Remmert (eds.).Numbers.Springer.ISBN 978-1-4612-1005-4.

External links

Weisstein, Eric W."Pi".MathWorld.
Demonstration by Lambert (1761) of irrationality of $π$ ,online Archived31 December 2014 at theWayback Machineand analysedBibNum Archived2 April 2015 at theWayback Machine(PDF).
$π$ Search Engine2 billion searchable digits of $π$ , $e$ and√2
approximation von π by lattice pointsandapproximation of π with rectangles and trapezoids(interactive illustrations)

[1] In particular, $π$ is conjectured to be anormal number,which implies a specific kind of statistical randomness on its digits in all bases.

[14] The precise integral that Weierstrass used was $\pi =\int _{-\infty }^{\infty }{\frac {dx}{1+x^{2}}}.$ Remmert 2012,p. 148

[28] The polynomial shown is the first few terms of theTaylor seriesexpansion of thesinefunction.

[FOOTNOTEAndrewsAskeyRoy199959-2] Andrews, Askey & Roy 1999,p. 59.

[3] Gupta, R. C. (1992). "On the remainder term in the Madhava–Leibniz's series".Ganita Bharati.14(1–4): 68–71.

[jones-4] Jones, William(1706).Synopsis Palmariorum Matheseos.London: J. Wale. pp.243,263.p. 263:There are various other ways of finding theLengths,orAreasof particularCurve LinesorPlanes,which may very much facilitate the Practice; as for instance, in theCircle,the Diameter is to Circumference as 1 to
${\overline {{\tfrac {16}{5}}-{\tfrac {4}{239}}}}-{\tfrac {1}{3}}{\overline {{\tfrac {16}{5^{3}}}-{\tfrac {4}{239^{3}}}}}+{\tfrac {1}{5}}{\overline {{\tfrac {16}{5^{5}}}-{\tfrac {4}{239^{5}}}}}-,\,\&c.=$
$3.14159, & c. = π$ .ThisSeries(among others for the same purpose, and drawn from the same Principle) I receiv'd from the Excellent Analyst, and my much Esteem'd Friend Mr.John Machin;and by means thereof,Van Ceulen's Number, or that in Art. 64.38. may be Examin'd with all desireable Ease and Dispatch.
Reprinted inSmith, David Eugene (1929)."William Jones: The First Use of $π$ for the Circle Ratio ".A Source Book in Mathematics.McGraw–Hill. pp. 346–347.

[5] "π^etrillion digits of π ".pi2e.ch.Archivedfrom the original on 6 December 2016.

[6] Haruka Iwao, Emma(14 March 2019)."Pi in the sky: Calculating a record-breaking 31.4 trillion digits of Archimedes' constant on Google Cloud".Google Cloud Platform.Archivedfrom the original on 19 October 2019.Retrieved12 April2019.

[FOOTNOTEArndtHaenel200617-7] Arndt & Haenel 2006,p. 17.

[8] Bailey, David H.; Plouffe, Simon M.; Borwein, Peter B.; Borwein, Jonathan M. (1997). "The quest for PI".The Mathematical Intelligencer.19(1): 50–56.CiteSeerX10.1.1.138.7085.doi:10.1007/BF03024340.ISSN 0343-6993.S2CID 14318695.

[firstPi-9] Oughtred, William (1652).Theorematum in libris Archimedis de sphaera et cylindro declarario(in Latin). Excudebat L. Lichfield, Veneunt apud T. Robinson. $δ . π$ :: semidiameter. semiperipheria

[10] "pi".Dictionary.reference. 2 March 1993.Archivedfrom the original on 28 July 2014.Retrieved18 June2012.

[FOOTNOTEArndtHaenel20068-11] Arndt & Haenel 2006,p. 8.

[12] Apostol, Tom(1967).Calculus.Vol. 1 (2nd ed.). Wiley. p. 102.From a logical point of view, this is unsatisfactory at the present stage because we have not yet discussed the concept of arc length

[FOOTNOTERemmert2012129-13] Remmert 2012,p. 129.

[15] Baltzer, Richard (1870).Die Elemente der Mathematik[The Elements of Mathematics] (in German). Hirzel. p. 195.Archivedfrom the original on 14 September 2016.

[16] Landau, Edmund(1934).Einführung in die Differentialrechnung und Integralrechnung(in German). Noordoff. p. 193.

[Rudin_1976-17] Rudin, Walter (1976).Principles of Mathematical Analysis.McGraw-Hill. p. 183.ISBN 978-0-07-054235-8.

[18] Rudin, Walter (1986).Real and complex analysis.McGraw-Hill. p. 2.

[19] Ahlfors, Lars(1966).Complex analysis.McGraw-Hill. p. 46.

[20] Bourbaki, Nicolas(1981).Topologie generale.Springer. §VIII.2.

[Nicolas_Bourbaki-21] Bourbaki, Nicolas(1979).Fonctions d'une variable réelle(in French). Springer. §II.3.

[FOOTNOTEArndtHaenel20065-22] Arndt & Haenel 2006,p. 5.

[23] Salikhov, V. (2008). "On the Irrationality Measure of pi".Russian Mathematical Surveys.53(3): 570–572.Bibcode:2008RuMaS..63..570S.doi:10.1070/RM2008v063n03ABEH004543.ISSN 0036-0279.S2CID 250798202.

[FOOTNOTEArndtHaenel200622–23-24] Arndt & Haenel 2006,pp. 22–23.

[FOOTNOTEArndtHaenel200622,_28–30-25] Arndt & Haenel 2006,pp. 22, 28–30.

[FOOTNOTEArndtHaenel20063-26] Arndt & Haenel 2006,p. 3.

[FOOTNOTEArndtHaenel20066-27] Arndt & Haenel 2006,p. 6.

[29] Posamentier & Lehmann 2004,p. 25

[30] Eymard & Lafon 2004,p. 129

[31] Beckmann, Peter (1989) [1974].History of Pi.St. Martin's Press. p. 37.ISBN 978-0-88029-418-8.

[32] Schlager, Neil; Lauer, Josh (2001).Science and Its Times: Understanding the Social Significance of Scientific Discovery.Gale Group.ISBN 978-0-7876-3933-4.Archivedfrom the original on 13 December 2019.Retrieved19 December2019.,p. 185.

[Eymard_1999_78-33] Eymard & Lafon 2004,p. 78

[FOOTNOTEArndtHaenel200633-34] Arndt & Haenel 2006,p. 33.

[mollin-35] Mollin, R. A. (1999). "Continued fraction gems".Nieuw Archief voor Wiskunde.17(3): 383–405.MR 1743850.

[36] Lange, L.J. (May 1999). "An Elegant Continued Fraction for $π$ ".The American Mathematical Monthly.106(5): 456–458.doi:10.2307/2589152.JSTOR 2589152.

[FOOTNOTEArndtHaenel2006240-37] Arndt & Haenel 2006,p. 240.

[FOOTNOTEArndtHaenel2006242-38] Arndt & Haenel 2006,p. 242.

[39] Kennedy, E.S. (1978). "Abu-r-Raihan al-Biruni, 973–1048".Journal for the History of Astronomy.9:65.Bibcode:1978JHA.....9...65K.doi:10.1177/002182867800900106.S2CID 126383231.Ptolemyused a three-sexagesimal-digit approximation, andJamshīd al-Kāshīexpanded this to nine digits; seeAaboe, Asger(1964).Episodes from the Early History of Mathematics.New Mathematical Library. Vol. 13. New York: Random House. p. 125.ISBN 978-0-88385-613-0.Archivedfrom the original on 29 November 2016.

[FOOTNOTEAbramson2014[httpsopenstaxorgbooksprecalculuspages8-5-polar-form-of-complex-numbers_Section_8.5:_Polar_form_of_complex_numbers]-40] Abramson 2014,Section 8.5: Polar form of complex numbers.

[EF-41] Bronshteĭn & Semendiaev 1971,p. 592

[42] Maor, Eli (2009).E: The Story of a Number.Princeton University Press. p. 160.ISBN 978-0-691-14134-3.

[FOOTNOTEAndrewsAskeyRoy199914-43] Andrews, Askey & Roy 1999,p. 14.

[FOOTNOTEArndtHaenel2006167-44] Arndt & Haenel 2006,p. 167.

[45] Herz-Fischler, Roger (2000).The Shape of the Great Pyramid.Wilfrid Laurier University Press. pp. 67–77, 165–166.ISBN 978-0-88920-324-2.Archivedfrom the original on 29 November 2016.Retrieved5 June2013.

[46] Plofker, Kim (2009).Mathematics in India.Princeton University Press. p.27.ISBN 978-0691120676.

[FOOTNOTEArndtHaenel2006170-47] Arndt & Haenel 2006,p. 170.

[FOOTNOTEArndtHaenel2006175,_205-48] Arndt & Haenel 2006,pp. 175, 205.

[life-of-pi-49] Borwein, Jonathan M.(2014). "The life of $π$ :from Archimedes to ENIAC and beyond ". In Sidoli, Nathan; Van Brummelen, Glen (eds.).From Alexandria, through Baghdad: Surveys and studies in the ancient Greek and medieval Islamic mathematical sciences in honor of J. L. Berggren.Heidelberg: Springer. pp. 531–561.doi:10.1007/978-3-642-36736-6_24.ISBN 978-3-642-36735-9.MR 3203895.

[FOOTNOTEArndtHaenel2006171-50] Arndt & Haenel 2006,p. 171.

[FOOTNOTEArndtHaenel2006176-51] Arndt & Haenel 2006,p. 176.

[FOOTNOTEBoyerMerzbach1991168-52] Boyer & Merzbach 1991,p. 168.

[ArPI-53] Arndt & Haenel 2006,pp. 15–16, 175, 184–186, 205. Grienberger achieved 39 digits in 1630; Sharp 71 digits in 1699.

[FOOTNOTEArndtHaenel2006176–177-54] Arndt & Haenel 2006,pp. 176–177.

[autogenerated202-55] Boyer & Merzbach 1991,p. 202

[FOOTNOTEArndtHaenel2006177-56] Arndt & Haenel 2006,p. 177.

[FOOTNOTEArndtHaenel2006178-57] Arndt & Haenel 2006,p. 178.

[FOOTNOTEArndtHaenel2006179-58] Arndt & Haenel 2006,p. 179.

[FOOTNOTEArndtHaenel2006180-59] Arndt & Haenel 2006,p. 180.

[60] Azarian, Mohammad K. (2010)."al-Risāla al-muhītīyya: A Summary".Missouri Journal of Mathematical Sciences.22(2): 64–85.doi:10.35834/mjms/1312233136.

[61] O'Connor, John J.; Robertson, Edmund F. (1999)."Ghiyath al-Din Jamshid Mas'ud al-Kashi".MacTutor History of Mathematics archive.Archivedfrom the original on 12 April 2011.Retrieved11 August2012.

[FOOTNOTEArndtHaenel2006182-62] Arndt & Haenel 2006,p. 182.

[FOOTNOTEArndtHaenel2006182–183-63] Arndt & Haenel 2006,pp. 182–183.

[FOOTNOTEArndtHaenel2006183-64] Arndt & Haenel 2006,p. 183.

[65] Grienbergerus, Christophorus(1630).Elementa Trigonometrica(PDF)(in Latin). Archived fromthe original(PDF)on 1 February 2014.His evaluation was 3.14159 26535 89793 23846 26433 83279 50288 4196 < $π$ < 3.14159 26535 89793 23846 26433 83279 50288 4199.

[66] Brezinski, C. (2009). "Some pioneers of extrapolation methods". InBultheel, Adhemar;Cools, Ronald (eds.).The Birth of Numerical Analysis.World Scientific. pp. 1–22.doi:10.1142/9789812836267_0001.ISBN 978-981-283-625-0.

[67] Yoder, Joella G. (1996)."Following in the footsteps of geometry: The mathematical world of Christiaan Huygens".De Zeventiende Eeuw.12:83–93 – viaDigital Library for Dutch Literature.

[Ais-68] Arndt & Haenel 2006,pp. 185–191

[Roypp-69] Roy, Ranjan (1990)."The Discovery of the Series Formula for $π$ by Leibniz, Gregory and Nilakantha "(PDF).Mathematics Magazine.63(5): 291–306.doi:10.1080/0025570X.1990.11977541.

[FOOTNOTEArndtHaenel2006185–186-70] Arndt & Haenel 2006,pp. 185–186.

[71] Joseph, George Gheverghese (1991).The Crest of the Peacock: Non-European Roots of Mathematics.Princeton University Press. p. 264.ISBN 978-0-691-13526-7.

[FOOTNOTEArndtHaenel2006187-72] Arndt & Haenel 2006,p. 187.

[73] OEIS:A060294

[74] Vieta, Franciscus (1593).Variorum de rebus mathematicis responsorum.Vol. VIII.

[Newton-75] Arndt & Haenel 2006,p. 188. Newton quoted by Arndt.

[76] Horvath, Miklos (1983)."On the Leibnizian quadrature of the circle"(PDF).Annales Universitatis Scientiarum Budapestiensis (Sectio Computatorica).4:75–83.

[LS-77] Eymard & Lafon 2004,pp. 53–54

[78] Cooker, M.J. (2011)."Fast formulas for slowly convergent alternating series"(PDF).Mathematical Gazette.95(533): 218–226.doi:10.1017/S0025557200002928.S2CID 123392772.Archived from the original on 4 May 2019.Retrieved23 February2023.{{cite journal}}:CS1 maint: bot: original URL status unknown (link)

[FOOTNOTEArndtHaenel2006189-79] Arndt & Haenel 2006,p. 189.

[tweddle-80] Tweddle, Ian (1991). "John Machin and Robert Simson on Inverse-tangent Series for $π$ ".Archive for History of Exact Sciences.42(1): 1–14.doi:10.1007/BF00384331.JSTOR 41133896.S2CID 121087222.

[FOOTNOTEArndtHaenel2006192–193-81] Arndt & Haenel 2006,pp. 192–193.

[A72n4-82] Arndt & Haenel 2006,pp. 72–74

[83] Lehmer, D. H.(1938)."On Arccotangent Relations for $π$ "(PDF).American Mathematical Monthly.45(10): 657–664 Published by: Mathematical Association of America.doi:10.1080/00029890.1938.11990873.JSTOR 2302434.

[84] Roy, Ranjan (2021) [1st ed. 2011].Series and Products in the Development of Mathematics.Vol. 1 (2 ed.). Cambridge University Press. pp. 215–216, 219–220.
Newton, Isaac(1971).Whiteside, Derek Thomas(ed.).The Mathematical Papers of Isaac Newton.Vol. 4, 1674–1684. Cambridge University Press. pp. 526–653.

[85] Sandifer, Ed (2009)."Estimating π"(PDF).How Euler Did It.Reprinted inHow Euler Did Even More.Mathematical Association of America. 2014. pp. 109–118.
Euler, Leonhard(1755)."§2.2.30".Institutiones Calculi Differentialis(in Latin). Academiae Imperialis Scientiarium Petropolitanae. p. 318.E 212.

Euler, Leonhard(1798) [written 1779]."Investigatio quarundam serierum, quae ad rationem peripheriae circuli ad diametrum vero proxime definiendam maxime sunt accommodatae".Nova Acta Academiae Scientiarum Petropolitinae.11:133–149, 167–168.E 705.

Chien-Lih, Hwang (2004). "88.38 Some Observations on the Method of Arctangents for the Calculation of $π$ ".Mathematical Gazette.88(512): 270–278.doi:10.1017/S0025557200175060.S2CID 123532808.

Chien-Lih, Hwang (2005). "89.67 An elementary derivation of Euler's series for the arctangent function".Mathematical Gazette.89(516): 469–470.doi:10.1017/S0025557200178404.S2CID 123395287.

[FOOTNOTEArndtHaenel2006192–196,_205-86] Arndt & Haenel 2006,pp. 192–196, 205.

[A194-87] Arndt & Haenel 2006,pp. 194–196

[hayes-2014-88] Hayes, Brian (September 2014)."Pencil, Paper, and Pi".American Scientist.Vol. 102, no. 5. p. 342.doi:10.1511/2014.110.342.Retrieved22 January2022.

[Aconverge-89] Borwein, J.M.; Borwein, P.B. (1988). "Ramanujan and Pi".Scientific American.256(2): 112–117.Bibcode:1988SciAm.258b.112B.doi:10.1038/scientificamerican0288-112.
Arndt & Haenel 2006,pp. 15–17, 70–72, 104, 156, 192–197, 201–202

[FOOTNOTEArndtHaenel200669–72-90] Arndt & Haenel 2006,pp. 69–72.

[91] Borwein, J.M.; Borwein, P.B.; Dilcher, K. (1989). "Pi, Euler Numbers, and Asymptotic Expansions".American Mathematical Monthly.96(8): 681–687.doi:10.2307/2324715.hdl:1959.13/1043679.JSTOR 2324715.

[FOOTNOTEArndtHaenel2006Formula_16.10,_p._223-92] Arndt & Haenel 2006,Formula 16.10, p. 223.

[93] Wells, David (1997).The Penguin Dictionary of Curious and Interesting Numbers(revised ed.). Penguin. p. 35.ISBN 978-0-14-026149-3.

[Posamentier-94] Posamentier & Lehmann 2004,p. 284

[95] Lambert, Johann, "Mémoire sur quelques propriétés remarquables des quantités transcendantes circulaires et logarithmiques", reprinted inBerggren, Borwein & Borwein 1997,pp. 129–140

[96] Lindemann, F.(1882)."Über die Ludolph'sche Zahl".Sitzungsberichte der Königlich Preussischen Akademie der Wissenschaften zu Berlin.2:679–682.

[FOOTNOTEArndtHaenel2006196-97] Arndt & Haenel 2006,p. 196.

[98] Hardy and Wright 1938 and 2000: 177 footnote § 11.13–14 references Lindemann's proof as appearing atMath. Ann.20 (1882), 213–225.

[99] Hardy and Wright 1938 and 2000:177 footnote § 11.13–14. The proofs that e and π are transcendental can be found on pp. 170–176. They cite two sources of the proofs at Landau 1927 or Perron 1910; see the "List of Books" at pp. 417–419 for full citations.

[Cajori-2007-100] Cajori, Florian (2007).A History of Mathematical Notations: Vol. II.Cosimo, Inc. pp. 8–13.ISBN 978-1-60206-714-1.the ratio of the length of a circle to its diameter was represented in the fractional form by the use of two letters... J.A. Segner... in 1767, he represented $3.14159...$ by $δ : π$ ,as did Oughtred more than a century earlier

[101] Schepler, H.C. (1950) "The Chronology of Pi"Mathematics Magazine.23.
Part 1. Jan/Feb. (3): 165–170.doi:10.2307/3029284.
Part 2. Mar/Apr. (4): 216-228.doi:10.2307/3029832.
Part 3. May/Jun. (5): 279-283.doi:10.2307/3029000.
See p. 220:William Oughtredused the letter $π$ to represent the periphery (that is, the circumference) of a circle.

[Smith-1958-102] Smith, David E. (1958).History of Mathematics.Courier Corporation. p. 312.ISBN 978-0-486-20430-7.

[103] Archibald, R.C. (1921). "Historical Notes on the Relation $e -(π /2) = i i$ ".The American Mathematical Monthly.28(3): 116–121.doi:10.2307/2972388.JSTOR 2972388.It is noticeable that these letters areneverused separately, that is, $π$ isnotused for 'Semiperipheria'

[FOOTNOTEArndtHaenel2006166-104] Arndt & Haenel 2006,p. 166.

[105] See, for example,Oughtred, William (1648).Clavis Mathematicæ[The key to mathematics] (in Latin). London: Thomas Harper. p.69.(English translation:Oughtred, William (1694).Key of the Mathematics.J. Salusbury.)

[106] Barrow, Isaac (1860)."Lecture XXIV".In Whewell, William (ed.).The mathematical works of Isaac Barrow(in Latin). Harvard University. Cambridge University press. p. 381.

[107] Gregorius, David (1695)."Ad Reverendum Virum D. Henricum Aldrich S.T.T. Decanum Aedis Christi Oxoniae"(PDF).Philosophical Transactions(in Latin).19(231): 637–652.Bibcode:1695RSPT...19..637G.doi:10.1098/rstl.1695.0114.JSTOR 102382.

[FOOTNOTEArndtHaenel2006165-108] Arndt & Haenel 2006,p. 165: A facsimile of Jones' text is inBerggren, Borwein & Borwein 1997,pp. 108–109.

[109] Segner, Joannes Andreas (1756).Cursus Mathematicus(in Latin). Halae Magdeburgicae. p. 282.Archivedfrom the original on 15 October 2017.Retrieved15 October2017.

[110] Euler, Leonhard (1727)."Tentamen explicationis phaenomenorum aeris"(PDF).Commentarii Academiae Scientiarum Imperialis Petropolitana(in Latin).2:351.E007.Archived(PDF)from the original on 1 April 2016.Retrieved15 October2017.Sumatur pro ratione radii ad peripheriem, $I: π$ English translation by Ian Bruce Archived10 June 2016 at theWayback Machine:" $π$ is taken for the ratio of the radius to the periphery [note that in this work, Euler's $π$ is double our $π$ .] "

[111] Euler, Leonhard (1747). Henry, Charles (ed.).Lettres inédites d'Euler à d'Alembert.Bullettino di Bibliografia e di Storia delle Scienze Matematiche e Fisiche (in French). Vol. 19 (published 1886). p. 139.E858.Car, soit π la circonference d'un cercle, dout le rayon est $= 1$ English translation inCajori, Florian (1913). "History of the Exponential and Logarithmic Concepts".The American Mathematical Monthly.20(3): 75–84.doi:10.2307/2973441.JSTOR 2973441.Letting $π$ be the circumference (!) of a circle of unit radius

[112] Euler, Leonhard (1736)."Ch. 3 Prop. 34 Cor. 1".Mechanica sive motus scientia analytice exposita. (cum tabulis)(in Latin). Vol. 1. Academiae scientiarum Petropoli. p. 113.E015.Denotet $1: π$ rationem diametri ad peripheriamEnglish translation by Ian Bruce Archived10 June 2016 at theWayback Machine:"Let $1: π$ denote the ratio of the diameter to the circumference "

[113] Euler, Leonhard (1707–1783) (1922).Leonhardi Euleri opera omnia. 1, Opera mathematica. Volumen VIII, Leonhardi Euleri introductio in analysin infinitorum. Tomus primus / ediderunt Adolf Krazer et Ferdinand Rudio(in Latin). Lipsae: B.G. Teubneri. pp. 133–134.E101.Archivedfrom the original on 16 October 2017.Retrieved15 October2017.{{cite book}}:CS1 maint: numeric names: authors list (link)

[114] Segner, Johann Andreas von (1761).Cursus Mathematicus: Elementorum Analyseos Infinitorum Elementorum Analyseos Infinitorvm(in Latin). Renger. p. 374.Si autem $π$ notet peripheriam circuli, cuius diameter eſt $2$

[FOOTNOTEArndtHaenel2006205-115] Arndt & Haenel 2006,p. 205.

[FOOTNOTEArndtHaenel2006197-116] Arndt & Haenel 2006,p. 197.

[117] Reitwiesner, George (1950). "An ENIAC Determination of pi and e to 2000 Decimal Places".Mathematical Tables and Other Aids to Computation.4(29): 11–15.doi:10.2307/2002695.JSTOR 2002695.

[118] Nicholson, J. C.; Jeenel, J. (1955). "Some comments on a NORC Computation of π".Math. Tabl. Aids. Comp.9(52): 162–164.doi:10.2307/2002052.JSTOR 2002052.

[FOOTNOTEArndtHaenel200615–17-119] Arndt & Haenel 2006,pp. 15–17.

[FOOTNOTEArndtHaenel2006131-120] Arndt & Haenel 2006,p. 131.

[FOOTNOTEArndtHaenel2006132,_140-121] Arndt & Haenel 2006,pp. 132, 140.

[FOOTNOTEArndtHaenel200687-122] Arndt & Haenel 2006,p. 87.

[123] Arndt & Haenel 2006,pp. 111 (5 times), pp. 113–114 (4 times). For details of algorithms, seeBorwein, Jonathan; Borwein, Peter (1987).Pi and the AGM: a Study in Analytic Number Theory and Computational Complexity.Wiley.ISBN 978-0-471-31515-5.

[Background-124] Bailey, David H. (16 May 2003)."Some Background on Kanada's Recent Pi Calculation"(PDF).Archived(PDF)from the original on 15 April 2012.Retrieved12 April2012.

[125] Arndt & Haenel 2006,pp. 17–19

[msnbc.msn.com-126] Schudel, Matt (25 March 2009). "John W. Wrench, Jr.: Mathematician Had a Taste for Pi".The Washington Post.p. B5.

[independent.co.uk-127] Connor, Steve (8 January 2010)."The Big Question: How close have we come to knowing the precise value of pi?".The Independent.London.Archivedfrom the original on 2 April 2012.Retrieved14 April2012.

[FOOTNOTEArndtHaenel200618-128] Arndt & Haenel 2006,p. 18.

[129] Arndt & Haenel 2006,pp. 103–104

[130] Arndt & Haenel 2006,p. 104

[131] Arndt & Haenel 2006,pp. 104, 206

[132] Arndt & Haenel 2006,pp. 110–111

[133] Eymard & Lafon 2004,p. 254

[NW-134] Bailey, David H.;Borwein, Jonathan M.(2016)."15.2 Computational records".Pi: The Next Generation, A Sourcebook on the Recent History of Pi and Its Computation.Springer International Publishing. p. 469.doi:10.1007/978-3-319-32377-0.ISBN 978-3-319-32375-6.

[135] Cassel, David (11 June 2022)."How Google's Emma Haruka Iwao Helped Set a New Record for Pi".The New Stack.

[136] PSLQ means Partial Sum of Least Squares.

[137] Plouffe, Simon(April 2006)."Identities inspired by Ramanujan's Notebooks (part 2)"(PDF).Archived(PDF)from the original on 14 January 2012.Retrieved10 April2009.

[138] Arndt & Haenel 2006,p. 39

[bn-139] Ramaley, J.F. (October 1969). "Buffon's Noodle Problem".The American Mathematical Monthly.76(8): 916–918.doi:10.2307/2317945.JSTOR 2317945.

[140] Arndt & Haenel 2006,pp. 39–40
Posamentier & Lehmann 2004,p. 105

[141] Grünbaum, B.(1960)."Projection Constants".Transactions of the American Mathematical Society.95(3): 451–465.doi:10.1090/s0002-9947-1960-0114110-9.

[142] Arndt & Haenel 2006,pp. 43
Posamentier & Lehmann 2004,pp. 105–108

[FOOTNOTEArndtHaenel200677–84-143] Arndt & Haenel 2006,pp. 77–84.

[Gibbons-144] Gibbons, Jeremy(2006)."Unbounded spigot algorithms for the digits of pi"(PDF).The American Mathematical Monthly.113(4): 318–328.doi:10.2307/27641917.JSTOR 27641917.MR 2211758.

[FOOTNOTEArndtHaenel200677-145] Arndt & Haenel 2006,p. 77.

[146] Rabinowitz, Stanley; Wagon, Stan (March 1995). "A spigot algorithm for the digits of Pi".American Mathematical Monthly.102(3): 195–203.doi:10.2307/2975006.JSTOR 2975006.

[FOOTNOTEArndtHaenel2006117,_126–128-147] Arndt & Haenel 2006,pp. 117, 126–128.

[bbpf-148] Bailey, David H.;Borwein, Peter B.;Plouffe, Simon(April 1997)."On the Rapid Computation of Various Polylogarithmic Constants"(PDF).Mathematics of Computation.66(218): 903–913.Bibcode:1997MaCom..66..903B.CiteSeerX10.1.1.55.3762.doi:10.1090/S0025-5718-97-00856-9.S2CID 6109631.Archived(PDF)from the original on 22 July 2012.

[149] Arndt & Haenel 2006,p. 20
Bellards formula in:Bellard, Fabrice."A new formula to compute the n^thbinary digit of pi ".Archived fromthe originalon 12 September 2007.Retrieved27 October2007.

[150] Palmer, Jason (16 September 2010)."Pi record smashed as team finds two-quadrillionth digit".BBC News.Archivedfrom the original on 17 March 2011.Retrieved26 March2011.

[151] Plouffe, Simon (2022). "A formula for the $n$ th decimal digit or binary of $π$ and powers of $π$ ".arXiv:2201.12601[math.NT].

[152] Bronshteĭn & Semendiaev 1971,pp. 200, 209

[153] Martini, Horst; Montejano, Luis;Oliveros, Déborah(2019).Bodies of Constant Width: An Introduction to Convex Geometry with Applications.Birkhäuser.doi:10.1007/978-3-030-03868-7.ISBN 978-3-030-03866-3.MR 3930585.S2CID 127264210.

See Barbier's theorem, Corollary 5.1.1, p. 98; Reuleaux triangles, pp. 3, 10; smooth curves such as an analytic curve due to Rabinowitz, § 5.3.3, pp. 111–112.

[154] Herman, Edwin;Strang, Gilbert(2016)."Section 5.5, Exercise 316".Calculus.Vol. 1.OpenStax.p. 594.

[FOOTNOTEAbramson2014[httpsopenstaxorgbooksprecalculuspages5-1-angles_Section_5.1:_Angles]-155] Abramson 2014,Section 5.1: Angles.

[WCS-156] Bronshteĭn & Semendiaev 1971,pp. 210–211

[157] Hilbert, David;Courant, Richard(1966).Methods of mathematical physics, volume 1.Wiley. pp. 286–290.

[FOOTNOTEDymMcKean197247-158] Dym & McKean 1972,p. 47.

[159] Thompson, William(1894). "Isoperimetrical problems".Nature Series: Popular Lectures and Addresses.II:571–592.

[160] Chavel, Isaac (2001).Isoperimetric inequalities.Cambridge University Press.

[161] Talenti, Giorgio (1976). "Best constant in Sobolev inequality".Annali di Matematica Pura ed Applicata.110(1): 353–372.CiteSeerX10.1.1.615.4193.doi:10.1007/BF02418013.ISSN 1618-1891.S2CID 16923822.

[162] L. Esposito; C. Nitsch; C. Trombetti (2011). "Best constants in Poincaré inequalities for convex domains".arXiv:1110.2960[math.AP].

[163] Del Pino, M.; Dolbeault, J. (2002). "Best constants for Gagliardo–Nirenberg inequalities and applications to nonlinear diffusions".Journal de Mathématiques Pures et Appliquées.81(9): 847–875.CiteSeerX10.1.1.57.7077.doi:10.1016/s0021-7824(02)01266-7.S2CID 8409465.

[164] Payne, L.E.; Weinberger, H.F. (1960). "An optimal Poincaré inequality for convex domains".Archive for Rational Mechanics and Analysis.5(1): 286–292.Bibcode:1960ArRMA...5..286P.doi:10.1007/BF00252910.ISSN 0003-9527.S2CID 121881343.

[165] Folland, Gerald(1989).Harmonic analysis in phase space.Princeton University Press. p. 5.

[howe-166] Howe, Roger (1980)."On the role of the Heisenberg group in harmonic analysis".Bulletin of the American Mathematical Society.3(2): 821–844.doi:10.1090/S0273-0979-1980-14825-9.MR 0578375.

[167] Feller, W.An Introduction to Probability Theory and Its Applications, Vol. 1,Wiley, 1968, pp. 174–190.

[GaussProb-168] Bronshteĭn & Semendiaev 1971,pp. 106–107, 744, 748

[FOOTNOTEDymMcKean1972Section_2.7-169] Dym & McKean 1972,Section 2.7.

[170] Stein, Elias;Weiss, Guido (1971).Fourier analysis on Euclidean spaces.Princeton University Press. p. 6.;Theorem 1.13.

[171] Spivak, Michael(1999).A Comprehensive Introduction to Differential Geometry.Vol. 3. Publish or Perish Press.;Chapter 6.

[172] Kobayashi, Shoshichi; Nomizu, Katsumi (1996).Foundations of Differential Geometry.Vol. 2 (New ed.).Wiley Interscience.p. 293.;Chapter XIICharacteristic classes

[173] Ahlfors, Lars(1966).Complex analysis.McGraw-Hill. p. 115.

[174] Joglekar, S. D. (2005).Mathematical Physics.Universities Press. p. 166.ISBN 978-81-7371-422-1.

[175] Schey, H. M. (1996).Div, Grad, Curl, and All That: An Informal Text on Vector Calculus.W.W. Norton.ISBN 0-393-96997-5.

[176] Yeo, Adrian (2006).The pleasures of pi, e and other interesting numbers.World Scientific Pub. p. 21.ISBN 978-981-270-078-0.

[177] Ehlers, Jürgen (2000).Einstein's Field Equations and Their Physical Implications.Springer. p. 7.ISBN 978-3-540-67073-5.

[Elliptic_PDE2-178] Gilbarg, D.;Trudinger, Neil(1983),Elliptic Partial Differential Equations of Second Order,New York: Springer,ISBN 3-540-41160-7

[179] Bronshteĭn & Semendiaev 1971,pp. 191–192

[180] Artin, Emil(1964).The Gamma Function.Athena series; selected topics in mathematics (1st ed.). Holt, Rinehart and Winston.

[181] Evans, Lawrence (1997).Partial Differential Equations.AMS. p. 615.

[182] Bronshteĭn & Semendiaev 1971,p. 190

[183] Benjamin Nill; Andreas Paffenholz (2014). "On the equality case in Erhart's volume conjecture".Advances in Geometry.14(4): 579–586.arXiv:1205.1270.doi:10.1515/advgeom-2014-0001.ISSN 1615-7168.S2CID 119125713.

[184] Arndt & Haenel 2006,pp. 41–43

[185] This theorem was proved byErnesto Cesàroin 1881. For a more rigorous proof than the intuitive and informal one given here, seeHardy, G. H. (2008).An Introduction to the Theory of Numbers.Oxford University Press. Theorem 332.ISBN 978-0-19-921986-5.

[186] Ogilvy, C. S.;Anderson, J. T. (1988).Excursions in Number Theory.Dover Publications Inc. pp. 29–35.ISBN 0-486-25778-9.

[187] Arndt & Haenel 2006,p. 43

[188] Platonov, Vladimir; Rapinchuk, Andrei (1994).Algebraic Groups and Number Theory.Academic Press. pp. 262–265.

[189] Sondow, J. (1994). "Analytic continuation of Riemann's zeta function and values at negative integers via Euler's transformation of series".Proceedings of the American Mathematical Society.120(2): 421–424.CiteSeerX10.1.1.352.5774.doi:10.1090/s0002-9939-1994-1172954-7.S2CID 122276856.

[190] T. Friedmann; C.R. Hagen (2015). "Quantum mechanical derivation of the Wallis formula for pi".Journal of Mathematical Physics.56(11): 112101.arXiv:1510.07813.Bibcode:2015JMP....56k2101F.doi:10.1063/1.4930800.S2CID 119315853.

[191] Tate, John T. (1950). "Fourier analysis in number fields, and Hecke's zeta-functions". In Cassels, J. W. S.; Fröhlich, A. (eds.).Algebraic Number Theory (Proc. Instructional Conf., Brighton, 1965).Thompson, Washington, DC. pp. 305–347.ISBN 978-0-9502734-2-6.MR 0217026.

[FOOTNOTEDymMcKean1972Chapter_4-192] Dym & McKean 1972,Chapter 4.

[Mumford_1983_1–117-193] Mumford, David(1983).Tata Lectures on Theta I.Boston: Birkhauser. pp. 1–117.ISBN 978-3-7643-3109-2.

[194] Port, Sidney; Stone, Charles (1978).Brownian motion and classical potential theory.Academic Press. p. 29.

[195] Titchmarsh, E.(1948).Introduction to the Theory of Fourier Integrals(2nd ed.). Oxford University: Clarendon Press (published 1986).ISBN 978-0-8284-0324-5.

[196] Stein, Elias(1970).Singular Integrals and Differentiability Properties of Functions.Princeton University Press.;Chapter II.

[KA-197] Klebanoff, Aaron (2001)."Pi in the Mandelbrot set"(PDF).Fractals.9(4): 393–402.doi:10.1142/S0218348X01000828.Archived fromthe original(PDF)on 27 October 2011.Retrieved14 April2012.

[198] Peitgen, Heinz-Otto (2004).Chaos and fractals: new frontiers of science.Springer. pp. 801–803.ISBN 978-0-387-20229-7.

[199] Ovsienko, V.; Tabachnikov, S. (2004). "Section 1.3".Projective Differential Geometry Old and New: From the Schwarzian Derivative to the Cohomology of Diffeomorphism Groups.Cambridge Tracts in Mathematics. Cambridge University Press.ISBN 978-0-521-83186-4.

[200] Halliday, David; Resnick, Robert; Walker, Jearl (1997).Fundamentals of Physics(5th ed.). John Wiley & Sons. p. 381.ISBN 0-471-14854-7.

[201] Urone, Paul Peter; Hinrichs, Roger (2022)."29.7 Probability: The Heisenberg Uncertainty Principle".College Physics 2e.OpenStax.

[202] Itzykson, C.;Zuber, J.-B.(1980).Quantum Field Theory(2005 ed.). Mineola, NY: Dover Publications.ISBN 978-0-486-44568-7.LCCN 2005053026.OCLC 61200849.

[203] Low, Peter (1971).Classical Theory of Structures Based on the Differential Equation.Cambridge University Press. pp. 116–118.ISBN 978-0-521-08089-7.

[204] Batchelor, G. K. (1967).An Introduction to Fluid Dynamics.Cambridge University Press. p. 233.ISBN 0-521-66396-2.

[A445-205] Arndt & Haenel 2006,pp. 44–45

[206] "Most Pi Places Memorized"Archived14 February 2016 at theWayback Machine,Guinness World Records.

[japantimes-207] Otake, Tomoko (17 December 2006)."How can anyone remember 100,000 numbers?".The Japan Times.Archivedfrom the original on 18 August 2013.Retrieved27 October2007.

[208] Danesi, Marcel (January 2021). "Chapter 4: Pi in Popular Culture".Pi ( $π$ ) in Nature, Art, and Culture.Brill. p.97.doi:10.1163/9789004433397.ISBN 9789004433373.S2CID 224869535.

[209] Raz, A.; Packard, M.G. (2009)."A slice of pi: An exploratory neuroimaging study of digit encoding and retrieval in a superior memorist".Neurocase.15(5): 361–372.doi:10.1080/13554790902776896.PMC4323087.PMID 19585350.

[210] Keith, Mike."Cadaeic Cadenza Notes & Commentary".Archivedfrom the original on 18 January 2009.Retrieved29 July2009.

[KeithNAW-211] Keith, Michael; Diana Keith (17 February 2010).Not A Wake: A dream embodying (pi)'s digits fully for 10,000 decimals.Vinculum Press.ISBN 978-0-9630097-1-5.

[212] For instance, Pickover calls π "the most famous mathematical constant of all time", and Peterson writes, "Of all known mathematical constants, however, pi continues to attract the most attention", citing theGivenchyπ perfume,Pi (film),andPi Dayas examples. See:Pickover, Clifford A.(1995).Keys to Infinity.Wiley & Sons. p.59.ISBN 978-0-471-11857-2.Peterson, Ivars(2002).Mathematical Treks: From Surreal Numbers to Magic Circles.MAA spectrum. Mathematical Association of America. p. 17.ISBN 978-0-88385-537-9.Archivedfrom the original on 29 November 2016.

[213] Posamentier & Lehmann 2004,p. 118
Arndt & Haenel 2006,p. 50

[214] Arndt & Haenel 2006,p. 14

[215] Polster, Burkard;Ross, Marty (2012).Math Goes to the Movies.Johns Hopkins University Press. pp. 56–57.ISBN 978-1-421-40484-4.

[216] Gill, Andy (4 November 2005)."Review of Aerial".The Independent.Archivedfrom the original on 15 October 2006.the almost autistic satisfaction of the obsessive-compulsive mathematician fascinated by 'Pi' (which affords the opportunity to hear Bush slowly sing vast chunks of the number in question, several dozen digits long)

[217] Rubillo, James M. (January 1989). "Disintegrate 'em".The Mathematics Teacher.82(1): 10.JSTOR 27966082.

[218] Petroski, Henry(2011).Title An Engineer's Alphabet: Gleanings from the Softer Side of a Profession.Cambridge University Press. p. 47.ISBN 978-1-139-50530-7.

[219] "Happy Pi Day! Watch these stunning videos of kids reciting 3.14".USAToday.14 March 2015.Archivedfrom the original on 15 March 2015.Retrieved14 March2015.

[220] Rosenthal, Jeffrey S. (February 2015)."Pi Instant".Math Horizons.22(3): 22.doi:10.4169/mathhorizons.22.3.22.S2CID 218542599.

[221] Griffin, Andrew."Pi Day: Why some mathematicians refuse to celebrate 14 March and won't observe the dessert-filled day".The Independent.Archivedfrom the original on 24 April 2019.Retrieved2 February2019.

[222] Freiberger, Marianne; Thomas, Rachel (2015)."Tau – the new $π$ ".Numericon: A Journey through the Hidden Lives of Numbers.Quercus. p. 159.ISBN 978-1-62365-411-5.

[223] Abbott, Stephen (April 2012)."My Conversion to Tauism"(PDF).Math Horizons.19(4): 34.doi:10.4169/mathhorizons.19.4.34.S2CID 126179022.Archived(PDF)from the original on 28 September 2013.

[224] Palais, Robert (2001)." $π$ Is Wrong! "(PDF).The Mathematical Intelligencer.23(3): 7–8.doi:10.1007/BF03026846.S2CID 120965049.Archived(PDF)from the original on 22 June 2012.

[225] "Life of pi in no danger – Experts cold-shoulder campaign to replace with tau".Telegraph India.30 June 2011. Archived fromthe originalon 13 July 2013.

[226] "Forget Pi Day. We should be celebrating Tau Day | Science News".Retrieved2 May2023.

[227] Arndt & Haenel 2006,pp. 211–212
Posamentier & Lehmann 2004,pp. 36–37
Hallerberg, Arthur (May 1977). "Indiana's squared circle".Mathematics Magazine.50(3): 136–140.doi:10.2307/2689499.JSTOR 2689499.

[228] Knuth, Donald(3 October 1990)."The Future of TeX and Metafont"(PDF).TeX Mag.5(1): 145.Archived(PDF)from the original on 13 April 2016.Retrieved17 February2017.

[229] "PEP 628 – Add math.tau".

[230] "Crate tau".Retrieved6 December2022.

[a]

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[b]

[13]

[14]

[15]

[16]

[17]

[18]

[19]

[20]

[21]

[22]

[23]

[24]

[25]

[c]

[26]

[27]

[28]

[29]

[30]

[31]

[32]

[33]

[34]

[35]

[36]

[37]

[38]

[39]

[40]

[41]

[42]

[43]

[44]

[45]

[46]

[47]

[48]

[49]

[50]

[51]

[52]

[53]

[54]

[55]

[56]

[57]

[58]

[59]

[60]

[61]

[62]

[63]

[64]

[65]

[66]

[67]

[68]

[69]

[70]

[71]

[72]

[73]

[74]

[75]

[76]

[77]

[78]

[79]

[80]

[81]

[82]

[83]

[84]

[85]

[86]

[87]

[88]

[89]

[90]

[91]

[92]

[93]

[94]

[95]

[96]

[97]