Squircle

Not to be confused withsquared circle.

Squircle centred on the origin (a=b= 0) with minor radiusr= 1:x⁴+y⁴= 1

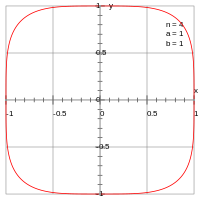
Asquircleis ashapeintermediate between asquareand acircle.There are at least two definitions of "squircle" in use, the most common of which is based on thesuperellipse.The word "squircle" is aportmanteauof the words "square" and "circle". Squircles have been applied indesignandoptics.

Superellipse-based squircle

In aCartesian coordinate system,thesuperellipseis defined by the equation $$\left|{\frac {x-a}{r_{a}}}\right|^{n}+\left|{\frac {y-b}{r_{b}}}\right|^{n}=1,$$ wherer_aandr_bare thesemi-majorandsemi-minoraxes,aandbare thexandycoordinates of the centre of the ellipse, andnis a positive number. The squircle is then defined as the superellipse withr_a=r_bandn= 4.Its equation is: $$\left|x-a\right|^{4}+\left|y-b\right|^{4}=r^{4}$$ whereris the minorradiusof the squircle, and the major radius is the geometric average between square and circle. Compare this to theequation of a circle.When the squircle is centred at the origin, thena=b= 0,and it is calledLamé's special quartic.

Theareainside the squircle can be expressed in terms of thegamma functionΓas $$\mathrm {Area} =4r^{2}{\frac {\left(\operatorname {\Gamma } \left(1+{\frac {1}{4}}\right)\right)^{2}}{\operatorname {\Gamma } \left(1+{\frac {2}{4}}\right)}}={\frac {8r^{2}\left(\operatorname {\Gamma } \left({\frac {5}{4}}\right)\right)^{2}}{\sqrt {\pi }}}=\varpi {\sqrt {2}}\,r^{2}\approx 3.708149\,r^{2},$$ whereris the minor radius of the squircle, and$\varpi$is thelemniscate constant.

p-norm notation

In terms of thep-norm‖ · ‖_ponR²,the squircle can be expressed as: $$\left\|\mathbf {x} -\mathbf {x} _{c}\right\|_{p}=r$$ wherep= 4,x_c= (a,b)is the vector denoting the centre of the squircle, andx= (x,y).Effectively, this is still a "circle" of points at a distancerfrom the centre, but distance is defined differently. For comparison, the usual circle is the casep= 2,whereas the square is given by thep→ ∞case (thesupremum norm), and a rotated square is given byp= 1(thetaxicab norm).This allows a straightforward generalization to aspherical cube,orsphube,inR³,orhypersphubein higher dimensions.

Fernández-Guasti squircle

Another squircle comes from work in optics.It may be called the Fernández-Guasti squircle, after one of its authors, to distinguish it from the superellipse-related squircle above.This kind of squircle, centred at the origin, can be defined by the equation: $$x^{2}+y^{2}-{\frac {s^{2}}{r^{2}}}x^{2}y^{2}=r^{2}$$ whereris the minor radius of the squircle,sis the squareness parameter, andxandyare in theinterval[−r,r].Ifs= 0,the equation is a circle; ifs= 1,this is a square. This equation allows a smoothparametrizationof the transition to a square from a circle, without involvinginfinity.

Periodic squircle

Another type of squircle arises fromtrigonometry.This type of squircle is periodic inR²and has the equation

$$\cos \left({\frac {s\pi x}{2r}}\right)\cos \left({\frac {s\pi y}{2r}}\right)=\cos \left({\frac {s\pi }{2}}\right)$$

whereris the minor radius of the squircle,sis the squareness parameter, andxandyare in the interval [−r, r]. Assapproaches 0 in thelimit,the equation becomes a circle. When s = 1, the equation is a square. This shape can be visualized using online graphing calculators such asDesmos.

Similar shapes

A squircle (blue) compared with a rounded square (red).(Larger image)

A shape similar to a squircle, called arounded square,may be generated by separating four quarters of a circle and connecting their loose ends with straightlines,or by separating the four sides of a square and connecting them with quarter-circles. Such a shape is very similar but not identical to the squircle. Although constructing a rounded square may be conceptually and physically simpler, the squircle has a simpler equation and can be generalised much more easily. One consequence of this is that the squircle and other superellipses can be scaled up or down quite easily. This is useful where, for example, one wishes to create nested squircles.

Various forms of a truncated circle

Another similar shape is atruncatedcircle,the boundary of theintersectionof the regions enclosed by a square and by a concentric circle whosediameteris both greater than the length of the side of the square and less than the length of the diagonal of the square (so that each figure has interior points that are not in the interior of the other). Such shapes lack the tangent continuity possessed by both superellipses and rounded squares.

Arounded cubecan be defined in terms ofsuperellipsoids.

Uses

Squircles are useful inoptics.If light is passed through a two-dimensional square aperture, the central spot in thediffractionpattern can be closely modelled by a squircle or supercircle. If a rectangular aperture is used, the spot can be approximated by asuperellipse.

Squircles have also been used to constructdinner plates.A squircular plate has a larger area (and can thus hold more food) than a circular one with the same radius, but still occupies the same amount of space in a rectangular or square cupboard.

ManyNokiaphone models have been designed with a squircle-shaped touchpad button,as was the second generationMicrosoft Zune.Appleuses an approximation of a squircle (actually a quintic superellipse) for icons iniOS,iPadOS,macOS,and the home buttons of some Apple hardware.One of the shapes for adaptive icons introduced in theAndroid "Oreo"operating system is a squircle.Samsunguses squircle-shaped icons in their Android software overlayOne UI,and inSamsung ExperienceandTouchWiz.

Italian car manufacturerFiatused numerous squircles in the interior and exterior design of the third generationPanda.

See also

• Astroid
• Ellipse
• Ellipsoid
• L^pspaces
• Oval
• Squround
• Superegg

External links

• What is the area of a Squircle?onYouTubebyMatt Parker
• Online Calculator for supercircle and super-ellipse
• Web based supercircle generator