Cartesian coordinate system

Illustration of a Cartesian coordinate plane. Four points are marked and labeled with their coordinates:(2, 3)in green,(−3, 1)in red,(−1.5, −2.5)in blue, and the origin(0, 0)in purple.

Ingeometry,aCartesian coordinate system(UK:/kɑːrˈtiːzjən/,US:/kɑːrˈtiːʒən/) in aplaneis acoordinate systemthat specifies eachpointuniquely by a pair ofreal numberscalledcoordinates,which are thesigneddistances to the point from two fixedperpendicularoriented lines, calledcoordinate lines,coordinate axesor justaxes(plural ofaxis) of the system. The point where they meet is called theoriginand has $(0, 0)$ as coordinates.

Similarly, the position of any point inthree-dimensional spacecan be specified by threeCartesian coordinates,which are the signed distances from the point to three mutually perpendicular planes. More generally, $n$ Cartesian coordinates specify the point in an $n$ -dimensionalEuclidean spacefor anydimension $n$ .These coordinates are the signed distances from the point to $n$ mutually perpendicular fixedhyperplanes.

Cartesian coordinate system with a circle of radius 2 centered at the origin marked in red. The equation of a circle is(x−a)²+ (y−b)²=r²whereaandbare the coordinates of the center(a,b)andris the radius.

Cartesian coordinates are named forRené Descartes,whose invention of them in the 17th century revolutionized mathematics by allowing the expression of problems of geometry in terms ofalgebraandcalculus.Using the Cartesian coordinate system, geometric shapes (such ascurves) can be described byequationsinvolving the coordinates of points of the shape. For example, acircleof radius 2, centered at the origin of the plane, may be described as thesetof all points whose coordinates $x$ and $y$ satisfy the equation $x 2 + y 2 = 4$ ;thearea,theperimeterand thetangent lineat any point can be computed from this equation by usingintegralsandderivatives,in a way that can be applied to any curve.

Cartesian coordinates are the foundation ofanalytic geometry,and provide enlightening geometric interpretations for many other branches of mathematics, such aslinear algebra,complex analysis,differential geometry,multivariatecalculus,group theoryand more. A familiar example is the concept of thegraph of a function.Cartesian coordinates are also essential tools for most applied disciplines that deal with geometry, includingastronomy,physics,engineeringand many more. They are the most common coordinate system used incomputer graphics,computer-aided geometric designand othergeometry-related data processing.

History

The adjectiveCartesianrefers to the Frenchmathematicianandphilosopher René Descartes,who published this idea in 1637 while he was resident in theNetherlands.It was independently discovered byPierre de Fermat,who also worked in three dimensions, although Fermat did not publish the discovery.^[1]The French clericNicole Oresmeused constructions similar to Cartesian coordinates well before the time of Descartes and Fermat.^[2]

Both Descartes and Fermat used a single axis in their treatments and have a variable length measured in reference to this axis.^[3]The concept of using a pair of axes was introduced later, after Descartes'La Géométriewas translated into Latin in 1649 byFrans van Schootenand his students. These commentators introduced several concepts while trying to clarify the ideas contained in Descartes's work.^[4]

The development of the Cartesian coordinate system would play a fundamental role in the development of thecalculusbyIsaac NewtonandGottfried Wilhelm Leibniz.^[5]The two-coordinate description of the plane was later generalized into the concept ofvector spaces.^[6]

Many other coordinate systems have been developed since Descartes, such as thepolar coordinatesfor the plane, and thesphericalandcylindrical coordinatesfor three-dimensional space.

Description

One dimension

Anaffine linewith a chosen Cartesian coordinate system is called anumber line.Every point on the line has a real-number coordinate, and every real number represents some point on the line.

There are twodegrees of freedomin the choice of Cartesian coordinate system for a line, which can be specified by choosing two distinct points along the line and assigning them to two distinctreal numbers(most commonly zero and one). Other points can then be uniquely assigned to numbers bylinear interpolation.Equivalently, one point can be assigned to a specific real number, for instance anoriginpoint corresponding to zero, and anorientedlength along the line can be chosen as a unit, with the orientation indicating the correspondence between directions along the line and positive or negative numbers.^[7]Each point corresponds to its signed distance from the origin (a number with an absolute value equal to the distance and a $+$ or $-$ sign chosen based on direction).

Ageometric transformationof the line can be represented by afunction of a real variable,for exampletranslationof the line corresponds to addition, andscalingthe line corresponds to multiplication. Any two Cartesian coordinate systems on the line can be related to each-other by alinear function(function of the form $x\mapsto ax+b$ )taking a specific point's coordinate in one system to its coordinate in the other system. Choosing a coordinate system for each of two different lines establishes anaffine mapfrom one line to the other taking each point on one line to the point on the other line with the same coordinate.

Two dimensions

A Cartesian coordinate system in two dimensions (also called arectangular coordinate systemor anorthogonal coordinate system^[8]) is defined by anordered pairofperpendicularlines (axes), a singleunit of lengthfor both axes, and an orientation for each axis. The point where the axes meet is taken as the origin for both, thus turning each axis into a number line. For any pointP,a line is drawn throughPperpendicular to each axis, and the position where it meets the axis is interpreted as a number. The two numbers, in that chosen order, are theCartesian coordinatesofP.The reverse construction allows one to determine the pointPgiven its coordinates.

The first and second coordinates are called theabscissaand theordinateofP,respectively; and the point where the axes meet is called theoriginof the coordinate system. The coordinates are usually written as two numbers in parentheses, in that order, separated by a comma, as in(3, −10.5).Thus the origin has coordinates(0, 0),and the points on the positive half-axes, one unit away from the origin, have coordinates(1, 0)and(0, 1).

In mathematics, physics, and engineering, the first axis is usually defined or depicted as horizontal and oriented to the right, and the second axis is vertical and oriented upwards. (However, in somecomputer graphicscontexts, the ordinate axis may be oriented downwards.) The origin is often labeledO,and the two coordinates are often denoted by the lettersXandY,orxandy.The axes may then be referred to as theX-axis andY-axis. The choices of letters come from the original convention, which is to use the latter part of the Alpha bet to indicate unknown values. The first part of the Alpha bet was used to designate known values.

AEuclidean planewith a chosen Cartesian coordinate system is called aCartesian plane.In a Cartesian plane, one can define canonical representatives of certain geometric figures, such as theunit circle(with radius equal to the length unit, and center at the origin), theunit square(whose diagonal has endpoints at(0, 0)and(1, 1)), theunit hyperbola,and so on.

The two axes divide the plane into fourright angles,calledquadrants.The quadrants may be named or numbered in various ways, but the quadrant where all coordinates are positive is usually called thefirst quadrant.

If the coordinates of a point are(x,y),then itsdistancesfrom theX-axis and from theY-axis are |y| and |x|, respectively; where | · | denotes theabsolute valueof a number.

Three dimensions

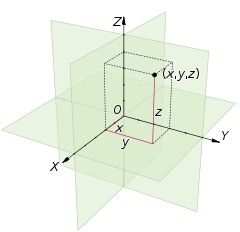
A Cartesian coordinate system for a three-dimensional space consists of an ordered triplet of lines (theaxes) that go through a common point (theorigin), and are pair-wise perpendicular; an orientation for each axis; and a single unit of length for all three axes. As in the two-dimensional case, each axis becomes a number line. For any pointPof space, one considers a plane throughPperpendicular to each coordinate axis, and interprets the point where that plane cuts the axis as a number. The Cartesian coordinates ofPare those three numbers, in the chosen order. The reverse construction determines the pointPgiven its three coordinates.

Alternatively, each coordinate of a pointPcan be taken as the distance fromPto the plane defined by the other two axes, with the sign determined by the orientation of the corresponding axis.

Each pair of axes defines acoordinate plane.These planes divide space into eightoctants.The octants are:

${\begin{aligned}(+x,+y,+z)&&(-x,+y,+z)&&(+x,-y,+z)&&(+x,+y,-z)\\(+x,-y,-z)&&(-x,+y,-z)&&(-x,-y,+z)&&(-x,-y,-z)\end{aligned}}$

The coordinates are usually written as three numbers (or algebraic formulas) surrounded by parentheses and separated by commas, as in $(3, -2.5, 1)$ or $(t, u + v, π /2)$ .Thus, the origin has coordinates $(0, 0, 0)$ ,and the unit points on the three axes are $(1, 0, 0)$ , $(0, 1, 0)$ ,and $(0, 0, 1)$ .

Standard names for the coordinates in the three axes areabscissa,ordinateandapplicate^[9].The coordinates are often denoted by the lettersx,y,andz.The axes may then be referred to as thex-axis,y-axis, andz-axis, respectively. Then the coordinate planes can be referred to as thexy-plane,yz-plane, andxz-plane.

In mathematics, physics, and engineering contexts, the first two axes are often defined or depicted as horizontal, with the third axis pointing up. In that case the third coordinate may be calledheightoraltitude.The orientation is usually chosen so that the 90-degree angle from the first axis to the second axis looks counter-clockwise when seen from the point $(0, 0, 1)$ ;a convention that is commonly calledtheright-hand rule.

Higher dimensions

Since Cartesian coordinates are unique and non-ambiguous, the points of a Cartesian plane can be identified with pairs ofreal numbers;that is, with theCartesian product $\mathbb {R} ^{2}=\mathbb {R} \times \mathbb {R}$ ,where $\mathbb {R}$ is the set of all real numbers. In the same way, the points in anyEuclidean spaceof dimensionnbe identified with thetuples(lists) ofnreal numbers; that is, with the Cartesian product $\mathbb {R} ^{n}$ .

Generalizations

The concept of Cartesian coordinates generalizes to allow axes that are not perpendicular to each other, and/or different units along each axis. In that case, each coordinate is obtained by projecting the point onto one axis along a direction that is parallel to the other axis (or, in general, to thehyperplanedefined by all the other axes). In such anoblique coordinate systemthe computations of distances and angles must be modified from that in standard Cartesian systems, and many standard formulas (such as the Pythagorean formula for the distance) do not hold (seeaffine plane).

Notations and conventions

The Cartesian coordinates of a point are usually written inparenthesesand separated by commas, as in(10, 5)or(3, 5, 7).The origin is often labelled with the capital letterO.In analytic geometry, unknown or generic coordinates are often denoted by the letters (x,y) in the plane, and (x,y,z) in three-dimensional space. This custom comes from a convention of algebra, which uses letters near the end of the Alpha bet for unknown values (such as the coordinates of points in many geometric problems), and letters near the beginning for given quantities.

These conventional names are often used in other domains, such as physics and engineering, although other letters may be used. For example, in a graph showing how apressurevaries withtime,the graph coordinates may be denotedpandt.Each axis is usually named after the coordinate which is measured along it; so one says thex-axis,they-axis,thet-axis,etc.

Another common convention for coordinate naming is to use subscripts, as (x₁,x₂,...,x_n) for thencoordinates in ann-dimensional space, especially whennis greater than 3 or unspecified. Some authors prefer the numbering (x₀,x₁,...,x_n−1). These notations are especially advantageous incomputer programming:by storing the coordinates of a point as anarray,instead of arecord,thesubscriptcan serve to index the coordinates.

In mathematical illustrations of two-dimensional Cartesian systems, the first coordinate (traditionally called theabscissa) is measured along ahorizontalaxis, oriented from left to right. The second coordinate (theordinate) is then measured along averticalaxis, usually oriented from bottom to top. Young children learning the Cartesian system, commonly learn the order to read the values before cementing thex-,y-, andz-axis concepts, by starting with 2D mnemonics (for example, 'Walk along the hall then up the stairs' akin to straight across thex-axis then up vertically along they-axis).

Computer graphics andimage processing,however, often use a coordinate system with they-axis oriented downwards on the computer display. This convention developed in the 1960s (or earlier) from the way that images were originally stored indisplay buffers.

For three-dimensional systems, a convention is to portray thexy-plane horizontally, with thez-axis added to represent height (positive up). Furthermore, there is a convention to orient thex-axis toward the viewer, biased either to the right or left. If a diagram (3D projectionor2D perspective drawing) shows thex- andy-axis horizontally and vertically, respectively, then thez-axis should be shown pointing "out of the page" towards the viewer or camera. In such a 2D diagram of a 3D coordinate system, thez-axis would appear as a line or ray pointing down and to the left or down and to the right, depending on the presumed viewer or cameraperspective.In any diagram or display, the orientation of the three axes, as a whole, is arbitrary. However, the orientation of the axes relative to each other should always comply with theright-hand rule,unless specifically stated otherwise. All laws of physics and math assume thisright-handedness,which ensures consistency.

For 3D diagrams, the names "abscissa" and "ordinate" are rarely used forxandy,respectively. When they are, thez-coordinate is sometimes called theapplicate.The wordsabscissa,ordinateandapplicateare sometimes used to refer to coordinate axes rather than the coordinate values.^[8]

Quadrants and octants

The axes of a two-dimensional Cartesian system divide the plane into four infinite regions, calledquadrants,^[8]each bounded by two half-axes. These are often numbered from 1st to 4th and denoted byRoman numerals:I (where the coordinates both have positive signs), II (where the abscissa is negative − and the ordinate is positive +), III (where both the abscissa and the ordinate are −), and IV (abscissa +, ordinate −). When the axes are drawn according to the mathematical custom, the numbering goescounter-clockwisestarting from the upper right ( "north-east" ) quadrant.

Similarly, a three-dimensional Cartesian system defines a division of space into eight regions oroctants,^[8]according to the signs of the coordinates of the points. The convention used for naming a specific octant is to list its signs; for example,(+ + +)or(− + −).The generalization of the quadrant and octant to an arbitrary number of dimensions is theorthant,and a similar naming system applies.

Cartesian formulae for the plane

Distance between two points

TheEuclidean distancebetween two points of the plane with Cartesian coordinates $(x_{1},y_{1})$ and $(x_{2},y_{2})$ is

$d={\sqrt {(x_{2}-x_{1})^{2}+(y_{2}-y_{1})^{2}}}.$

This is the Cartesian version ofPythagoras's theorem.In three-dimensional space, the distance between points $(x_{1},y_{1},z_{1})$ and $(x_{2},y_{2},z_{2})$ is

$d={\sqrt {(x_{2}-x_{1})^{2}+(y_{2}-y_{1})^{2}+(z_{2}-z_{1})^{2}}},$

which can be obtained by two consecutive applications of Pythagoras' theorem.^[10]

Euclidean transformations

TheEuclidean transformationsorEuclidean motionsare the (bijective) mappings of points of theEuclidean planeto themselves which preserve distances between points. There are four types of these mappings (also called isometries):translations,rotations,reflectionsandglide reflections.^[11]

Translation

Translatinga set of points of the plane, preserving the distances and directions between them, is equivalent to adding a fixed pair of numbers(a,b)to the Cartesian coordinates of every point in the set. That is, if the original coordinates of a point are(x,y),after the translation they will be

$(x',y')=(x+a,y+b).$

Rotation

Torotatea figurecounterclockwisearound the origin by some angle $\theta$ is equivalent to replacing every point with coordinates (x,y) by the point with coordinates (x',y'), where

${\begin{aligned}x'&=x\cos \theta -y\sin \theta \\y'&=x\sin \theta +y\cos \theta.\end{aligned}}$

Thus:

$(x',y')=((x\cos \theta -y\sin \theta \,),(x\sin \theta +y\cos \theta \,)).$

Reflection

If(x,y)are the Cartesian coordinates of a point, then(−x,y)are the coordinates of itsreflectionacross the second coordinate axis (the y-axis), as if that line were a mirror. Likewise,(x,−y)are the coordinates of its reflection across the first coordinate axis (the x-axis). In more generality, reflection across a line through the origin making an angle $\theta$ with the x-axis, is equivalent to replacing every point with coordinates(x,y)by the point with coordinates(x′,y′),where

${\begin{aligned}x'&=x\cos 2\theta +y\sin 2\theta \\y'&=x\sin 2\theta -y\cos 2\theta.\end{aligned}}$

Thus: $(x',y')=((x\cos 2\theta +y\sin 2\theta \,),(x\sin 2\theta -y\cos 2\theta \,)).$

Glide reflection

A glide reflection is the composition of a reflection across a line followed by a translation in the direction of that line. It can be seen that the order of these operations does not matter (the translation can come first, followed by the reflection).

General matrix form of the transformations

Allaffine transformationsof the plane can be described in a uniform way by using matrices. For this purpose, the coordinates $(x,y)$ of a point are commonly represented as thecolumn matrix ${\begin{pmatrix}x\\y\end{pmatrix}}.$ The result $(x',y')$ of applying an affine transformation to a point $(x,y)$ is given by the formula ${\begin{pmatrix}x'\\y'\end{pmatrix}}=A{\begin{pmatrix}x\\y\end{pmatrix}}+b,$ where $A={\begin{pmatrix}A_{1,1}&A_{1,2}\\A_{2,1}&A_{2,2}\end{pmatrix}}$ is a 2×2matrixand $b={\begin{pmatrix}b_{1}\\b_{2}\end{pmatrix}}$ is a column matrix.^[12]That is, ${\begin{aligned}x'&=xA_{1,1}+yA_{1,1}+b_{1}\\y'&=xA_{2,1}+yA_{2,2}+b_{2}.\end{aligned}}$

Among the affine transformations, theEuclidean transformationsare characterized by the fact that the matrix $A$ isorthogonal;that is, its columns areorthogonal vectorsofEuclidean normone, or, explicitly, $A_{1,1}A_{1,2}+A_{2,1}A_{2,2}=0$ and $A_{1,1}^{2}+A_{2,1}^{2}=A_{1,2}^{2}+A_{2,2}^{2}=1.$

This is equivalent to saying that $A$ times itstransposeis theidentity matrix.If these conditions do not hold, the formula describes a more generalaffine transformation.

The transformation is a translationif and only if $A$ is theidentity matrix.The transformation is a rotation around some point if and only if $A$ is arotation matrix,meaning that it is orthogonal and $A_{1,1}A_{2,2}-A_{2,1}A_{1,2}=1.$

A reflection or glide reflection is obtained when, $A_{1,1}A_{2,2}-A_{2,1}A_{1,2}=-1.$

Assuming that translations are not used (that is, $b_{1}=b_{2}=0$ ) transformations can becomposedby simply multiplying the associated transformation matrices. In the general case, it is useful to use theaugmented matrixof the transformation; that is, to rewrite the transformation formula ${\begin{pmatrix}x'\\y'\\1\end{pmatrix}}=A'{\begin{pmatrix}x\\y\\1\end{pmatrix}},$ where $A'={\begin{pmatrix}A_{1,1}&A_{1,2}&b_{1}\\A_{2,1}&A_{2,2}&b_{2}\\0&0&1\end{pmatrix}}.$ With this trick, the composition of affine transformations is obtained by multiplying the augmented matrices.

Affine transformation

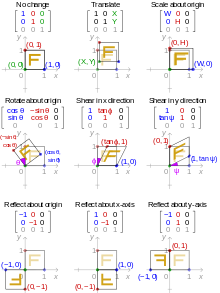
Affine transformationsof theEuclidean planeare transformations that map lines to lines, but may change distances and angles. As said in the preceding section, they can be represented with augmented matrices: ${\begin{pmatrix}A_{1,1}&A_{2,1}&b_{1}\\A_{1,2}&A_{2,2}&b_{2}\\0&0&1\end{pmatrix}}{\begin{pmatrix}x\\y\\1\end{pmatrix}}={\begin{pmatrix}x'\\y'\\1\end{pmatrix}}.$

The Euclidean transformations are the affine transformations such that the 2×2 matrix of the $A_{i,j}$ isorthogonal.

The augmented matrix that represents thecompositionof two affine transformations is obtained by multiplying their augmented matrices.

Some affine transformations that are not Euclidean transformations have received specific names.

Scaling

An example of an affine transformation which is not Euclidean is given by scaling. To make a figure larger or smaller is equivalent to multiplying the Cartesian coordinates of every point by the same positive numberm.If(x,y)are the coordinates of a point on the original figure, the corresponding point on the scaled figure has coordinates

$(x',y')=(mx,my).$

Ifmis greater than 1, the figure becomes larger; ifmis between 0 and 1, it becomes smaller.

Shearing

Ashearing transformationwill push the top of a square sideways to form a parallelogram. Horizontal shearing is defined by:

$(x',y')=(x+ys,y)$

Shearing can also be applied vertically:

$(x',y')=(x,xs+y)$

Orientation and handedness

In two dimensions

Fi xing or choosing thex-axis determines they-axis up to direction. Namely, they-axis is necessarily theperpendicularto thex-axis through the point marked 0 on thex-axis. But there is a choice of which of the two half lines on the perpendicular to designate as positive and which as negative. Each of these two choices determines a different orientation (also calledhandedness) of the Cartesian plane.

The usual way of orienting the plane, with the positivex-axis pointing right and the positivey-axis pointing up (and thex-axis being the "first" and they-axis the "second" axis), is considered thepositiveorstandardorientation, also called theright-handedorientation.

A commonly used mnemonic for defining the positive orientation is theright-hand rule.Placing a somewhat closed right hand on the plane with the thumb pointing up, the fingers point from thex-axis to they-axis, in a positively oriented coordinate system.

The other way of orienting the plane is following theleft-hand rule,placing the left hand on the plane with the thumb pointing up.

When pointing the thumb away from the origin along an axis towards positive, the curvature of the fingers indicates a positive rotation along that axis.

Regardless of the rule used to orient the plane, rotating the coordinate system will preserve the orientation. Switching any one axis will reverse the orientation, but switching both will leave the orientation unchanged.

In three dimensions

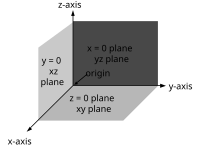
Once thex- andy-axes are specified, they determine thelinealong which thez-axis should lie, but there are two possible orientations for this line. The two possible coordinate systems, which result are called 'right-handed' and 'left-handed'.^[13]The standard orientation, where thexy-plane is horizontal and thez-axis points up (and thex- and they-axis form a positively oriented two-dimensional coordinate system in thexy-plane if observed fromabovethexy-plane) is calledright-handedorpositive.

The name derives from theright-hand rule.If theindex fingerof the right hand is pointed forward, themiddle fingerbent inward at a right angle to it, and thethumbplaced at a right angle to both, the three fingers indicate the relative orientation of thex-,y-, andz-axes in aright-handedsystem. The thumb indicates thex-axis, the index finger they-axis and the middle finger thez-axis. Conversely, if the same is done with the left hand, a left-handed system results.

Figure 7 depicts a left and a right-handed coordinate system. Because a three-dimensional object is represented on the two-dimensional screen, distortion and ambiguity result. The axis pointing downward (and to the right) is also meant to pointtowardsthe observer, whereas the "middle" -axis is meant to pointawayfrom the observer. The red circle isparallelto the horizontalxy-plane and indicates rotation from thex-axis to they-axis (in both cases). Hence the red arrow passesin front ofthez-axis.

Figure 8 is another attempt at depicting a right-handed coordinate system. Again, there is an ambiguity caused by projecting the three-dimensional coordinate system into the plane. Many observers see Figure 8 as "flipping in and out" between aconvexcube and aconcave"corner". This corresponds to the two possible orientations of the space. Seeing the figure as convex gives a left-handed coordinate system. Thus the "correct" way to view Figure 8 is to imagine thex-axis as pointingtowardsthe observer and thus seeing a concave corner.

Representing a vector in the standard basis

A point in space in a Cartesian coordinate system may also be represented by a positionvector,which can be thought of as an arrow pointing from the origin of the coordinate system to the point.^[14]If the coordinates represent spatial positions (displacements), it is common to represent the vector from the origin to the point of interest as $\mathbf {r}$ .In two dimensions, the vector from the origin to the point with Cartesian coordinates (x, y) can be written as:

$\mathbf {r} =x\mathbf {i} +y\mathbf {j},$

where $\mathbf {i} ={\begin{pmatrix}1\\0\end{pmatrix}}$ and $\mathbf {j} ={\begin{pmatrix}0\\1\end{pmatrix}}$ areunit vectorsin the direction of thex-axis andy-axis respectively, generally referred to as thestandard basis(in some application areas these may also be referred to asversors). Similarly, in three dimensions, the vector from the origin to the point with Cartesian coordinates $(x,y,z)$ can be written as:^[15] $\mathbf {r} =x\mathbf {i} +y\mathbf {j} +z\mathbf {k},$

where $\mathbf {i} ={\begin{pmatrix}1\\0\\0\end{pmatrix}},$ $\mathbf {j} ={\begin{pmatrix}0\\1\\0\end{pmatrix}},$ and $\mathbf {k} ={\begin{pmatrix}0\\0\\1\end{pmatrix}}.$

There is nonaturalinterpretation of multiplying vectors to obtain another vector that works in all dimensions, however there is a way to usecomplex numbersto provide such a multiplication. In a two-dimensional cartesian plane, identify the point with coordinates(x,y)with the complex numberz=x+iy.Here,iis theimaginary unitand is identified with the point with coordinates(0, 1),so it isnotthe unit vector in the direction of thex-axis. Since the complex numbers can be multiplied giving another complex number, this identification provides a means to "multiply" vectors. In a three-dimensional cartesian space a similar identification can be made with a subset of thequaternions.

Citations

^Bix, Robert A.; D'Souza, Harry J."Analytic geometry".Encyclopædia Britannica.Retrieved6 August2017.
^Kent & Vujakovic 2017,Seehere
^Katz, Victor J. (2009).A history of mathematics: an introduction(3rd ed.). Boston: Addison-Wesley. p. 484.ISBN 978-0-321-38700-4.OCLC 71006826.
^Burton 2011,p. 374.
^Berlinski 2011
^Axler 2015,p. 1
^Consider the tworaysor half-lines resulting from splitting the line at the origin. One of the half-lines can be assigned to positive numbers, and the other half-line to negative numbers.
^^a ^b ^c ^d"Cartesian orthogonal coordinate system".Encyclopedia of Mathematics.Retrieved6 August2017.
^"Cartesian coordinates".planetmath.org.Retrieved25 August2024.
^Hughes-Hallett, McCallum & Gleason 2013
^Smart 1998,Chap. 2
^Brannan, Esplen & Gray 1998,pg. 49
^Anton, Bivens & Davis 2021,p.657
^Brannan, Esplen & Gray 1998,Appendix 2, pp. 377–382
^Griffiths 1999

General and cited references

Axler, Sheldon (2015).Linear Algebra Done Right.Undergraduate Texts in Mathematics. Springer.doi:10.1007/978-3-319-11080-6.ISBN 978-3-319-11079-0.Archived fromthe originalon 27 May 2022.Retrieved17 April2022.
Berlinski, David (2011).A Tour of the Calculus.Knopf Doubleday Publishing Group.ISBN 9780307789730.
Brannan, David A.; Esplen, Matthew F.; Gray, Jeremy J. (1998).Geometry.Cambridge: Cambridge University Press.ISBN 978-0-521-59787-6.
Burton, David M. (2011).The History of Mathematics/An Introduction(7th ed.). New York: McGraw-Hill.ISBN 978-0-07-338315-6.
Griffiths, David J. (1999).Introduction to Electrodynamics.Prentice Hall.ISBN 978-0-13-805326-0.
Hughes-Hallett, Deborah; McCallum, William G.; Gleason, Andrew M. (2013).Calculus: Single and Multivariable(6th ed.).John Wiley & Sons.ISBN 978-0470-88861-2.
Kent, Alexander J.; Vujakovic, Peter (4 October 2017).The Routledge Handbook of Mapping and Cartography.Routledge.ISBN 9781317568216.
Smart, James R. (1998),Modern Geometries(5th ed.), Pacific Grove: Brooks/Cole,ISBN 978-0-534-35188-5
Anton, Howard; Bivens, Irl C.; Davis, Stephen (2021).Calculus: Multivariable.John Wiley & Sons.p. 657.ISBN 978-1-119-77798-4.

External links

Cartesian Coordinate System
Weisstein, Eric W."Cartesian Coordinates".MathWorld.
Coordinate Converter – converts between polar, Cartesian and spherical coordinates
Coordinates of a point– interactive tool to explore coordinates of a point
open source JavaScript class for 2D/3D Cartesian coordinate system manipulation

[1] Bix, Robert A.; D'Souza, Harry J."Analytic geometry".Encyclopædia Britannica.Retrieved6 August2017.

[2] Kent & Vujakovic 2017,Seehere

[3] Katz, Victor J. (2009).A history of mathematics: an introduction(3rd ed.). Boston: Addison-Wesley. p. 484.ISBN 978-0-321-38700-4.OCLC 71006826.

[4] Burton 2011,p. 374.

[5] Berlinski 2011

[6] Axler 2015,p. 1

[7] Consider the tworaysor half-lines resulting from splitting the line at the origin. One of the half-lines can be assigned to positive numbers, and the other half-line to negative numbers.

[:0-8] "Cartesian orthogonal coordinate system".Encyclopedia of Mathematics.Retrieved6 August2017.

[9] "Cartesian coordinates".planetmath.org.Retrieved25 August2024.

[10] Hughes-Hallett, McCallum & Gleason 2013

[11] Smart 1998,Chap. 2

[12] Brannan, Esplen & Gray 1998,pg. 49

[13] Anton, Bivens & Davis 2021,p.657

[14] Brannan, Esplen & Gray 1998,Appendix 2, pp. 377–382

[15] Griffiths 1999

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]

[15]

v t e Orthogonal coordinate systems
Two dimensional	Cartesian Polar(Log-polar) Parabolic Bipolar Elliptic
Three dimensional	Cartesian Cylindrical Spherical Parabolic Paraboloidal Oblate spheroidal Prolate spheroidal Ellipsoidal Elliptic cylindrical Toroidal Bispherical Bipolar cylindrical Conical 6-sphere